\(y + 2 x = 1\) and \(-2x + 3 = y\)
\(\therefore\)Parallel lines.
|
Previous
4.3 Inclination of a line
|
Next
4.5 Perpendicular lines
|
Another method of determining the equation of a straight line is to be given a point on the unknown line, \(\left({x}_{1};{y}_{1}\right)\), and the equation of a line which is parallel to the unknown line.
Let the equation of the unknown line be \(y = m_1x + c_1\) and the equation of the given line be \(y = m_2x + c_2\).
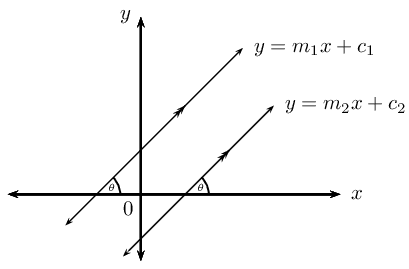
If the two lines are parallel then
\[m_1 = m_2\]
Important: when determining the gradient of a line using the coefficient of \(x\), make sure the given equation is written in the gradient–intercept (standard) form.
\[y=mx+c\]Substitute the value of \(m_2\) and the given point \(\left({x}_{1};{y}_{1}\right)\), into the gradient–intercept form of a straight line equation
\[y - y_1 = m(x - x_1)\]
and determine the equation of the unknown line.
Determine the equation of the line that passes through the point \((-1;1)\) and is parallel to the line \(y - 2x + 1 = 0\).
We write the given equation in gradient–intercept form and determine the value of \(m\).
\[y = 2x - 1\]
We know that the two lines are parallel, therefore \(m_1 = m_2 = 2\).
\[y - y_1 = m(x - x_1)\]
Substitute \(m = 2\)
\[y - y_1 = 2(x - x_1)\]
Substitute the given point \((-1;1)\)
\begin{align*} y - 1 & = 2(x - (-1)) \\ y - 1& = 2x + 2\\ y & = 2x + 2 + 1\\ &= 2x + 3 \end{align*}
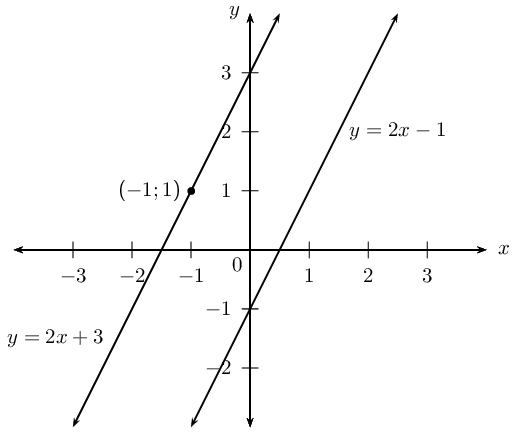
A sketch was not required, but it is always helpful and can be used to check answers.
The equation of the straight line is \(y = 2x + 3\).
Line \(AB\) passes through the point \(A(0;3)\) and has an angle of inclination of \(\text{153,4}\)\(\text{°}\). Determine the equation of the line \(CD\) which passes through the point \(C(2;-3)\) and is parallel to \(AB\).
Since we are given \(AB \parallel CD\),
\[m_{CD} = m_{AB} = -\text{0,5}\]
\[y - y_1 = m(x - x_1)\]
Substitute the gradient \(m_{CD} = -\text{0,5}\).
\[y - y_1 = -\frac{1}{2}(x - x_1)\]
Substitute the given point \((2;-3)\).
\begin{align*} y - (-3) & = -\frac{1}{2}(x - 2) \\ y + 3 & = -\frac{1}{2}x + 1\\ y & = -\frac{1}{2}x - 2 \end{align*}

A sketch was not required, but it is always useful.
The equation of the straight line is \(y = -\frac{1}{2}x - 2\).
Determine whether or not the following two lines are parallel:
\(y + 2 x = 1\) and \(-2x + 3 = y\)
\(\therefore\)Parallel lines.
\(\frac{y}{3} + x + 5 = 0\) and \(2y + 6x = 1\)
\(\therefore\)Parallel lines.
\(y = 2x - 7\) and the line passing through \((1;-2)\) and \((\frac{1}{2};-1)\)
\(\therefore\)Parallel lines.
\(y + 1 = x\) and \(x + y = 3\)
\(\therefore\)Not parallel lines.
The line passing through points \((-2;-1)\) and \((-4;-3)\) and the line \(-y + x - 4 = 0\)
\(\therefore\)Parallel lines.
\(y - 1 = \frac{1}{3}x\) and the line passing through points \((-2;4)\) and \((1;5)\)
\(\therefore\)Parallel lines.
Determine the equation of the straight line that passes through the point \((1;-5)\) and is parallel to the line \(y + 2x - 1 = 0\).
Determine the equation of the straight line that passes through the point \((-2;-6)\) and is parallel to the line \(2y + 1 = 6x\).
Determine the equation of the straight line that passes through the point \((-2;-2)\) and is parallel to the line with angle of inclination \(\theta = \text{56,31}\text{°}\).
Determine the equation of the straight line that passes through the point \((-2;\frac{2}{5})\) and is parallel to the line with angle of inclination \(\theta = \text{145}\text{°}\).
|
Previous
4.3 Inclination of a line
|
Table of Contents |
Next
4.5 Perpendicular lines
|