\(\text{Zn (s)} + \text{Ag}^{+}\text{(aq)} \rightarrow \text{Zn}^{2+}\text{(aq)}
+ \text{Ag (s)}\)
Write a reaction for each compound:
\[\text{Zn} \rightarrow \text{Zn}^{2+}\]
\[\text{Ag}^{+} \rightarrow \text{Ag}\]
The atoms are balanced.
Add electrons to each reaction so that the charges balance. We add the electrons
to the side with the greater positive charge.
\[\text{Zn} \rightarrow \text{Zn}^{2+} + 2e^{-}\]
\[\text{Ag}^{+} + e^{-} \rightarrow \text{Ag}\]
We now make sure that the number of electrons in both reactions is the same.
The reaction for zinc has two electrons, while the reaction for silver has one
electron. So we must multiply the reaction for silver by \(\text{2}\) to ensure
that the charges balance.
\[\text{Zn} \rightarrow \text{Zn}^{2+} + 2e^{-}\]
\[2\text{Ag}^{+} + 2e^{-} \rightarrow 2\text{Ag}\]
We combine the two half-reactions:
|
\(\text{Zn}\) |
\(\rightarrow\) |
\(\text{Zn}^{2+} + 2e^{-}\) |
| \(+\) |
\(2\text{Ag}^{+} + 2e^{-}\) |
\(\rightarrow\) |
\(2\text{Ag}\) |
|
\(\text{Zn} + \text{Ag}^{+} + 2e^{-}\) |
\(\rightarrow\) |
\(\text{Zn}^{2+} + 2\text{Ag} + 2e^{-}\) |
Cancelling out the electrons gives:
\[\text{Zn (s)} + 2\text{Ag}^{+}\text{(aq)} \rightarrow \text{Zn}^{2+}\text{(aq)} +
2\text{Ag (s)}\]
\(\text{Cu}^{2+}\text{(aq)} + \text{Cl}^{-}\text{(aq)} \rightarrow \text{Cu (s)}
+ \text{Cl}_{2}\text{(g)}\)
Write a reaction for each compound:
\[\text{Cu}^{2+} \rightarrow \text{Cu}\]
\[\text{Cl}^{-} \rightarrow \text{Cl}_{2}\]
Balance the atoms:
\[\text{Cu}^{2+} \rightarrow \text{Cu}\]
\[2\text{Cl}^{-} \rightarrow \text{Cl}_{2}\]
Add electrons to each reaction so that the charges balance. We add the electrons
to the side with the greater positive charge.
\[\text{Cu}^{2+} + 2e^{-} \rightarrow \text{Cu}\]
\[2\text{Cl}^{-} \rightarrow \text{Cl}_{2} + 2e^{-}\]
We now make sure that the number of electrons in both reactions is the same.
The reaction for copper has two electrons and the reaction for chlorine also has
two electrons. So the charges balance.
We combine the two half-reactions:
|
\(\text{Cu}^{2+} + 2e^{-}\) |
\(\rightarrow\) |
\(\text{Cu}\) |
| \(+\) |
\(2\text{Cl}^{-}\) |
\(\rightarrow\) |
\(\text{Cl}_{2} + 2e^{-}\) |
|
\(\text{Cu}^{2+} + 2\text{Cl}^{-} + 2e^{-}\) |
\(\rightarrow\) |
\(\text{Cu} + \text{Cl}_{2} + 2e^{-}\) |
Cancelling out the electrons gives:
\[\text{Cu}^{2+}\text{(aq)} + 2\text{Cl}^{-}\text{(aq)} \rightarrow \text{Cu
(s)} + \text{Cl}_{2}\text{(g)}\]
\(\text{Pb}^{2+}\text{(aq)} + \text{Br}^{-}\text{(aq)} \rightarrow \text{Pb (s)}
+ \text{Br}_{2}\text{(aq)}\)
Write a reaction for each compound:
\[\text{Pb}^{2+} \rightarrow \text{Pb}\]
\[\text{Br}^{-} \rightarrow \text{Br}_{2}\]
Balance the atoms:
\[\text{Pb}^{2+} \rightarrow \text{Pb}\]
\[2\text{Br}^{-} \rightarrow \text{Br}_{2}\]
Add electrons to each reaction so that the charges balance. We add the electrons
to the side with the greater positive charge.
\[\text{Pb}^{2+} + 2e^{-} \rightarrow \text{Pb}\]
\[2\text{Br}^{-} \rightarrow \text{Br}_{2} + 2e^{-}\]
We now make sure that the number of electrons in both reactions is the same.
The reaction for lead has two electrons and the reaction for bromine also has two
electrons. So the charges balance.
We combine the two half-reactions:
|
\(\text{Pb}^{2+} + 2e^{-}\) |
\(\rightarrow\) |
\(\text{Pb}\) |
| \(+\) |
\(2\text{Br}^{-}\) |
\(\rightarrow\) |
\(\text{Br}_{2} + 2e^{-}\) |
|
\(\text{Pb}^{2+} + 2\text{Br}^{-} + 2e^{-}\) |
\(\rightarrow\) |
\(\text{Pb} + \text{Br}_{2} + 2e^{-}\) |
Cancelling out the electrons gives:
\[\text{Pb}^{2+}\text{(aq)} + 2\text{Br}^{-}\text{(aq)} \rightarrow \text{Pb (s)} +
\text{Br}_{2}\text{(g)}\]
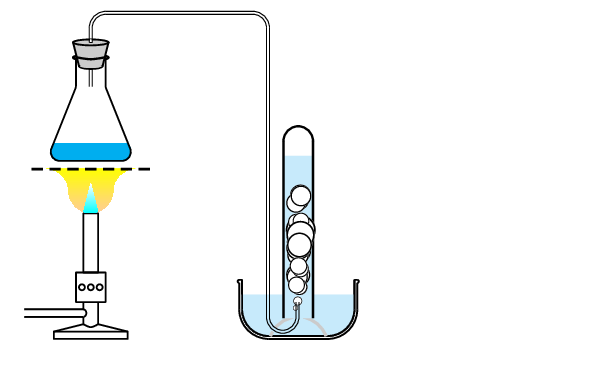
\(\text{HCl (aq)} + \text{MnO}_{2}\text{(s)} \rightarrow \text{Cl}_{2}\text{(g)}
+ \text{Mn}^{2+}\text{(aq)}\)
This reaction takes place in an acidic medium.
Write a reaction for each compound:
\[\text{HCl} \rightarrow \text{Cl}_{2}\]
\[\text{MnO}_{2} \rightarrow \text{Mn}^{2+}\]
Balance the atoms.
In the first reaction we have \(\text{1}\) chlorine atom on the left hand side
and \(\text{2}\) chlorine atoms on the right hand side. So we multiply the left
hand side by 2:
\[2\text{HCl} \rightarrow \text{Cl}_{2}\]
Now we note that there are \(\text{2}\) hydrogen atoms on the left hand side and
no hydrogen atoms on the right hand so we add \(\text{2}\) hydrogen ions to the
right hand side:
\[2\text{HCl} \rightarrow \text{Cl}_{2} + 2\text{H}^{+}\]
The equation is now balanced.
For the second equation we need to add two water molecules to the right hand
side:
\[\text{MnO}_{2} \rightarrow \text{Mn}^{2+} + 2\text{H}_{2}\text{O}\]
We now need to add four hydrogen ions to the left hand side to balance the
hydrogens:
\[\text{MnO}_{2} + 4\text{H}^{+} \rightarrow \text{Mn}^{2+} +
2\text{H}_{2}\text{O}\]
This equation is now balanced.
Add electrons to each reaction so that the charges balance. We add the electrons
to the side with the greater positive charge.
\[2\text{HCl} \rightarrow \text{Cl}_{2} + 2\text{H}^{+} + 2e^{-}\]
\[\text{MnO}_{2} + 4\text{H}^{+} + 2e^{-} \rightarrow \text{Mn}^{2+} +
2\text{H}_{2}\text{O}\]
We now make sure that the number of electrons in both reactions is the same.
The reaction for chlorine has two electrons and the reaction for manganese also
has two electrons. So the charges balance.
We combine the two half-reactions:
|
\(2\text{HCl}\) |
\(\rightarrow\) |
\(\text{Cl}_{2} + 2\text{H}^{+} + 2e^{-}\) |
| \(+\) |
\(\text{MnO}_{2} + 4\text{H}^{+} + 2e^{-}\) |
\(\rightarrow\) |
\(\text{Mn}^{2+} + 2\text{H}_{2}\text{O}\) |
|
\(2\text{HCl} + \text{MnO}_{2} + 4\text{H}^{+} + 2e^{-}\) |
\(\rightarrow\) |
\(\text{Cl}_{2} + 2\text{H}^{+} + 2e^{-} + \text{Mn}^{2+} +
2\text{H}_{2}\text{O}\) |
Cancelling out the electrons and hydrogen ions gives:
\[2\text{HCl} + \text{MnO}_{2} + 2\text{H}^{+} \rightarrow \text{Cl}_{2} +
\text{Mn}^{2+} + 2\text{H}_{2}\text{O}\]