12.2 Angles on a straight line
Let us look at angles formed on one side of a straight line.
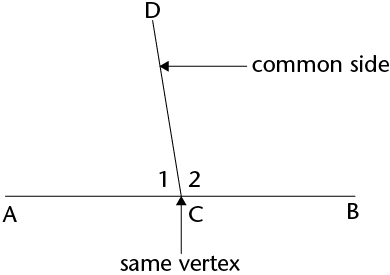
In this diagram, line segment \(AB\) meets line segment \(DC\). The angle at the vertex, \(C\), where they
meet, is now split into two angles: \(\hat{C_1}\) and \(\hat{C_2}\).
\(\hat{C_1}\) is the name for the angle at vertex \(C\) labelled "1" (or \(A\hat{C}D\)).
The sum of the angles that are formed on a straight line is always \(180^{\circ}\).
We can shorten this property as: \(\angle\)s on a straight line.
- Two angles that add up to \(180^{\circ}\) are called supplementary angles.
- Angles that share a vertex and a common side are said to be adjacent angles. \(\hat{C_1}\)
and \(\hat{C_2}\) are supplementary angles.
- Hence, \(\hat{C_1}\) and \(\hat{C_2}\) are called adjacent supplementary angles.
- supplementary angles
- two angles that add up to \(180^{\circ}\)
- adjacent angles
- angles that share a vertex and a common side
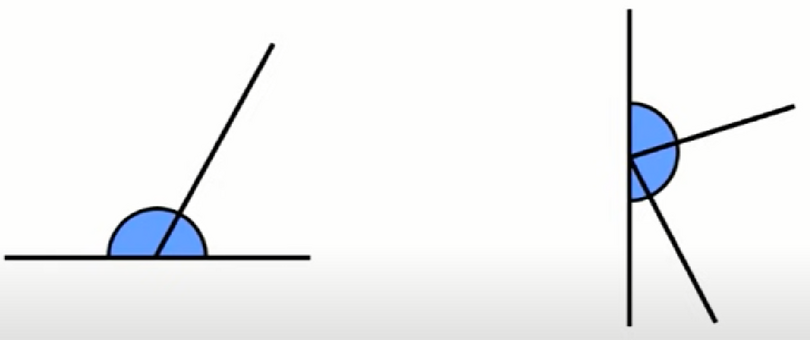
You can have more than one line meeting at the same point on a straight line. Here are a few examples of angles
on a straight line.
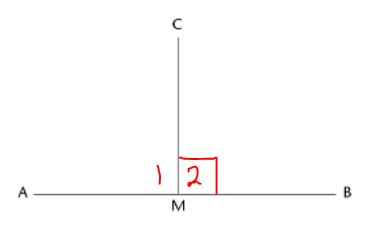
The sum of the angles on perpendicular lines
When two lines are perpendicular, the adjacent supplementary angles are both equal to \(90^{\circ}\).
In the diagram, \(\hat{M_1}=\hat{M_2} =90^{\circ}\).
A right angle is shown by forming a square at one of the right angles, like this: ⦜.
Finding unknown angles on straight lines
Worked example 12.1: Calculating
unknown angles on a straight line
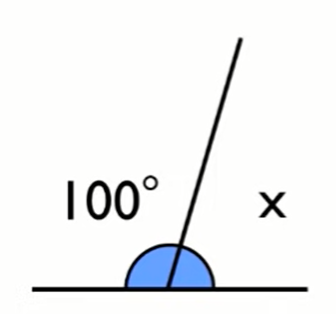
Calculate the size of \(x\).
In the diagram, we have two angles that are on the same side of the straight line. The first angle is
\(100^{\circ}\) and the second angle is unknown (\(x\)). We need to calculate the size of \(x\).
We know that the two angles have
a sum of \(180^{\circ}\), so we can say that:
\(100^{\circ}+x=180^{\circ}\) (\(\angle\)s on a straight line)
Now we can solve this equation.
\[\begin{align}
x&=180^{\circ}-100^{\circ} \\
x&=80^{\circ}
\end{align}\]
Worked example 12.2: Calculating
unknown angles on a straight line
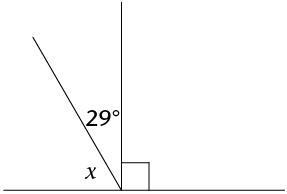
Calculate the size of \(x\).
Notice that there are three angles on the same side of the straight line. We have \(x\), an angle of
\(29^{\circ}\) and an angle of \(90^{\circ}\). (Remember that the ⦜ symbol on the diagram indicates a
\(90^{\circ}\) angle.) These three angles have a sum of \(180^{\circ}\), so we can say that:
\(x+29^{\circ}+90^{\circ}=180^{\circ}\) (\(\angle\)s on a straight line)
Now we can solve this equation.
\[\begin{align}
x+29^{\circ}+90^{\circ}&=180^{\circ} \\
x+119^{\circ}&=180^{\circ} \\
x&=180^{\circ}-119^{\circ} \\
x&=61^{\circ}
\end{align}\]
There is a simpler way to solve for \(x\). It is given that we have a perpendicular line. Adjacent angles on
a perpendicular line are both equal to \(90^{\circ}\). So, we have a different equation to solve.
\[\begin{align}
x+29^{\circ}&=90^{\circ} \\
x&=90^{\circ}-29^{\circ} \\
x&=61^{\circ}
\end{align}\]
Worked example 12.3: Calculating
unknown angles on a straight line
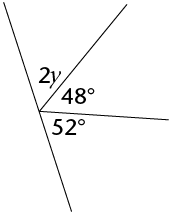
Calculate the size of \(y\).
Notice that we have three angles on the same side of the straight line. We have \(2y\), an angle of
\(48^{\circ}\) and an angle of \(52^{\circ}\). These three angles have a sum of \(180^{\circ}\), so we can
say that:
\(2y+48^{\circ}+52^{\circ}=180^{\circ}\) (\(\angle\)s on a straight line)
Now we can solve this equation.
\[\begin{align}
2y+48^{\circ}+52^{\circ}&=180^{\circ} \\
2y+100^{\circ}&=180^{\circ} \\
2y&=180^{\circ}-100^{\circ} \\
2y&=80^{\circ} \\
y&=40^{\circ}
\end{align}\]
Exercise 12.1
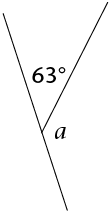
Calculate the size of \(a\).
\[\begin{align}
a+63^{\circ}&=180^{\circ} &(\angle\text{s on a straight line}) \\
x&=180^{\circ}-63^{\circ} \\
x&=117^{\circ}
\end{align}\]
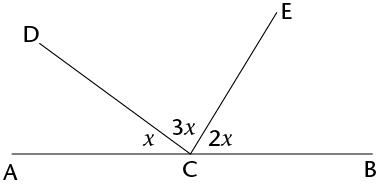
Calculate the size of:
- \(x\)
- \(\hat{ECB}\)
-
\[\begin{align}
x+3x+2x&=180^{\circ} &(\angle\text{s on a straight line}) \\
6x&=180^{\circ} \\
x&=30^{\circ}
\end{align}\]
-
\[\begin{align}
\hat{ECB}&=2x \\
&=2(30^{\circ})\\
&=60^{\circ}
\end{align}\]
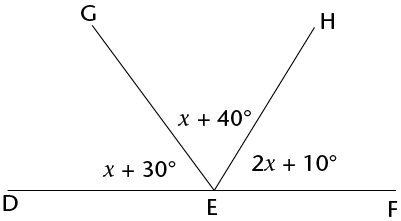
Calculate the size of:
- \(x\)
- \(\hat{GEH}\)
-
\[\begin{align}
(x+30^{\circ})+(x+40^{\circ}) +(2x+10^{\circ}) &=180^{\circ} &(\angle\text{s on a straight line})
\\
4x+80^{\circ}&=180^{\circ} \\
4x&=100^{\circ} \\
x&=25^{\circ}
\end{align}\]
-
\[\begin{align}
\hat{GEH}&=x+40^{\circ} \\
&=25^{\circ}+40^{\circ} \\
&=65^{\circ}
\end{align}\]
Hint: Remember that the matching curved lines “))” indicate that the angles are
equal.
Calculate the size of:
- \(k\)
- \(\hat{TYP}\)
-
\[\begin{align}
(2k)+(k+65^{\circ}) +(2k)
&=180^{\circ}
&&(\angle\text{s on a straight line}) \\
5k+65^{\circ}&=180^{\circ} \\
5k &=115^{\circ} \\
&=23^{\circ}
\end{align}\]
-
\[\begin{align}
\hat{TYP}&=k+65^{\circ} \\
&=23^{\circ}+65^{\circ} \\
&=88^{\circ}
\end{align}\]