Chapter 12: Geometry of straight lines
12.1 Introduction
In this chapter, we will explore the relationships between pairs of angles that are created when:
- straight lines intersect (meet or cross)
- lines are perpendicular to each other
- a third line crosses two parallel lines

You will come to understand what is meant by vertically opposite angles, corresponding angles, alternate angles and co-interior angles. You will then use your knowledge to help you work out unknown angles in geometric figures.
Notation
An angle is formed when two straight lines meet at a point. The point where the lines meet is called the vertex. Angles are labelled with a caret or 'hat' on a letter, for example, \(\hat{A}\).
- angle
- the space between two straight lines that meet at a point
- vertex
- the point at which two straight lines meet
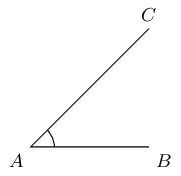
In this diagram, angle \(\hat{A}\) is formed between line segments \(BA\) and \(CA\). We can also name the angle according to the line segments that make up the angle, for example \(C\hat{A}B\) or \(B\hat{A}C\).
The \(\angle\) symbol is a short method of writing the word angle in geometry, and is often used in phrases such as "the sum of \(\angle\)s in \(\triangle\)".
