\(\log_{8}{(10 \times 10)}\)
2.8 Enrichment: more on logarithms
|
Previous
2.7 Summary
|
Next
3.1 Calculating the period of an investment
|
2.8 Enrichment: more on logarithms (EMCFR)
NOTE: THIS SECTION IS NOT PART OF THE CURRICULUM
Laws of logarithms (EMCFS)
Logarithmic law:
\[\log_{a}{xy} = \log_{a}{x} + \log_{a}{y} \qquad (x > 0 \text{ and } y > 0)\]Now we change from the exponential form back to logarithmic form:
\begin{align*} \log_{a}{xy} &= m + n \\ \text{But } m = {\log}_{a}\left(x\right) & \text{ and } n = {\log}_{a}\left(y\right) \\ \therefore \log_{a}{xy} &= {\log}_{a}\left(x\right) +{\log}_{a}\left(y\right) \end{align*}In words: the logarithm of a product is equal to the sum of the logarithms of the factors.
Worked example 22: Applying the logarithmic law \(\log_{a}{xy} = \log_{a}{x} + \log_{a}{y}\)
Simplify: \(\log{5} + \log{2} - \log{30}\)
Use the logarithmic law to simplify the expression
We combine the first two terms since the product of \(\text{5}\) and \(\text{2}\) is equal to \(\text{10}\), which is always useful when simplifying logarithms.
\begin{align*} \log{5} + \log{2} - \log{30} &= \left( \log{5} + \log{2} \right) - \log{30} \\ &= \log{\left( 5 \times 2\right)} - \log{30} \\ &= \log{10} - \log{30} \\ &= 1 - \log{30} \end{align*}We expand the last term to simplify the expression further:
\begin{align*} &= 1 - \log{( 3 \times 10)} \\ &= 1 - \left( \log{3} + \log{10} \right) \\ &= 1 - \left( \log{3} + 1 \right) \\ &= 1 - \log{3} - 1 \\ &= - \log{3} \end{align*}Write the final answer
\(\log{5} + \log{2} - \log{30} = - \log{3}\)Applying logarithmic law: \(\log_{a}{xy} = {\log}_{a}\left(x\right) +{\log}_{a}\left(y\right)\)
Simplify the following, if possible:
\(\log_{2}{14}\)
\(\log_{2}{(8 \times 5)}\)
\(\log_{16}{(x + y)}\)
\(\log_{16}{(x + y)}\) cannot be written as separate logarithms.
\(\log_{2}{2xy}\)
\(\log{(5 + 2)}\)
Note: \(\log{(5 + 2)} \ne \log{5} + \log{2}\). Do not confuse this with applying the distributive law: \(a(b + c) = ab + ac\).
Write the following as a single term, if possible:
\(\log{15} + \log{2}\)
\(\log{1} + \log{5} +\log{\frac{1}{5}}\)
\(1 + \log_{3}{4}\)
\(\left( \log{x} \right) \left( \log{y} \right) + \log{x}\)
\(\log{7} \times \log{2}\)
\(\log_{2}{7} + \log_{3}{2}\)
This cannot be written as one term because the bases are not the same.
\(\log_{a}{p} + \log_{a}{q}\)
\(\log_{a}{p} \times \log_{a}{q}\)
This is already a single term: \((\log_{a}{p})(\log_{a}{q})\)
Simplify the following:
\(\log{x} + \log{y} + \log{z}\)
\(\log{ab} + \log{bc} + \log{cd}\)
\(\log{125} + \log{2} + \log{8}\)
\(\log_{4}{\frac{3}{8}} + \log_{4}{\frac{10}{3}} + \log_{4}{\frac{16}{5}}\)
Logarithmic law:
\[\log_{a}{\frac{x}{y}} = \log_{a}{x} - \log_{a}{y} \qquad (x > 0 \text{ and } y > 0)\]Now we change from the exponential form back to logarithmic form:
\begin{align*} \log_{a}{\frac{x}{y}} &= m - n \\ \text{But } m = {\log}_{a}\left(x\right) & \text{ and } n = {\log}_{a}\left(y\right) \\ \therefore \log_{a}{\frac{x}{y}} &= {\log}_{a}\left(x\right) - {\log}_{a}\left(y\right) \end{align*}In words: the logarithm of a quotient is equal to the difference of the logarithms of the numerator and the denominator.
Worked example 23: Applying the logarithmic law \(\log_{a}{\frac{x}{y}} = \log_{a}{x} - \log_{a}{y}\)
Simplify: \(\log{40} - \log{4} + \log_{5}{\frac{8}{5}}\)
Use the logarithmic law to simplify the expression
We combine the first two terms since both terms have the same base and the quotient of \(\text{40}\) and \(\text{4}\) is equal to \(\text{10}\):
\begin{align*} \log{40} - \log{4} + \log_{5}{\frac{8}{5}} &= \left(\log{40} - \log{4} \right) + \log_{5}{\frac{8}{5}} \\ &= \left(\log{\frac{40}{4}} \right) + \log_{5}{\frac{8}{5}} \\ &= \log{10} + \log_{5}{\frac{8}{5}} \\ &= 1 + \log_{5}{\frac{8}{5}} \end{align*}We expand the last term to simplify the expression further:
\begin{align*} &= 1 + \left( \log_{5}{8} - \log_{5}{5} \right) \\ &= 1 + \log_{5}{8} - 1 \\ &= \log_{5}{8} \end{align*}Write the final answer
\(\log{40} - \log{4} + \log_{5}{\frac{8}{5}} = \log_{5}{8}\)Applying logarithmic law: \(\log_{a}{\frac{x}{y}} = {\log}_{a}x - {\log}_{a}y\)
Expand and simplify the following:
This cannot be simplified.
Write the following as a single term:
This cannot be simplified.
This cannot be simplified because the bases are not the same.
Simplify the following:
Vini and Dirk complete their mathematics homework and check each other's answers. Compare the two methods shown below and decide if they are correct or incorrect:
Question:
Simplify the following:
\[\log{m} - \log{n} - \log{p} - \log{q}\]Vini's answer:
\begin{align*} \log{m} - \log{n} - \log{p} - \log{q} &= \left( \log{m} - \log{n} \right) - \log{p} - \log{q} \\ &= \left( \log{\frac{m}{n}} - \log{p} \right) - \log{q} \\ &= \log{\left( \frac{m}{n} \times \frac{1}{p} \right)} - \log{q} \\ &= \log{\frac{m}{np} } - \log{q} \\ &= \log{\frac{m}{np} \times \frac{1}{q}} \\ &= \log{\frac{m}{npq} } \end{align*}Dirk's answer:
\begin{align*} \log{m} - \log{n} - \log{p} - \log{q} &= \log{m} - \left( \log{n} + \log{p} + \log{q} \right) \\ &= \log{m} - \log{(n \times p \times q)} \\ &= \log{m} - \log{(npq)} \\ &= \log{\frac{m}{npq} } \end{align*}Useful summary:
-
\(\log1=0\)
-
\(\log10=1\)
-
\(\log100=2\)
-
\(\log1000=3\)
-
\(\log{\frac{1}{10}}=-1\)
-
\(\log{\text{0,1}}=-1\)
-
\(\log{\text{0,01}}=-2\)
-
\(\log{\text{0,001}}=-3\)
Simplification of logarithms (EMCFT)
Worked example 24: Simplification of logarithms
Simplify (without a calculator): \(3\log3+\log125\)
Apply the appropriate logarithmic laws to simplify the expression
\begin{align*} 3\log3+\log125 &= 3\log3+\log{5^{3}} \\ &= 3\log3 + 3\log{5} \\ &= 3 \left( \log3 + \log{5} \right) \\ &= 3 \log{(3 \times 5)} \\ &= 3 \log{15} \end{align*}Write the final answer
We cannot simplify any further, therefore \(3\log3+\log125 = 3 \log{15}\).
Important: all the algebraic manipulation techniques \((\times, \div, +, -\), factorisation etc.) also apply for logarithmic expressions. Always be aware of the number of terms in an expression as this will help to determine how to simplify.
Simplification of logarithms
Simplify the following without using a calculator:
Solving logarithmic equations (EMCFV)
Worked example 25: Solving logarithmic equations
Solve for \(p\):
\[18 \log{p} - 36 = 0\]Make \(\log p\) the subject of the equation
\begin{align*} 18 \log{p} - 36 &= 0 \\ 18 \log{p} &= 36 \\ \frac{18 \log{p}}{18} &= \frac{36}{18} \\ \therefore \log{p} &= 2 \end{align*}Change from logarithmic form to exponential form
\begin{align*} \log{p} &= 2 \\ \therefore p &= 10^{2} \\ &= 100 \end{align*}Write the final answer
\(p = 100\)
Worked example 26: Solving logarithmic equations
Solve for \(n\) (correct to the nearest integer):
\[(\text{1,02})^{n} = 2\]Change from exponential form to logarithmic form
\begin{align*} (\text{1,02})^{n} &= 2 \\ \therefore n &= \log_{\text{1,02}}{2} \end{align*}Use a change of base to solve for \(n\)
\begin{align*} n &= \frac{\log{2}}{\log{\text{1,02}}} \\ \therefore n &= \text{35,00} \ldots \end{align*}Write the final answer
\(n = 35\)
Solving logarithmic equations
Determine the value of \(a\) (correct to \(\text{2}\) decimal places):
Alternative (longer) method:
\begin{align*} \log_{3}{a} - \log{\text{1,2}} &= 0 \\ \log_{3}{a} &= \log{\text{1,2}} \\ \frac{\log{a}}{\log{3}} &= \log{\text{1,2}} \\ \log{a} &= \log{3} \times \log{\text{1,2}} \\ \log{a} &= \text{0,037} \ldots \\ \therefore a &= \text{1,09} \end{align*}Alternative (longer) method:
\begin{align*} \log_{2}{(a - 1)} &= \text{1,5} \\ \frac{\log{(a - 1)}}{\log{2}} &= \text{1,5} \\ \log{(a - 1)} &= \log{2} \times \text{1,5} \\ \therefore a - 1 &= \text{2,83} \ldots \\ \therefore a &= \text{3,83} \end{align*}Alternative (longer) method:
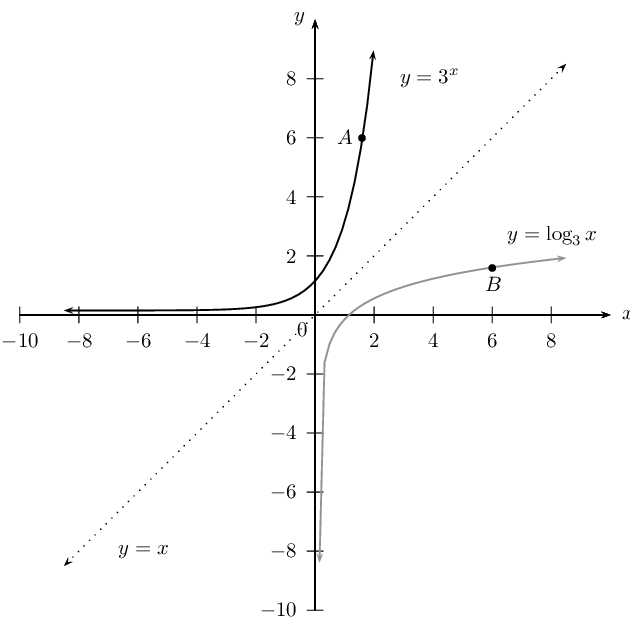
\begin{align*} \log_{2}{a} - 1 &= \text{1,5} \\ \frac{\log{a}}{\log{2}} &= \text{2,5} \\ \log{a} &= \log{2} \times \text{2,5} \\ \therefore a &= \text{5,66} \end{align*}Given \(y = 3^{x}\).
Summary (EMCFW)
-
The logarithm of a number \((x)\) with a certain base \((a)\) is equal to the exponent \((y)\), the value to which that certain base must be raised to equal the number \((x)\).
If \(x = {a}^{y}\), then \(y = {\log}_{a}\left(x\right)\), where \(a>0\), \(a \ne 1\) and \(x>0\).
-
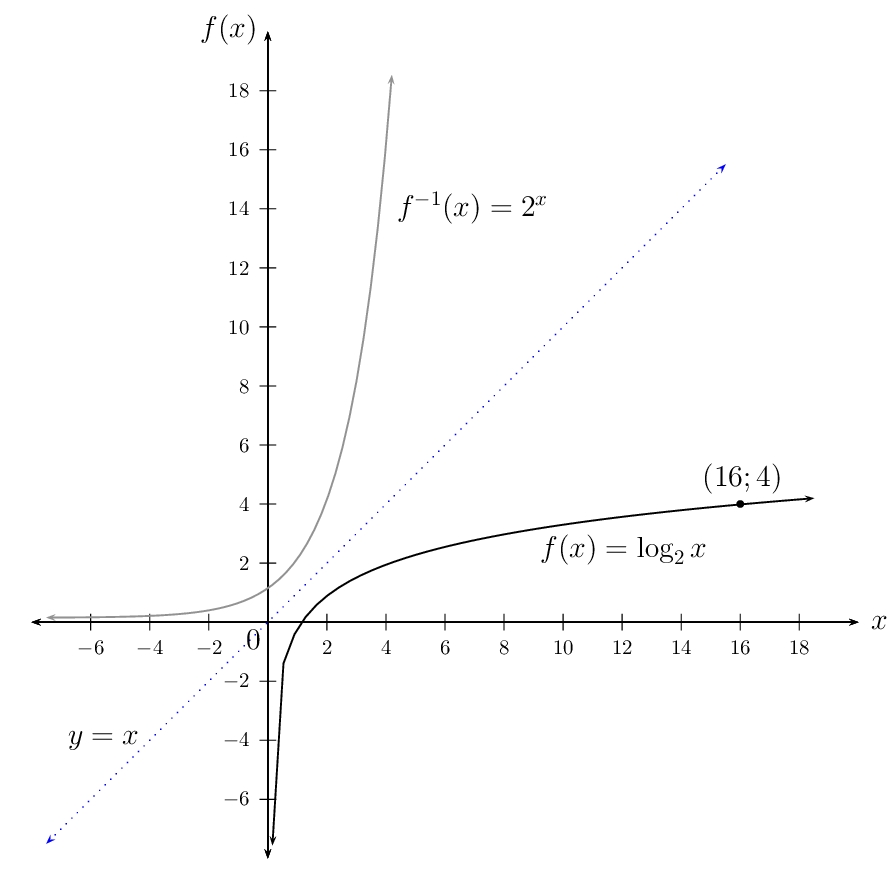
Logarithms and exponentials are inverses of each other.
\(f(x) = \log_{a}{x} \quad\) and \(\quad f^{-1}(x) = a^{x}\)
- Common logarithm: \(\log{a}\) means \(\log_{\text{10}}{a}\)
- The “LOG” function on your calculator uses a base of \(\text{10}\).
- Natural logarithm: \(\ln\) uses a base of \(e\).
-
Special values:
- \(a^{0} = 1 \qquad \log_{a}{1} = 0\)
- \(a^{1} = a \qquad \log_{a}{a} = 1\)
-
Logarithmic laws:
- \(\log_{a}{xy} = \log_{a}{x} + \log_{a}{y} \qquad (x > 0 \text{ and } y > 0)\)
- \(\log_{a}{\frac{x}{y}} = \log_{a}{x} - \log_{a}{y} \qquad (x > 0 \text{ and } y > 0)\)
- \(\log_{a}{x^{b}} = b \log_{a}{x} \qquad (x > 0)\)
- \(\log_{a}{x} = \frac{\log_{b}{x}}{\log_{b}{a}} \qquad (b > 0 \text{ and } b \ne 1)\)
-
Special reciprocal applications:
- \(\log_{a}{x} = \frac{1}{\log_{x}{a}}\)
- \(\log_{a}{\frac{1}{x}} = - \log_{a}{x}\)
Logarithms (ENRICHMENT ONLY)
State whether the following are true or false. If false, change the statement so that it is true.
False: \(\log{t} + \log{d} = \log{(t \times d)}\)
If \(p^{q} = r\), then \(q = \log_{r}{p}\)
False: \(q = \log_{p}{r}\)
True
False: \(\log{(A)} - B\) cannot be simplified further.
True
False: \(\log_{k}{m} = \frac{\log_{p}{m}}{\log_{p}{k}}\)
True
True
False: bases are different
True
False: cannot be simplified to single logarithm
False: do not confuse with \(\log{(AB)} = \log{A} + \log{B}\) or with the distributive law \(x(a + b) = ax + ab\).
False: \(\log{2a^{3}} = \log{2} + 3\log{a}\)
False: do not confuse with \(\log_{n}{ \left( \frac{a}{b} \right) } = \log_{n}{a} - \log_{n}{b}\). LHS cannot be simplified.
Simplify the following without using a calculator:
Given \(\log{5} = \text{0,7}\). Find the value of the following without using a calculator:
If \(\log{5} = \text{0,7}\) , then \(10^{\text{0,7}} = \text{5}\).
Given \(A = \log_{8}{1} - \log_{5}{\frac{1}{25}} + \log_{3}{9}\).
Solve for \(x\) if \(\frac{35^{x}}{7^{x}} = \text{15}\). Give answer correct to two decimal places.
Given \(f(x) = 5 \times \left( \text{1,5} \right)^{x}\) and \(g(x) = \left( \frac{1}{4} \right)^{x}\).
Therefore, \(x < 10, (x \in \mathbb{Z})\).
Important: notice that the inequality sign changed direction when we divided both sides by \(\log{\left( \frac{1}{4} \right)} = -\log{4}\), since it has a negative value.
|
Previous
2.7 Summary
|
Table of Contents |
Next
3.1 Calculating the period of an investment
|
