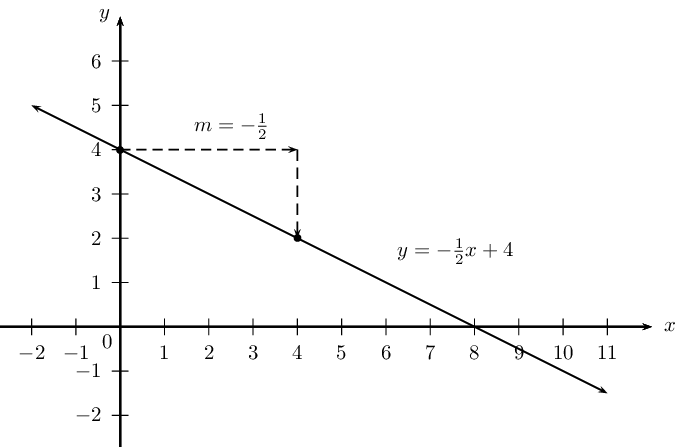
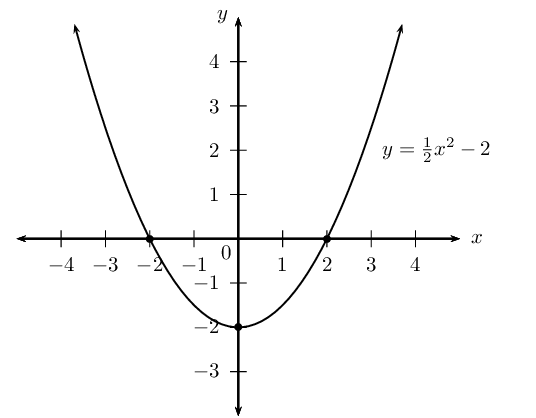
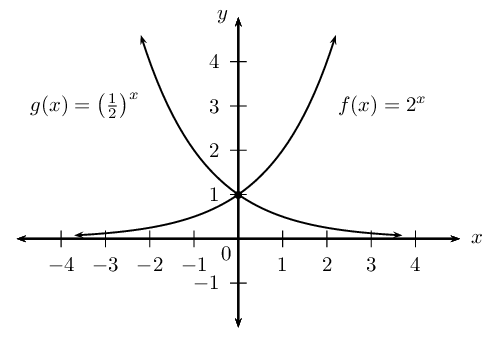
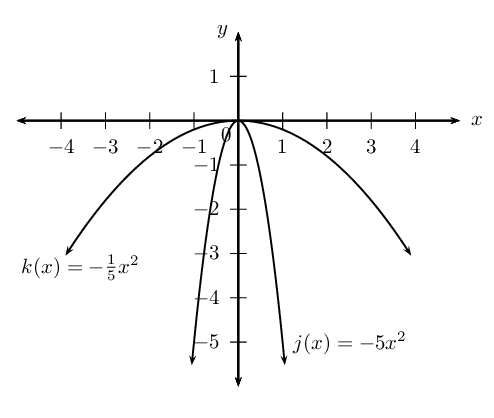
\(f(x) = 3x^{2}\) and \(g(x) = - x^{2}\)
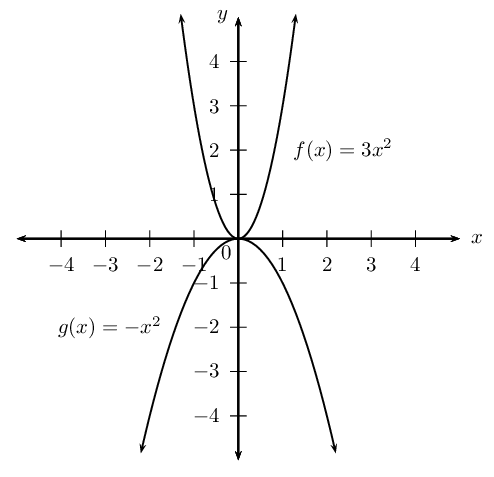
For \(f(x)\):
\begin{align*} \text{Intercept: } & (0;0) \\ \text{Turning point: } & (0;0) \\ \text{Axes of symmetry: } & x = 0 \\ \text{Domain: } & \{x: x \in \mathbb{R} \} \\ \text{Range: } & \{y: y \geq 0, y \in \mathbb{R} \} \\ \text{Minimum value: } & y = 0 \end{align*}For \(g(x)\):
\begin{align*} \text{Intercept: } & (0;0) \\ \text{Turning point: } & (0;0) \\ \text{Axes of symmetry: } & x = 0 \\ \text{Domain: } & \{x: x \in \mathbb{R} \} \\ \text{Range: } & \{y: y \leq 0, y \in \mathbb{R} \} \\ \text{Maximum value: } & y = 0 \end{align*}