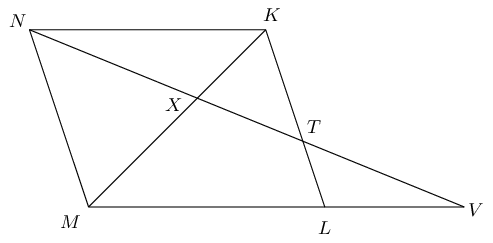
Calculate \(SV\)
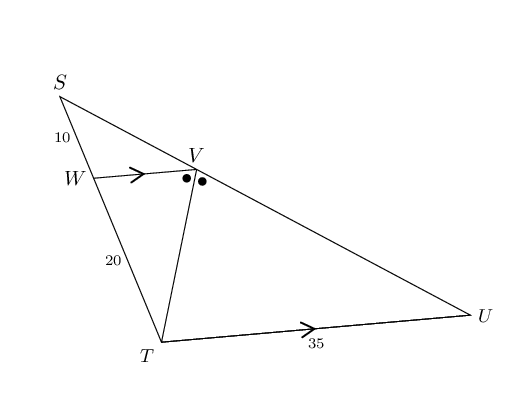
\[\begin{array}{rll}
V\hat{T}U & = W\hat{V}T & \quad \text{(alt. } \angle \text{s}, WV \parallel TU) \\
\therefore TU & = VU = 35 & \quad \text{(isosceles } \triangle \text{)} \\
\frac{SW}{WT} & = \frac{SV}{VU} & \quad \text{(proportion Theorem)} \\
\therefore SV & = \frac{SW.VU}{WT} & \\
& = \frac{(10)(35)}{20} & \\
& = \text{17,5}\text{ units} & \\
\end{array}\]
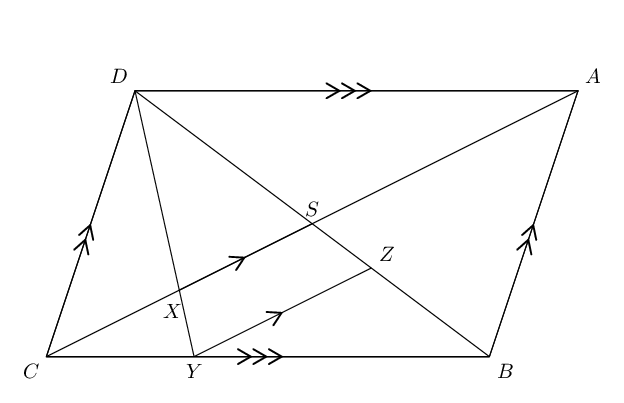
\(\frac{CB}{YB}=\frac{3}{2}\). Find \(\frac{DS}{SZ}\).
\[\begin{array}{rll}
DABC & \text{ is a parallelogram } & (DA \parallel CB \text{ and } DC \parallel AB)\\
DS &= SB & (\text{diagonals bisect})\\
& & \\
\dfrac{SZ}{ZB} &= \dfrac{CY}{YB} = \frac{3}{2} & (CS \parallel YZ)\\
& & \\
\dfrac{SZ}{SB} &= \dfrac{CY}{CB} = \frac{3}{5} & (CS \parallel YZ)\\
& & \\
\therefore SZ &= \frac{3}{5} SB & \\
& & \\
\therefore \dfrac{DS}{SZ} &= \dfrac{DS}{\frac{3}{5} SB} & (DS = SB) \\
& & \\
&= \frac{5}{3} &
\end{array}\]
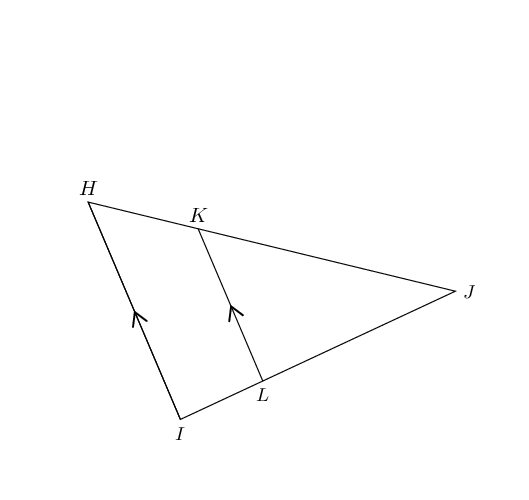
Using the following figure and lengths, find \(IJ\) and \(KJ\) (correct to one decimal place).
\(HI= \text{20}\text{ m},KL= \text{14}\text{ m}, JL=\text{18}\text{ m}\) and \(HJ=\text{32}\text{
m}\).
\[\begin{array}{rll}
\dfrac{IJ}{LJ} & = \dfrac{HI}{KL} & \quad \text{(proportion Theorem)}\\
& & \\
IJ & = \dfrac{HI}{KL}(LJ) & \\
& & \\
& = \dfrac{20}{14}(18) & \\
& & \\
& = \dfrac{180}{7} & \\
& & \\
& = \text{25,7}\text{ m} &
\end{array}\]
\[\begin{array}{rll}
KJ & = \dfrac{LJ}{IJ}(HJ) & \\
& & \\
& = \dfrac{18}{\text{25,7}}(32) & \\
& & \\
& = \text{22,4}\text{ m} & \\
\end{array}\]
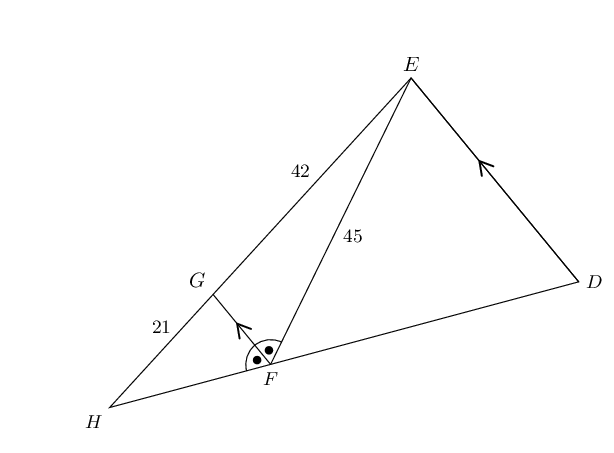
Find \(FH\) in the following figure.
\[\begin{array}{rll}
G\hat{F}H & = \hat{D} & \text{(corresp. } \angle \text{s}, GF \parallel ED) \\
G\hat{F}E & = F\hat{E}D & \text{(alt. } \angle \text{s}, GF \parallel ED) \\
\therefore F\hat{E}D & = \hat{D} & \\
\therefore EF &= FD = \text{45}\text{ cm} & \\
\frac{HF}{FD } &= \frac{21}{42} & \\
&= \frac{1}{2} & \\
\therefore HF & = \frac{1}{2}(45) & \\
& = \text{23,5}\text{ cm} &
\end{array}\]
In \(\triangle GHI\), \(GH\parallel LJ\), \(GJ\parallel LK\) and \(\frac{JK}{KI}=\frac{5}{3}\).
Determine \(\frac{HJ}{KI}\).
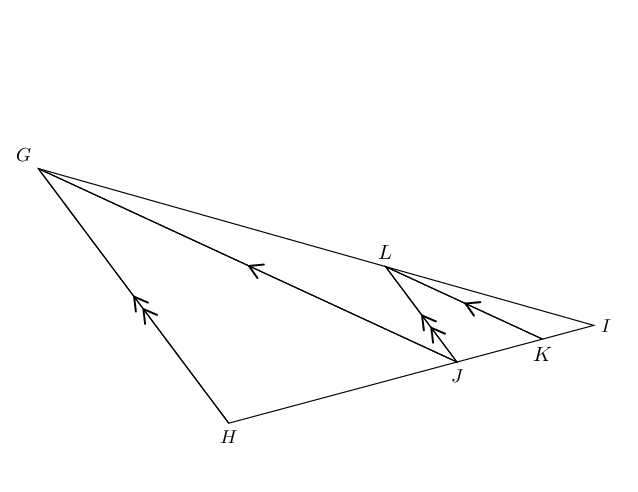
\[\begin{array}{rll} L\hat{I}J & = G\hat{I}H &\\
J\hat{L}I & = H\hat{G}I & \text{(corresp. }\angle \text{s}, HG \parallel JL) \\
\therefore \triangle LIJ & \enspace ||| \enspace \triangle GIH & \text{(Equiangular
}\triangle \text{s)}
\end{array}\]
\[\begin{array}{rll}
\frac{HJ}{JI}& =\frac{GL}{LI} & \left(\triangle LIJ \enspace ||| \enspace \triangle
GIH\right)\\
\text{and }\frac{GL}{LI}& =\frac{JK}{KI} & \left(\triangle LIK \enspace ||| \enspace
\triangle GIJ\right)\\
& =\frac{5}{3} \\
\therefore \frac{HJ}{JI}& =\frac{5}{3} \end{array}\]
\begin{align*} \frac{HJ}{KI} & = \frac{HJ}{JI} \times \frac{JI}{KI} \end{align*}
\begin{align*} JI & = JK+KI \\ & = \frac{5}{3}KI+KI \\ & = \frac{8}{3}KI \\
\frac{JI}{KI} & = \frac{8}{3} \\ & \\ \frac{HJ}{KI} & = \frac{HJ}{JI} \times
\frac{JI}{KI} \\ & = \frac{5}{3}\times \frac{8}{3} \\ & = \frac{40}{9} \end{align*}
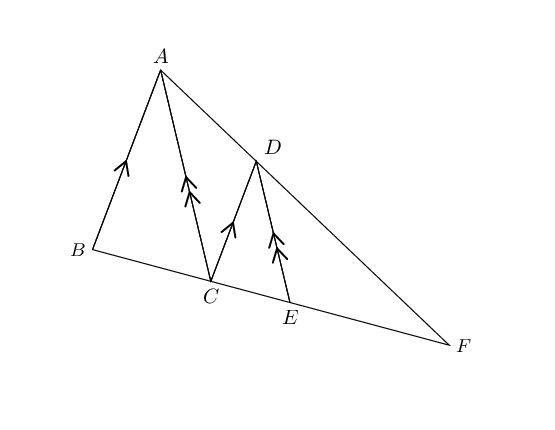
\(BF=\text{25}\text{ m}\), \(AB=\text{13}\text{ m}\), \(AD=\text{9}\text{ m}\),
\(DF=\text{18}\text{ m}\).
Calculate the lengths of \(BC\), \(CF\), \(CD\), \(CE\) and \(EF\), and find the ratio
\(\frac{DE}{AC}\).
\[\begin{array}{rll}
\frac{BC}{BF} & = \frac{AD}{AF} = \frac{9}{27} = \frac{1}{3} & (CD \parallel BA) \\
\therefore BC & = \frac{1}{3} \times 25 & \\
&= \text{8,3}\text{ m} & \\
& & \\
CF &= BF - BC & \\
&= 25 - \text{8,3} & \\
&= \text{16,7}\text{ m} & \\
& & \\
\frac{CD}{AB} &= \frac{DF}{AF} & (CD \parallel BA) \\
CD &= \frac{DF}{AF} \times AB & \\
&= \frac{18}{27} \times 13 & \\
&= \text{8,7}\text{ m} & \\
& & \\
\frac{CE}{CF} &= \frac{AD}{AF} & (DE \parallel AC) \\
CE &= \frac{AD}{AF} \times CF & \\
&= \frac{9}{27} \times \text{16,7} & \\
&= \text{5,6}\text{ m} & \\
& & \\
EF &= BF - (BC + CE) & \\
&= 25 - (\text{8,3} - \text{5,6}) & \\
&= \text{11,1}\text{ m} &
\end{array}\]
In \(\triangle XYZ\), \(X\hat{Y}Z =\text{90}°\) and \(YT \perp XZ\). If \(XY =
\text{14}\text{ cm}\) and \(XT = \text{4}\text{ cm}\), determine \(XZ\) and \(YZ\) (correct to
two decimal places).
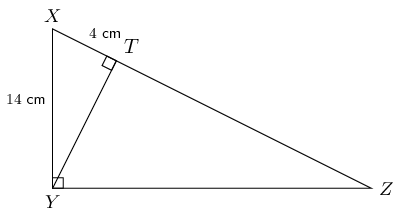
Use the theorem of Pythagoras to determine \(YT\):
\[\begin{array}{rll}
\text{In } \triangle XTY, \quad YT^{2} &= XY^{2} - XT^{2} & (\text{Pythagoras}) \\
&= 14^{2} - 4^{2} & \\
&= 196 - 16 & \\
\therefore YT &= \sqrt{180} & \\
&= \sqrt{36 \times 5} & \\
&= 6 \sqrt{5} ~\text{cm} &
\end{array}\]
Use proportionality to determine \(XZ\) and \(YZ\):
\[\begin{array}{rll}
X\hat{Y}Z &= \text{90}° & \text{(given)} \\
YT &\perp XZ & \text{(given)} \\
\therefore \enspace \triangle XYT \enspace & ||| \enspace \triangle YZT \enspace ||| \enspace
\triangle XZY & (\text{right-angled } \triangle \text{s}) \\
& & \\
\therefore \dfrac{YT}{TZ} &= \dfrac{XT}{YT} & (\triangle YZT \enspace ||| \enspace \triangle
XYT ) \\
& & \\
\therefore YT^{2} &= TZ \cdot XT & \\
& & \\
\left( 6 \sqrt{5} \right)^{2} &= TZ \cdot 4 & \\
& & \\
\therefore TZ &= 45 & \\
& & \\
\text{And } XZ &= XT + TZ & \\
&= 4 + 45 & \\
&= \text{49}\text{ cm} & \\
& & \\
\text{In } \triangle XYZ, \quad YZ^{2} &= XZ^{2} - XY^{2} & (\text{Pythagoras}) \\
&= 49^{2} - 14^{2} & \\
\therefore YZ &= \sqrt{2205} & \\
&= \text{46,96}\text{ cm} &
\end{array}\]
Given the following figure with the following lengths, find \(AE\), \(EC\) and \(BE\).
\(BC=15\) cm, \(AB=4\) cm, \(CD=18\) cm, and \(ED=9\) cm.
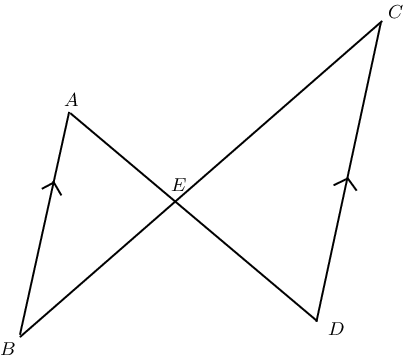
\[\begin{array}{rll}
B\hat{A}E & = C\hat{D}E & \text{(alt. } \angle \text{s}, AB \parallel CD) \\
A\hat{B}E & = D\hat{C}E & \text{(alt. } \angle \text{s}, AB \parallel CD) \\
A\hat{E}B & = D\hat{E}C & \text{(vert. opp. } \angle \text{s)}\\
\therefore \triangle AEB & \enspace ||| \enspace \triangle DEC & \text{(AAA)}\\
\therefore \frac{AE}{DE} & = \frac{AB}{DC} = \frac{4}{18} = \frac{2}{9} & (\triangle AEB
\enspace ||| \enspace \triangle DEC) \\
& & \\
AE & = \dfrac{2}{9} DE & \\
& & \\
& = \dfrac{2}{9} (9) & \\
& & \\
& = \text{2}\text{ cm} & \\
\end{array}\]
\[\begin{array}{rll}
\frac{EC}{BC} & = \dfrac{ED}{AD} = \dfrac{9}{11} & (AB \parallel CD) \\
& & \\
EC & = \dfrac{ED}{AD}(BC) & \\
& & \\
& = \dfrac{9}{11}(15) & \\
& & \\
& = \text{12,3}\text{ cm} & \\
\end{array}\]
\[\begin{array}{rll}
\frac{BE}{BC} & = \dfrac{AE}{AD} = \dfrac{2}{11} & (AB \parallel CD) \\
& & \\
BE & = \dfrac{AE}{AD}(BC) & \\
& & \\
& = \dfrac{2}{11}(15) & \\
& & \\
& = \text{2,7}\text{ cm} & \\
\end{array}\]
\(SNRE\) is a cyclic quadrilateral
\[\begin{array}{rll}
M\hat{S}N&= \text{90}° & (\angle \text{ in semi-circle}) \\
N\hat{R}E&=\text{90}° & (\text{given}) \\
\therefore SNRE&\text{ is a cyclic quad. } & (\text{ext. }\angle = \text{ opp. int. }
\angle)
\end{array}\]
\[\begin{array}{rll}
N\hat{S}R&=\hat{M} = x & (\text{tangent/chord}) \\
\therefore E\hat{S}R&= \text{90}° - x & \\
\hat{E}&= \text{90}° - x & (MRE = \text{90}°, \enspace \hat{M} = x) \\
\therefore E\hat{S}R&= \hat{E} & \\
\therefore RS&= RE & (\text{isos. } \triangle)
\end{array}\]
\(\triangle MSN \enspace ||| \enspace \triangle MRE\)
In \(\triangle MSN\) and \(\triangle MRE\):
\[\begin{array}{rll}
\hat{M} &=\hat{M} & \\
M\hat{S}N &= \text{90}° & (\angle \text{ in semi-circle}) \\
M\hat{R}E &= \text{90}° & (\text{given}) \\
\therefore M\hat{S}N &= M\hat{R}E & \\
\therefore \triangle MSN& \enspace ||| \enspace \triangle MRE & (\text{AAA})
\end{array}\]
\(\triangle RSN \enspace ||| \enspace \triangle RMS\)
In \(\triangle RSN\) and \(\triangle RMS\):
\[\begin{array}{rll}
\hat{R}&= \hat{R} & (\text{common } \angle) \\
R\hat{S}N&= \hat{M} & (\text{tangent/chord}) \\
\therefore \triangle RSN & \enspace ||| \enspace \triangle RMS & (\text{AAA})
\end{array}\]
\[\begin{array}{rll}
\dfrac{RS}{RN} &= \dfrac{RM}{RS} & \\
RS^{2} &= RN.RM & \\
\text{But } RS &= RE & \\
RE^{2} &= RN.RM &
\end{array}\]
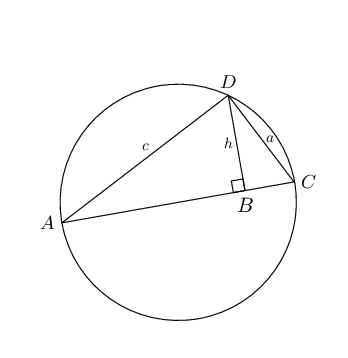
Prove that \(h = \dfrac{ac}{d}\).
\[\begin{array}{rll}
\triangle ADB& \enspace ||| \enspace \triangle DCB \enspace ||| \enspace \triangle ACD &
(A\hat{D}C = \text{90}°, DB \perp AC) \\
\therefore \dfrac{DB}{AD}&= \dfrac{CD}{AC} & \\
\therefore \dfrac{h}{c}&= \dfrac{a}{d} & \\
\therefore h&= \dfrac{ac}{d} &
\end{array}\]
Hence, deduce that \(\enspace \dfrac{1}{h^{2}}=\dfrac{1}{a^{2}}+\dfrac{1}{c^{2}}\).
\[\begin{array}{rll}
h^{2}&= \dfrac{a^{2}c^{2}}{d^{2}} & \\
\text{But } d^{2}&= a^{2} + c^{2} & (\text{In } \triangle ADC, \hat{D} =
\text{90}°, \text{ Pythagoras}) \\
\therefore h^{2}&= \dfrac{a^{2}c^{2}}{a^{2}+c^{2}} & \\
\therefore \dfrac{1}{h^{2}}&= \dfrac{a^{2}+c^{2}}{a^{2}c^{2}} & \\
\dfrac{1}{h^{2}}&= \dfrac{a^{2}}{a^{2}c^{2}} + \dfrac{c^{2}}{a^{2}c^{2}} & \\
\dfrac{1}{h^{2}}&= \dfrac{1}{c^{2}} + \dfrac{1}{a^{2}} &
\end{array}\]
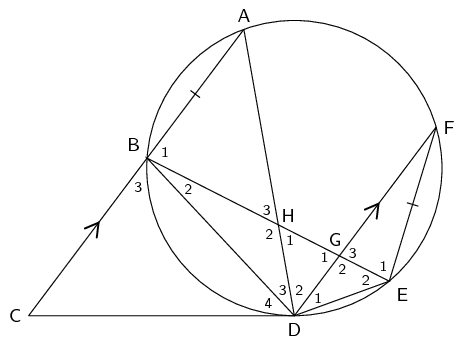
\(AC \text{ bisects } E\hat{A}F\)
\[\begin{array}{rll}
BC &= CD & (\text{given}) \\
\therefore B\hat{A}C &= D\hat{A}C & (\angle \text{s on equal chords})
\end{array}\]
\[\begin{array}{rll}
D\hat{C}F&= \hat{A_{2}} & (\text{tangent/chord}) \\
B\hat{D}C&= \hat{A_{1}} & (\angle \text{s on same chord}) \\
\hat{A_{1}} &= \hat{A_{2}} & (\text{proved in (a)}) \\
\therefore B\hat{D}C &= D\hat{C}F & \\
\therefore BD & \parallel EF & (\text{alt. } \angle \text{s are equal})
\end{array}\]
\(\triangle ADC \enspace ||| \enspace \triangle CBE\)
In \(\triangle ADC\) and \(\triangle CBE\):
\[\begin{array}{rll}
\hat{A_{2}} &= D\hat{B}C & (\angle \text{s on chord } CD) \\
&= B\hat{C}E & (\text{alt. } \angle \text{s, } BD \parallel EF) \\
A\hat{D}C &= E\hat{B}C & (\text{ext. } \angle \text{ of a cyclic quad}) \\
\therefore \triangle ADC & \enspace ||| \enspace \triangle CBE & (\text{AAA})
\end{array}\]
\[\begin{array}{rll}
\dfrac{DC}{AD} &= \dfrac{BE}{BC} & (\triangle ADC \enspace ||| \enspace \triangle CBE)
\\
\text{But } DC &= BC & (\text{given}) \\
\therefore \dfrac{DC}{AD} &= \dfrac{BE}{DC} & \\
\therefore DC^{2} &= AD.BE &
\end{array}\]