9.2 Simple and compound depreciation | Finance growth and decay

9.2 Simple and compound depreciation (EMBJF)

As soon as a new car leaves the dealership, its value decreases and it is considered “second-hand”. Vehicles, equipment, machinery and other similar assets, all lose value over time as a result of usage and age. This loss in value is called depreciation. Assets that have a relatively long useful lifetime, such as machines, trucks, farming equipment etc., depreciate slower than assets like office equipment, computers, furniture etc. which need to be replaced more often and therefore depreciate more quickly.

Depreciation is used to calculate the value of a company's assets, which determines how much tax a company must pay. Companies can take depreciation into account as an expense, and thereby reduce their taxable income. A lower taxable income means that the company will pay less income tax to SARS (South African Revenue Service).

We can calculate two different kinds of depreciation: simple decay and compound decay. Decay is also a term used to describe a reduction or decline in value. Simple decay is also called straight-line depreciation and compound decay can also be referred to as reducing-balance depreciation. In the straight-line method the value of the asset is reduced by a constant amount each year, which is calculated on the principal amount. In reducing-balance depreciation we calculate the depreciation on the reduced value of the asset. This means that the value of an asset decreases by a different amount each year.

Simple and compound depreciation

Mr. Sontange buys an Opel Fiesta for \(\text{R}\,\text{72 000}\). He expects that the value of the car will depreciate by \(\text{R}\,\text{6 000}\) every year. He draws up a table to calculate the depreciated value of his Opel Fiesta.

Complete Mr. Sontange's table of values for the \(\text{7}\) year period:

Year	Value at beginning of year	Depreciation amount	Value at end of year
\(\text{1}\)	\(\text{R}\,\text{72 000}\)	\(\text{R}\,\text{6 000}\)	\(\text{R}\,\text{66 000}\)
\(\text{2}\)	\(\text{R}\,\text{66 000}\)	\(\text{R}\,\text{6 000}\)
\(\text{3}\)
\(\text{4}\)
\(\text{5}\)
\(\text{6}\)
\(\text{7}\)

His son, David, does not agree that the value of the car will reduce by the same amount each year. David thinks that the car will depreciate by \(\text{10}\%\) every year.

Complete David's table of values:

Year	Value at beginning of year	Depreciation amount	Value at end of year
\(\text{1}\)	\(\text{R}\,\text{72 000}\)	\(\text{R}\,\text{7 200}\)	\(\text{R}\,\text{64 800}\)
\(\text{2}\)	\(\text{R}\,\text{64 800}\)	\(\text{R}\,\text{6 480}\)
\(\text{3}\)
\(\text{4}\)
\(\text{5}\)
\(\text{6}\)
\(\text{7}\)

Compare and discuss the results of the two different tables.
Consider the graph below, which represents Mr. Sontange's table of values:
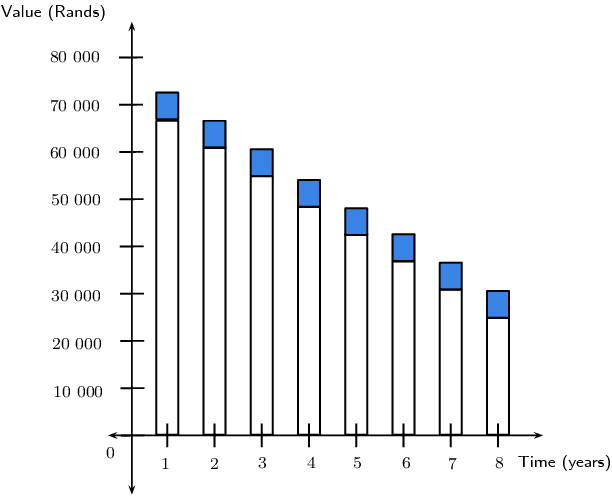
1. Draw a similar graph using David's table of values.
2. Interpret the two graphs and discuss the differences between them.
3. Explain how the graphs can be used to determine the total depreciation in each case.
4. 1. Draw two new graphs by plotting the maximum value of each bar.
  2. Join the points with a line to show the general trend.
  3. Is it mathematically correct to join these points? Explain your answer.

Simple depreciation (EMBJG)

Worked example 3: Straight-line depreciation

A new smartphone costs \(\text{R}\,\text{6 000}\) and depreciates at \(\text{22}\%\) p.a. on a straight-line basis. Determine the value of the smartphone at the end of each year over a \(\text{4}\) year period.

Calculate depreciation amount

\begin{align*} \text{Depreciation} &= \text{6 000} \times \frac{22}{\text{100}} \\ &= \text{1 320} \end{align*}

Therefore the smartphone depreciates by \(\text{R}\,\text{1 320}\) every year.

Complete a table of values

Year	Value at beginning of year	Depreciation amount	Value at end of year
\(\text{1}\)	\(\text{R}\,\text{6 000}\)	\(\text{R}\,\text{1 320}\)	\(\text{R}\,\text{4 680}\)
\(\text{2}\)	\(\text{R}\,\text{4 680}\)	\(\text{R}\,\text{1 320}\)	\(\text{R}\,\text{3 360}\)
\(\text{3}\)	\(\text{R}\,\text{3 360}\)	\(\text{R}\,\text{1 320}\)	\(\text{R}\,\text{2 040}\)
\(\text{4}\)	\(\text{R}\,\text{2 040}\)	\(\text{R}\,\text{1 320}\)	\(\text{R}\,\text{720}\)

We notice that

\[\text{Total depreciation} = P \times i \times n\]

where

\begin{align*} P &= \text{principal amount} \\ i &= \text{interest rate written as a decimal} \\ n &= \text{time period in years} \end{align*}

Therefore the depreciated value of the asset (also called the book value) can be calculated as:

\[A = P(1 - in)\]

Note the similarity to the simple interest formula \(A = P(1 + in)\). Interest increases the value of the principal amount, whereas with simple decay, depreciation reduces the value of the principal amount.

Important: to get an accurate answer do all calculations in one step on your calculator. Do not round off answers in your calculations until the final answer. In the worked examples in this chapter, we use dots to show that the answer has not been rounded off. We always round the final answer to two decimal places (cents).

Worked example 4: Straight-line depreciation method

A car is valued at \(\text{R}\,\text{240 000}\). If it depreciates at \(\text{15}\%\) p.a. using straight-line depreciation, calculate the value of the car after \(\text{5}\) years.

Write down the known variables and the simple decay formula

\begin{align*} P &= \text{240 000} \\ i &= \text{0,15} \\ n &= 5 \end{align*}\[A = P(1 - in)\]

Substitute the values and solve for \(A\)

\begin{align*} A &= \text{240 000}(1 - \text{0,15} \times 5) \\ &= \text{240 000}(\text{0,25}) \\ &= \text{60 000} \end{align*}

Write the final answer

At the end of \(\text{5}\) years, the car is worth \(\text{R}\,\text{60 000}\).

temp text

Worked example 5: Simple decay

A small business buys a photocopier for \(\text{R}\,\text{12 000}\). For the tax return the owner depreciates this asset over \(\text{3}\) years using a straight-line depreciation method. What amount will he fill in on his tax form at the end of each year?

Write down the known variables

The owner of the business wants the photocopier to have a book value of \(\text{R}\,\text{0}\) after \(\text{3}\) years.

\begin{align*} A &= 0 \\ P &= \text{12 000} \\ n &= 3 \end{align*}

Therefore we can calculate the annual depreciation as \begin{align*} \text{Depreciation} &= \frac{P}{n} \\ &= \frac{\text{12 000}}{3} \\ &= \text{R}\,\text{4 000} \end{align*}

Determine the book value at the end of each year

\begin{align*} \text{Book value end of first year} &= \text{12 000} - \text{4 000} \\ &= \text{R}\,\text{8 000} \\ \\ \text{Book value end of second year} &= \text{8 000} - \text{4 000} \\ &= \text{R}\,\text{4 000} \\ \\ \text{Book value end of third year} &= \text{4 000} - \text{4 000} \\ &= \text{R}\,\text{0} \end{align*}

Simple decay

Textbook Exercise 9.2

A business buys a truck for \(\text{R}\,\text{560 000}\). Over a period of \(\text{10}\) years the value of the truck depreciates to \(\text{R}\,\text{0}\) using the straight-line method. What is the value of the truck after \(\text{8}\) years?

\begin{align*} \text{Depreciation} &= \frac{\text{560 000}}{10} \\ &= \text{R}\,\text{56 000}\text{ per year} \\ \text{For } n &= 8 \\ A &= \text{560 000} - 8(\text{56 000}) \\ &= \text{R}\,\text{112 000} \end{align*}

Harry wants to buy his grandpa's donkey for \(\text{R}\,\text{800}\). His grandpa is quite pleased with the offer, seeing that it only depreciated at a rate of \(\text{3}\%\) per year using the straight-line method. Grandpa bought the donkey \(\text{5}\) years ago. What did grandpa pay for the donkey then?

\begin{align*} A &= P(1 - in) \\ \text{800} &= P(1 - (\text{0,03} \times 5)) \\ \therefore \frac{\text{800}}{\text{0,85}} &= P \\ \therefore P &= \text{R}\,\text{941,18} \end{align*}

Seven years ago, Rocco's drum kit cost him \(\text{R}\,\text{12 500}\). It has now been valued at \(\text{R}\,\text{2 300}\). What rate of simple depreciation does this represent?

\begin{align*} A &= P(1 - in) \\ \text{2 300} &= \text{12 500}(1 - (i \times 7)) \\ \therefore \frac{\text{2 300}}{\text{12 500}} &= 1 - 7i \\ \text{0,184} - 1 &= - 7i \\ \frac{-\text{0,816}}{-7} &= i \\ \therefore i &= \text{11,66}\% \end{align*}

Fiona buys a DStv satellite dish for \(\text{R}\,\text{3 000}\). Due to weathering, its value depreciates simply at \(\text{15}\%\) per annum. After how long will the satellite dish have a book value of zero?

\begin{align*} \text{Depreciation} &= \text{3 000} \times \frac{15}{\text{100}} \\ &= \text{R}\,\text{450}\text{ per year} \\ \therefore n &= \frac{\text{3 000}}{\text{450}} \\ &= \text{6,666} \ldots \\ \therefore n &= \text{7}\text{ years} \end{align*}

\begin{align*} A &= P(1 - in) \\ 0 &= \text{3 000}(1 - \text{0,15} \times n) \\ \therefore 0 &= 1 - \text{0,15}n \\ \text{0,15}n &= 1 \\ n &= \frac{1}{\text{0,15}} \\ &= \text{6,666} \ldots \\ \therefore n &= \text{7}\text{ years} \end{align*}

Compound depreciation (EMBJH)

Worked example 6: Reducing-balance depreciation

A second-hand farm tractor worth \(\text{R}\,\text{60 000}\) has a limited useful life of \(\text{5}\) years and depreciates at \(\text{20}\%\) p.a. on a reducing-balance basis. Determine the value of the tractor at the end of each year over the \(\text{5}\) year period.

Write down the known variables

\begin{align*} P &= \text{60 000} \\ i &= \text{0,2} \\ n &= 5 \end{align*}

When we calculate depreciation using the reducing-balance method:

the depreciation amount changes for each year.
the depreciation amount gets smaller each year.
the book value at the end of a year becomes the principal amount for the next year.
the asset will always have some value (the book value will never equal zero).

Complete a table of values

Year	Book value	Depreciation	Value at end of year
\(\text{1}\)	\(\text{R}\,\text{60 000}\)	\(\text{60 000} \times \text{0,2} = \text{12 000}\)	\(\text{R}\,\text{48 000}\)
\(\text{2}\)	\(\text{R}\,\text{48 000}\)	\(\text{48 000} \times \text{0,2} = \text{9 600}\)	\(\text{R}\,\text{38 400}\)
\(\text{3}\)	\(\text{R}\,\text{38 400}\)	\(\text{38 400} \times \text{0,2} = \text{7 680}\)	\(\text{R}\,\text{30 720}\)
\(\text{4}\)	\(\text{R}\,\text{30 720}\)	\(\text{30 720} \times \text{0,2} = \text{6 144}\)	\(\text{R}\,\text{24 576}\)
\(\text{5}\)	\(\text{R}\,\text{24 576}\)	\(\text{24 576} \times \text{0,2} = \text{4 915,20}\)	\(\text{R}\,\text{19 660,80}\)

Notice in the example above that we could also write the book value at the end of each year as:

\[\begin{array}{l@{\;}l@{\;}l} \text{Book value end of first year} &= \text{60 000}(1 - \text{0,2}) & \\ \text{Book value end of second year} &= \text{48 000}(1 - \text{0,2}) &= \text{60 000} (1 - \text{0,2})^2 \\ \text{Book value end of third year} &= \text{38 400}(1 - \text{0,2}) &= \text{60 000} (1 - \text{0,2})^3 \\ \text{Book value end of fourth year} &= \text{30 720}(1 - \text{0,2}) &= \text{60 000} (1 - \text{0,2})^4 \\ \text{Book value end of fifth year} &= \text{24 576}(1 - \text{0,2}) &= \text{60 000} (1 - \text{0,2})^5 \end{array}\]

Using the formula for simple decay and the observed pattern in the calculation above, we obtain the following formula for compound decay:

\[A = P(1 - i)^n\]

where

\begin{align*} A &= \text{book value or depreciated value} \\ P &= \text{principal amount} \\ i &= \text{interest rate written as a decimal} \\ n &= \text{time period in years} \end{align*}

Again, notice the similarity to the compound interest formula \(A = P(1 + i)^n\).

Worked example 7: Reducing-balance depreciation

The number of pelicans at the Berg river mouth is decreasing at a compound rate of \(\text{12}\%\) p.a. If there are currently \(\text{3 200}\) pelicans in the wetlands of the Berg river mouth, what will the population be in \(\text{5}\) years?

Write down the known variables and the compound decay formula

\begin{align*} P &= \text{3 200} \\ i &= \text{0,12} \\ n &= 5 \end{align*}\[A = P(1 - i)^n\]

Substitute the values and solve for \(A\)

\begin{align*} A &= \text{3 200}(1 - \text{0,12})^5 \\ &= \text{3 200}(\text{0,88})^5 \\ &= \text{1 688,7421} \ldots \end{align*}

Write the final answer

In \(\text{5}\) years, the pelican population will be approximately \(\text{1 689}\).

temp text

Worked example 8: Compound decay

A school buys a minibus for \(\text{R}\,\text{950 000}\), which depreciates at \(\text{13,5}\%\) per annum. Determine the value of the minibus after \(\text{3}\) years if the depreciation is calculated:
1. on a straight-line basis.
2. on a reducing-balance basis.
Which is the better option?

Write down known variables

\begin{align*} P &= \text{950 000} \\ i &= \text{0,135} \\ n &= 3 \end{align*}

Use the simple decay formula and solve for \(A\)

\begin{align*} A &= \text{950 000}(1 - 3 \times \text{0,135}) \\ &= \text{950 000}(\text{0,865}) \\ &= \text{565 250} \\ \therefore A &= \text{R}\,\text{565 250} \end{align*}

Use the compound decay formula and solve for \(A\)

\begin{align*} A &= \text{950 000}(1 - \text{0,135})^3 \\ &= \text{950 000}(\text{0,865})^3 \\ &= \text{614 853,89} \\ \therefore A &= \text{R}\,\text{614 853,89} \end{align*}

Interpret the answers

After a period of \(\text{3}\) years, the value of the minibus calculated on the straight-line method is less than the value of the minibus calculated on the reducing-balance method. The value of the minibus depreciated less on the reducing-balance basis because the amount of depreciation is calculated on a smaller amount every year, whereas the straight-line method is based on the full value of the minibus every year.

Worked example 9: Compound depreciation

Farmer Jack bought a tractor and it has depreciated by \(\text{20}\%\) p.a. on a reducing-balance basis. If the current value of the tractor is \(\text{R}\,\text{52 429}\), calculate how much Farmer Jack paid for his tractor if he bought it \(\text{7}\) years ago.

Write down known variables and compound decay formula

\begin{align*} A &= \text{52 429} \\ i &= \text{0,2} \\ n &= 7 \end{align*}\[A = P(1 - i)^n\]

Substitute the values and solve for \(P\)

\begin{align*} \text{52 429} &= P(1 - \text{0,2})^7 \\ &= P(\text{0,8})^7 \\ \therefore P &= \frac{\text{52 429}}{(\text{0,8})^7} \\ &= \text{250 000,95} \ldots \end{align*}

Write the final answer

\(\text{7}\) years ago, Farmer Jack paid \(\text{R}\,\text{250 000}\) for his tractor.

Compound depreciation

Textbook Exercise 9.3

Jwayelani buys a truck for \(\text{R}\,\text{89 000}\) and depreciates it by \(\text{9}\%\) p.a. using the compound depreciation method. What is the value of the truck after \(\text{14}\) years?

\begin{align*} A &= P(1 - i)^n \\ &= \text{89 000}(1 - \text{0,09})^{14} \\ &= \text{89 000}(\text{0,91})^{14} \\ \therefore A &= \text{R}\,\text{23 766,73} \end{align*}

The number of cormorants at the Amanzimtoti river mouth is decreasing at a compound rate of \(\text{8}\%\) p.a. If there are now \(\text{10 000}\) cormorants, how many will there be in \(\text{18}\) years' time?

\begin{align*} A &= P(1 - i)^n \\ &= \text{10 000}(1 - \text{0,08})^{18} \\ &= \text{10 000}(\text{0,92})^{18} \\ &= \text{2 229,36} \ldots \\ \therefore A &= \text{2 229}\text{ cormorants} \end{align*}

On January \(\text{1}\), \(\text{2 008}\) the value of my Kia Sorento is \(\text{R}\,\text{320 000}\). Each year after that, the car's value will decrease \(\text{20}\%\) of the previous year's value. What is the value of the car on January \(\text{1}\), \(\text{2 012}\)?

\begin{align*} A &= P(1 - i)^n \\ &= \text{320 000}(1 - \text{0,2})^4 \\ &= \text{320 000}(\text{0,8})^4 \\ \therefore A &= \text{R}\,\text{131 072} \end{align*}

The population of Bonduel decreases at a reducing-balance rate of \(\text{9,5}\%\) per annum as people migrate to the cities. Calculate the decrease in population over a period of \(\text{5}\) years if the initial population was \(\text{2 178 000}\).

\begin{align*} A &= P(1 - i)^n \\ &= \text{2 178 000}(1 - \text{0,095})^5 \\ &= \text{2 178 000}(\text{0,905})^5 \\ \therefore A &= \text{132 221} \end{align*}

A \(\text{20}\) \(\text{kg}\) watermelon consists of \(\text{98}\%\) water. If it is left outside in the sun it loses \(\text{3}\%\) of its water each day. How much does it weigh after a month of \(\text{31}\) days?

\begin{align*} A &= P(1 - i)^n \\ A &= \left( 20 \times \frac{98}{\text{100}} \right) (1 - \text{0,03})^{31} \\ &= \text{19,6}(\text{0,97})^{31} \\ \therefore A &= \text{7,62}\text{ kg} \end{align*}

Richard bought a car \(\text{15}\) years ago and it depreciated by \(\text{17}\%\) p.a. on a compound depreciation basis. How much did he pay for the car if it is now worth \(\text{R}\,\text{5 256}\)?

\begin{align*} A &= P(1 - i)^n \\ \text{5 256} &= P (1 - \text{0,17})^{15} \\ \frac{\text{5 256}}{{\text{0,83}}^{15}}&= P\\ \therefore P &= \text{R}\,\text{85 997,13} \end{align*}

Finding \(i\) (EMBJJ)

Worked example 10: Finding \(i\) for simple decay

After \(\text{4}\) years, the value of a computer is halved. Assuming simple decay, at what annual rate did it depreciate? Give your answer correct to two decimal places.

Write down known variables and simple decay formula

Let the value of the computer be \(x\), therefore:

\begin{align*} A &= \frac{x}{2} \\ P &= x \\ n &= 4 \end{align*}\[A = P(1 - in)\]

Substitute the values and solve for \(i\)

\begin{align*} \frac{x}{2} &= x (1 - 3i) \\ \frac{1}{2} &= 1 - 3i \\ \therefore 3i &= 1 - \frac{1}{2} \\ \therefore i &= \text{0,1667} \end{align*}

Write the final answer

The computer depreciated at a rate of \(\text{16,67}\%\) p.a.

temp text

Worked example 11: Finding \(i\) for compound decay

Cristina bought a fridge at the beginning of \(\text{2 009}\) for \(\text{R}\,\text{8 999}\) and sold it at the end of \(\text{2 011}\) for \(\text{R}\,\text{4 500}\). At what rate did the value of her fridge depreciate assuming a reducing-balance method? Give your answer correct to two decimal places.

Write down known variables and compound decay formula

\begin{align*} A &= \text{4 500} \\ P &= \text{8 999} \\ n &= 3 \end{align*}\[A = P(1 - i)^n\]

Substitute the values and solve for \(i\)

\begin{align*} \text{4 500} &= \text{8 999} (1 - i)^3 \\ \frac{\text{4 500}}{\text{8 999}}&= (1 - i)^3 \\ \sqrt[3]{\frac{\text{4 500}}{\text{8 999}}} &= 1 - i \\ \therefore i &= 1 - \sqrt[3]{\frac{\text{4 500}}{\text{8 999}}} \\ &= \text{0,206} \end{align*}

Write the final answer

Cristina's fridge depreciated at a rate of \(\text{20,6}\%\) p.a.

temp text

Finding \(i\)

Textbook Exercise 9.4

A machine costs \(\text{R}\,\text{45 000}\) and has a scrap value of \(\text{R}\,\text{9 000}\) after \(\text{10}\) years. Determine the annual rate of depreciation if it is calculated on the reducing balance method.

\begin{align*} A &= P(1 - i)^n \\ \text{9 000} &= \text{45 000} (1 - i)^{10} \\ \frac{\text{9 000}}{\text{45 000}}&= (1 - i)^{10} \\ \sqrt[10]{\frac{\text{9 000}}{\text{45 000}}} - 1 &= -i \\ \therefore i &= \text{14,9}\% \end{align*}

After \(\text{15}\) years, an aeroplane is worth \(\frac{1}{6}\) of its original value. At what annual rate was depreciation compounded?

\begin{align*} A &= P(1 - i)^n \\ \frac{1}{6}P &= P (1 - i)^{15} \\ \frac{1}{6} &= (1 - i)^{15} \\ \sqrt[10]{\frac{1}{6}} - 1 &= -i \\ \therefore i &= \text{16,4}\% \end{align*}

Mr. Mabula buys furniture for \(\text{R}\,\text{20 000}\). After \(\text{6}\) years he sells the furniture for \(\text{R}\,\text{9 300}\). Calculate the annual compound rate of depreciation of the furniture.

\begin{align*} A &= P(1 - i)^n \\ \text{9 300} &= \text{20 000}(1 - i)^6 \\ \frac{\text{9 300}}{\text{20 000}} &= (1 - i)^6 \\ \sqrt[6]{\frac{\text{9 300}}{\text{20 000}}} - 1 &= -i \\ \therefore i &= \text{12,0}\% \end{align*}

Ayanda bought a new car \(\text{7}\) years ago for double what it is worth today. At what yearly compound rate did her car depreciate?

\begin{align*} A &= P(1 - i)^n \\ \frac{1}{2} P &= P(1 - i)^7 \\ \frac{1}{2}&= (1 - i)^7 \\ \frac{1}{2}&= (1 - i)^7 \\ \sqrt[7]{\frac{1}{2}} - 1 &= -i \\ \therefore i &= \text{9,4}\% \end{align*}

9.1 Revision

Table of Contents

9.3 Timelines