Which one of the following is not a unit of impulse?
-
\(\text{N·s}\)
-
\(\text{kg·m·s$^{-1}$}\)
-
\(\text{J·m·s$^{-1}$}\)
-
\(\text{J·m$^{-1}$·s}\)
\(\text{J·m·s$^{-1}$}\)
|
Previous
2.4 Conservation of momentum
|
Next
2.6 Physics in action: Impulse
|
When a net force acts on a body it will result in an acceleration which alters the motion of the body. A large net force will cause a larger acceleration than a small net force. The total change in motion of the object can be the same if the large and small forces act for different time intervals. The combination of the force and time that it acts is a useful quantity which leads us to define impulse.
Impulse is the product of the net force and the time interval for which the force acts.
\[\text{Impulse}=\vec{F}_{net}·\Delta t\]However, from Newton's Second Law, we know that
\begin{align*} \vec{F}_{net}& = \frac{\Delta \vec{p}}{\Delta t} \\ \therefore \vec{F}_{net}·\Delta t& = \Delta \vec{p} \\ & = \text{Impulse} \end{align*}Therefore we can define the impulse-momentum theorem:
\[\text{Impulse}=\Delta \vec{p}\]Impulse is equal to the change in momentum of an object. From this equation we see, that for a given change in momentum, \(\vec{F}_{net}\Delta t\) is fixed. Thus, if \(\vec{F}_{net}\) is reduced, \(\Delta t\) must be increased (i.e. a smaller resultant force must be applied for longer to bring about the same change in momentum). Alternatively if \(\Delta t\) is reduced (i.e. the resultant force is applied for a shorter period) then the resultant force must be increased to bring about the same change in momentum.
The graphs below show how the force acting on a body changes with time.
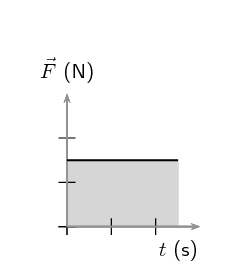
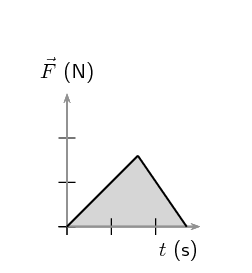
The area under the graph, shaded in, represents the impulse of the body.
A \(\text{150}\) \(\text{N}\) resultant force acts on a \(\text{300}\) \(\text{kg}\) trailer. Calculate how long it takes this force to change the trailer's velocity from \(\text{2}\) \(\text{m·s$^{-1}$}\) to \(\text{6}\) \(\text{m·s$^{-1}$}\) in the same direction. Assume that the forces acts to the right which is the direction of motion of the trailer.
The question explicitly gives
the trailer's mass as \(\text{300}\) \(\text{kg}\),
the trailer's initial velocity as \(\text{2}\) \(\text{m·s$^{-1}$}\) to the right,
the trailer's final velocity as \(\text{6}\) \(\text{m·s$^{-1}$}\) to the right, and
the resultant force acting on the object.
We are asked to calculate the time taken \(\Delta t\) to accelerate the trailer from the \(\text{2}\) to \(\text{6}\) \(\text{m·s$^{-1}$}\). From the Newton's second law,
\begin{align*} \vec{F}_{net}\Delta t& = \Delta \vec{p} \\ & = m\vec{v}_{f}-m\vec{v}_{i} \\ \Delta t& = \frac{m}{\vec{F}_{net}}\left(\vec{v}_{f}-\vec{v}_{i}\right). \end{align*}Thus we have everything we need to find \(\Delta t\)!
Choose right as the positive direction.
It takes \(\text{8}\) \(\text{s}\) for the force to change the object's velocity from \(\text{2}\) \(\text{m·s$^{-1}$}\) to the right to \(\text{6}\) \(\text{m·s$^{-1}$}\) to the right.
A cricket ball weighing \(\text{156}\) \(\text{g}\) is moving at \(\text{54}\) \(\text{km·hr$^{-1}$}\) towards a batsman. It is hit by the batsman back towards the bowler at \(\text{36}\) \(\text{km·hr$^{-1}$}\). Calculate
the ball's impulse, and
the average force exerted by the bat if the ball is in contact with the bat for \(\text{0,13}\) \(\text{s}\).
The question explicitly gives
the ball's mass,
the ball's initial velocity,
the ball's final velocity, and
the time of contact between bat and ball
We are asked to calculate the impulse:
\[\text{Impulse}=\Delta \vec{p}=\vec{F}_{\text{net}}\Delta t\]Since we do not have the force exerted by the bat on the ball ( \(\vec{F}_{\text{net}}\)), we have to calculate the impulse from the change in momentum of the ball. Now, since
\begin{align*} \Delta \vec{p}& = \vec{p}_{f}-\vec{p}_{i} \\ & = m\vec{v}_{f}-m\vec{v}_{i}, \end{align*}we need the ball's mass, initial velocity and final velocity, which we are given.
Firstly let us change units for the mass
\begin{align*} \text{1 000} \text{g}& = 1 \text{kg} \\ \text{So,} 1 \text{g}& = \frac{1}{\text{1 000}} \text{kg} \\ \therefore 156\times 1 \text{g}& = 156\times \frac{1}{\text{1 000}} \text{kg} \\ & = \text{0,156} \text{kg} \end{align*}Next we change units for the velocity
\begin{align*} 1 \text{km}·{\text{h}}^{-1}& = \frac{\text{1 000} \text{m}}{\text{3 600} \text{s}} \\ \therefore 54\times 1 \text{km}·{\text{h}}^{-1}& = 54\times \frac{\text{1 000} \text{m}}{\text{3 600} \text{s}} \\ & = 15 \text{m·s$^{-1}$} \end{align*}Similarly, \(\text{36}\) \(\text{km·hr$^{-1}$}\) = \(\text{10}\) \(\text{m·s$^{-1}$}\).
Let us choose the direction from the batsman to the bowler as the positive direction. Then the initial velocity of the ball is \(\vec{v}_{i}=-\text{15}\text{ m·s$^{-1}$}\), while the final velocity of the ball is \(\vec{v}_{f}=+\text{10}\text{ m·s$^{-1}$}\).
Now we calculate the change in momentum,
\begin{align*} \Delta \vec{p}& = \vec{p}_{f}-\vec{p}_{i} \\ & = m\vec{v}_{f}-m\vec{v}_{i} \\ & = m\left(\vec{v}_{f}-\vec{v}_{i}\right) \\ & = \left(\text{0,156}\right)\left(\left(+10 \right)-\left(-15 \right)\right) \\ & = \text{+3,9} \\ & = \text{3,9}\text{ kg·m·s$^{-1}$}~\text{in the direction from the batsman to the bowler} \end{align*}Finally since impulse is just the change in momentum of the ball,
\begin{align*} \text{Impulse}& = \Delta \vec{p} \\ & =\text{3,9}\text{ kg·m·s$^{-1}$}~\text{in the direction from the batsman to the bowler} \end{align*}We are given \(\Delta t\) and we have calculated the impulse of the ball.
\begin{align*} \vec{F}_{net}\Delta t& = \text{Impulse} \\ \vec{F}_{net}\left(\text{0,13}\right)& = \text{+3,9} \\ \vec{F}_{net}& = \frac{\text{+3,9}}{\text{0,13}} \\ & = +30 \\ & = \text{30}\text{ N}~\text{in the direction from the batsman to the bowler} \end{align*}Analyse the Force vs. time graph provided and answer the following questions:
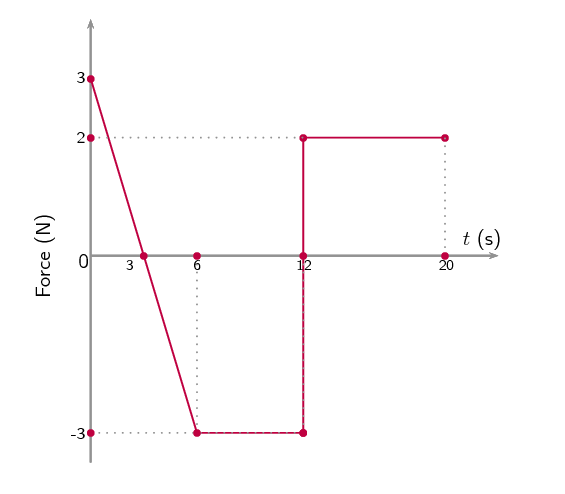
A graph of force versus time is provided. We are asked to determine both impulse and change in momentum from it.
We know that the area under the graph is the impulse and we can relate impulse to change in momentum with the impulse-momentum theorem.
We need to calculate the area under the graph for the various intervals to determine impulse and then work from there.
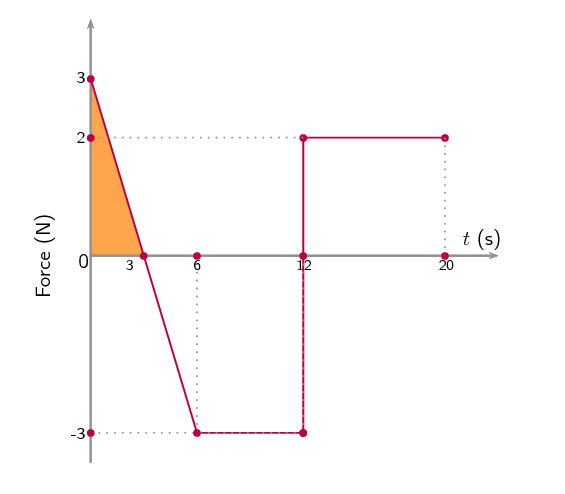
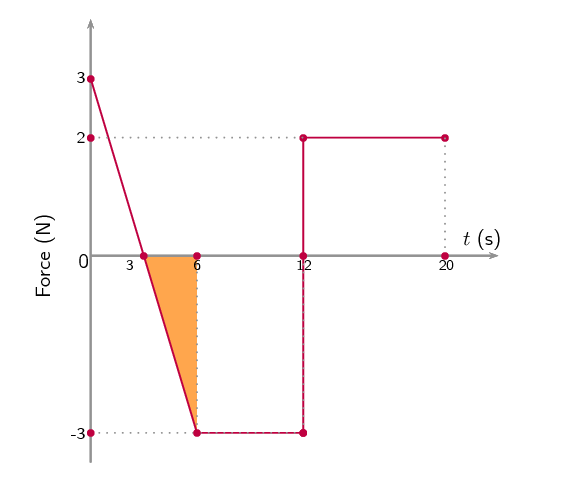
We need to calculate the area of the shaded portion under the graph. This is a triangle with a base of \(\text{3}\) \(\text{s}\) and a height of \(\text{3}\) \(\text{N}\) therefore:
\begin{align*} \text{Impulse} &= \frac{1}{2}bh \\ & = \frac{1}{2}(3)(3) \\ & = \text{4,5}\text{ N·s} \end{align*}The impulse is \(\text{4,5}\) \(\text{N·s}\) in the positive direction.
We need to calculate the area of the shaded portion under the graph. This is a triangle with a base of \(\text{3}\) \(\text{s}\) and a height of \(-\text{3}\) \(\text{N}\). Note that the force has a negative value so is pointing in the negative direction.
\begin{align*} \text{Impulse} &= \frac{1}{2}bh \\ & = \frac{1}{2}(3)(-3) \\ & = -\text{4,5}\text{ N·s} \end{align*}The impulse is \(\text{4,5}\) \(\text{N·s}\) in the negative direction.
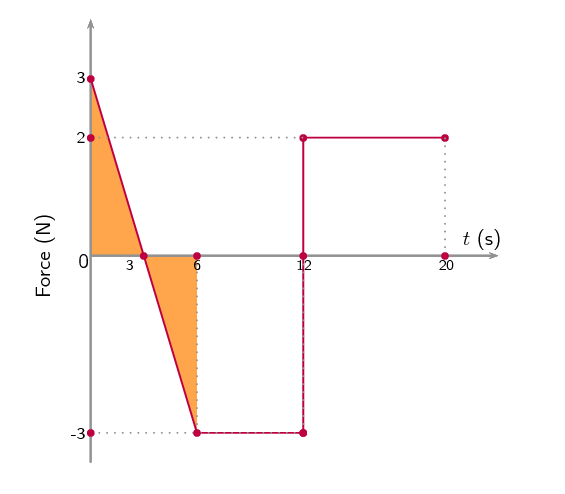
From the impulse-momentum theorem we know that that impulse is equal to the change in momentum. We have worked out the impulse for the two sub-intervals making up \(\text{0}\) \(\text{s}\) to \(\text{6}\) \(\text{s}\). We can sum them to find the impulse for the total interval:
\begin{align*} \text{impulse}_{0-6} & = \text{impulse}_{0-3} + \text{impulse}_{3-6} \\ &= (\text{4,5})+(-\text{4,5}) \\ &= \text{0}\text{ N·s} \end{align*}The positive impulse in the first 3 seconds is exactly opposite to the impulse in the second 3 second interval making the total impulse for the first 6 seconds zero:
\[\text{impulse}_{0-6} = \text{0}\text{ N·s}\]From the impulse-momentum theorem we know that:
\[\Delta \vec{p} = \text{impulse} = \text{0}\text{ N·s}\]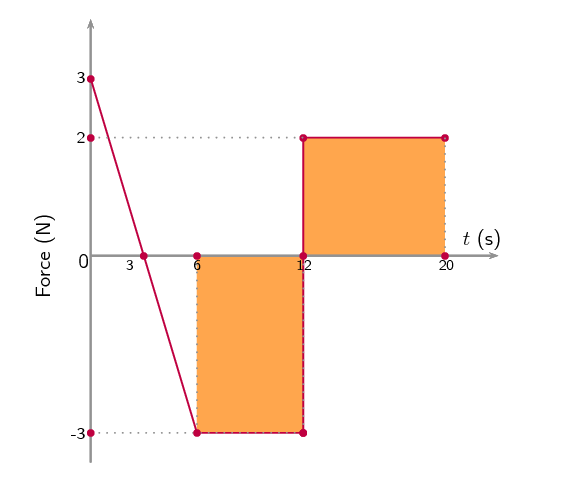
We need to calculate the area of the shaded portion under the graph. This is divided into two areas, \(\text{6}\) \(\text{s}\) to \(\text{12}\) \(\text{s}\) and \(\text{12}\) \(\text{s}\) to \(\text{20}\) \(\text{s}\), which we need to sum to get the total impulse.
\begin{align*} \text{Impulse}_{6-12} &= (6)(-3) \\ & = -\text{18}\text{ N·s} \end{align*}\begin{align*} \text{Impulse}_{12-20} &= (8)(2) \\ & = +\text{16}\text{ N·s} \end{align*}The total impulse is the sum of the two:
\begin{align*} \text{Impulse}_{6-20} &= \text{Impulse}_{6-12} + \text{Impulse}_{12-20} \\ &= (-18) + (16) \\ &= -\text{2}\text{ N·s} \end{align*}The impulse is \(\text{2}\) \(\text{N·s}\) in the negative direction.
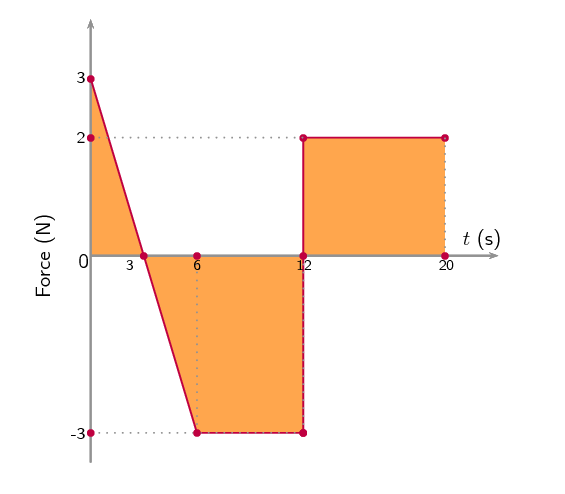
The impulse is \(\text{2}\) \(\text{N·s}\) in the negative direction.
A patrol car is moving on a straight horizontal road at a velocity of \(\text{10}\) \(\text{m·s$^{-1}$}\) east. At the same time a thief in a car ahead of him is driving at a velocity of \(\text{40}\) \(\text{m·s$^{-1}$}\) in the same direction.

\(v_{PG}\): velocity of the patrol car relative to the ground \(v_{TG}\): velocity of the thief's car relative to the ground
Questions 1 and 2 from the original version in 2011 Paper 1 are no longer part of the curriculum.
While travelling at \(\text{40}\) \(\text{m·s$^{-1}$}\), the thief's car of mass \(\text{1 000}\) \(\text{kg}\), collides head-on with a truck of mass \(\text{5 000}\) \(\text{kg}\) moving at \(\text{20}\) \(\text{m·s$^{-1}$}\). After the collision, the car and the truck move together. Ignore the effects of friction.

State the law of conservation of linear momentum in words.
(2 marks)
Calculate the velocity of the thief's car immediately after the collision.
(6 marks)
Research has shown that forces greater than 85 000 N during collisions may cause fatal injuries. The collision described above lasts for \(\text{0,5}\) \(\text{s}\).
Determine, by means of a calculation, whether the collision above could result in a fatal injury.
(5 marks)
[TOTAL: 13 marks]
Question 1
The total (linear) momentum remains constant (OR is conserved OR does not change) in an isolated (OR in a closed system OR in the absence of external forces).
(2 marks)
Question 2
Option 1:
Taking to the right as positive
\begin{align*} \sum p_{\text{before}} & = \sum p_{\text{after}} \\ (\text{1 000})(\text{40})+(\text{5 000})(-\text{20}) & = (\text{1 000}+\text{5 000})v_f \\ v_f & = -\text{10 m}\cdot\text{s}^{-1}\\ & = \text{10 m}\cdot\text{s}^{-1} \quad \text{left OR west} \end{align*}Option 2:
Taking to the right as positive
\begin{align*} \Delta p_{\text{car}}& = - \Delta p_{\text{truck}} \\ m_{\text{car}}(v_f -v_{i,\text{car}}) & = - m_{\text{truck}}(v_f -v_{i,\text{truck}}) \\ (\text{1 000})(v_f-(\text{40})) & = -(\text{5 000})(v_f -(-\text{20})) \\ \text{6 000}v_f & = -\text{60 000} \\ \therefore v_f & = -\text{10 m}\cdot\text{s}^{-1} \\ \therefore v_f & = \text{10 m}\cdot\text{s}^{-1} \quad \text{left OR west} \end{align*}(6 marks)
Question 3
Option 1:
Force on the car: (Taking to the right as positive)
\begin{align*} F_{\text{net}} \Delta t & = \Delta p = mv_f - mv_i \\ F_{\text{net}} (\text{0,5}) & = (\text{1 000})(-\text{10}-\text{40}) \\ \therefore F_{\text{net}} & = -10^5\text{ N} \\ & \text{OR} \\ \therefore F_{\text{net}} & = 10^5\text{ N} \quad (\text{100 000 N})\\ \therefore F_{\text{net}} & > \text{85 000 N} \end{align*}Yes, the collision is fatal.
OR
Force on the car: (Taking to the left as positive)
\begin{align*} F_{\text{net}} \Delta t & = \Delta p = mv_f - mv_i \\ F_{\text{net}} (\text{0,5}) & = (\text{1 000})(\text{10}-(-40)) \\ \therefore F_{\text{net}} & = 10^5\text{ N} \quad (\text{100 000 N})\\ \therefore F_{\text{net}} & > \text{85 000 N} \end{align*}Yes, the collision is fatal.
Option 2:
Force on the truck: (Taking to the right as positive)
\begin{align*} F_{\text{net}} \Delta t & = \Delta p = mv_f - mv_i \\ F_{\text{net}} (\text{0,5}) & = (\text{5 000})(-10-(-20)) \\ \therefore F_{\text{net}} & = 10^5\text{ N} \quad (\text{100 000 N})\\ \therefore F_{\text{net}} & > \text{85 000 N} \end{align*}Yes, the collision is fatal.
OR
Force on the truck: (Taking to the left as positive)
\begin{align*} F_{\text{net}} \Delta t & = \Delta p = mv_f - mv_i \\ F_{\text{net}} (\text{0,5}) & = (\text{1 000})(\text{10}-\text{20}) \\ \therefore F_{\text{net}} & = -10^5\text{ N} \\ & \text{OR} \\ \therefore F_{\text{net}} & = 10^5\text{ N} \quad (\text{100 000 N})\\ \therefore F_{\text{net}} & > \text{85 000 N} \end{align*}Yes, the collision is fatal.
Option 3:
Force on the car: (Taking to the right as positive)
\begin{align*} v_f & = v_i + a \Delta t \\ -10 & = \text{40} + a (\text{0,5})\\ \therefore a & = -\text{100 m}\cdot\text{s}^{-2}\\ F_{\text{net}} & = ma \\ & = (\text{1 000})(-100) \\ F_{\text{net}} & = -10^5\text{ N} \quad (-\text{100 000 N})\\ F_{\text{net}} & = 10^5\text{ N} \quad (\text{100 000 N})\\ \therefore F_{\text{net}} & > \text{85 000 N} \end{align*}Yes, the collision is fatal.
OR
Force on the car: (Taking to the left as positive)
\begin{align*} v_f & = v_i + a \Delta t \\ \text{10} & = -40 + a (\text{0,5})\\ \therefore a & = \text{100 m}\cdot\text{s}^{-2}\\ F_{\text{net}} & = ma \\ & = (\text{1 000})(\text{100}) \\ F_{\text{net}} & = 10^5\text{ N} \quad (\text{100 000 N})\\ \therefore F_{\text{net}} & > \text{85 000 N} \end{align*}Yes, the collision is fatal.
Option 4:
Force on the truck: (Taking to the right as positive)
\begin{align*} v_f & = v_i + a \Delta t \\ -10 & = -20 + a (\text{0,5})\\ \therefore a & = \text{20 m}\cdot\text{s}^{-2}\\ F_{\text{net}} & = ma \\ & = (\text{5 000})(\text{20}) \\ F_{\text{net}} & = 10^5\text{ N} \quad (\text{100 000 N})\\ \therefore F_{\text{net}} & > \text{85 000 N} \end{align*}Yes, the collision is fatal.
OR
Force on the truck: (Taking to the left as positive)
\begin{align*} v_f & = v_i + a \Delta t \\ \text{10} & = \text{20} + a (\text{0,5})\\ \therefore a & = -\text{20 m}\cdot\text{s}^{-2}\\ F_{\text{net}} & = ma \\ & = (\text{5 000})(-20) \\ F_{\text{net}} & = -10^5\text{ N} \quad (-\text{100 000 N})\\ F_{\text{net}} & = 10^5\text{ N} \quad (\text{100 000 N})\\ \therefore F_{\text{net}} & > \text{85 000 N} \end{align*}Yes, the collision is fatal.
(5 marks)
[TOTAL: 13 marks]
Which one of the following is not a unit of impulse?
\(\text{N·s}\)
\(\text{kg·m·s$^{-1}$}\)
\(\text{J·m·s$^{-1}$}\)
\(\text{J·m$^{-1}$·s}\)
\(\text{J·m·s$^{-1}$}\)
A toy car of mass \(\text{1}\) \(\text{kg}\) moves eastwards with a speed of \(\text{2}\) \(\text{m·s$^{-1}$}\). It collides head-on with a toy train. The train has a mass of \(\text{2}\) \(\text{kg}\) and is moving at a speed of \(\text{1,5}\) \(\text{m·s$^{-1}$}\) westwards. The car rebounds (bounces back) at \(\text{3,4}\) \(\text{m·s$^{-1}$}\) and the train rebounds at \(\text{1,2}\) \(\text{m·s$^{-1}$}\).
Calculate the change in momentum for each toy.
Take Eastwards as positive.
For the car:
\begin{align*} Δp & = mv_{f} - mv_{i} \\ & = (1)(-\text{3,4}) - (1)(\text{2}) \\ & = \text{5,4}\text{ kg·m·s$^{-1}$} \end{align*}For the train:
\begin{align*} Δp & = mv_{f} - mv_{i} \\ & = (2)(\text{1,2}) - (2)(-\text{1,5}) \\ & = \text{5,4}\text{ kg·m·s$^{-1}$} \end{align*}Determine the impulse for each toy.
For the car:
\[\text{Impulse} = \text{5,4}\text{ kg·m·s$^{-1}$}\]For the train:
\[\text{Impulse} = \text{5,4}\text{ kg·m·s$^{-1}$}\]Determine the duration of the collision if the magnitude of the force exerted by each toy is \(\text{8}\) \(\text{N}\).
A bullet of mass \(\text{20}\) \(\text{g}\) strikes a target at \(\text{300}\) \(\text{m·s$^{-1}$}\) and exits at \(\text{200}\) \(\text{m·s$^{-1}$}\). The tip of the bullet takes \(\text{0,0001}\) \(\text{s}\) to pass through the target. Determine:
the change of momentum of the bullet.
the impulse of the bullet.
the magnitude of the force experienced by the bullet.
A bullet of mass \(\text{20}\) \(\text{g}\) strikes a target at \(\text{300}\) \(\text{m·s$^{-1}$}\). Determine under which circumstances the bullet experiences the greatest change in momentum, and hence impulse:
When the bullet exits the target at \(\text{200}\) \(\text{m·s$^{-1}$}\).
If the bullet leaves the target its total momentum is: \(\Delta\vec{p} = (\text{0,02})(\text{200}) - (\text{0,02})(\text{300}) = -\text{2}\text{ kg·m·s$^{-1}$}\)
When the bullet stops in the target.
If the bullet remains in the target its total momentum is: \(\Delta\vec{p} = (\text{0,02})(\text{0}) - (\text{0,02})(\text{300}) = -\text{6}\text{ kg·m·s$^{-1}$}\)
When the bullet rebounds at \(\text{200}\) \(\text{m·s$^{-1}$}\).
If the bullet rebounds from the target its total momentum is: \(\Delta\vec{p} = (\text{0,02})(-\text{200}) - (\text{0,02})(\text{300}) = -\text{10}\text{ kg·m·s$^{-1}$}\)
So the bullet experiences the greatest change in momentum and hence impulse, when it rebounds from the target.
A ball with a mass of \(\text{200}\) \(\text{g}\) strikes a wall perpendicularly at a velocity of \(\text{12}\) \(\text{m·s$^{-1}$}\) and rebounds at a velocity of \(\text{9}\) \(\text{m·s$^{-1}$}\).
Calculate the change in the momentum of the ball.
Take the direction towards the wall as positive.
\begin{align*} \Delta p & = mv_i - mv_f \\ & = (\text{200})(\text{12}) - (\text{200})(-\text{9}) \\ & = -\text{4,2}\text{ kg·m·s$^{-1}$} \end{align*}What is the impulse of the wall on the ball?
\(-\text{4,2}\) \(\text{kg·m·s$^{-1}$}\)
Calculate the magnitude of the force exerted by the wall on the ball if the collision takes \(\text{0,02}\) \(\text{s}\).
If the ball in the previous problem is replaced with a piece of clay of \(\text{200}\) \(\text{g}\) which is thrown against the wall with the same velocity, but then sticks to the wall, calculate:
The impulse of the clay on the wall.
Calculate the change in momentum of the clay:
\begin{align*} \Delta\vec{p} & = m\vec{v}_f - m\vec{v}_i \\ & = (\text{0,2})(0) - (\text{0,2})(12) \\ & = - (\text{0,2})(12) \\ & = - \text{2,4} \\ & = \text{2,4}\text{ kg·m·s$^{-1}$}~\text{away from the wall} \end{align*}The change in momentum of the wall is equal and opposite to the change in momentum of the clay, therefore:
\(\Delta\vec{p}_{wall} = \text{2,4}\text{ kg·m·s$^{-1}$}~\text{into the wall}\)The impulse on the wall is equivalent to its change in momentum, therefore
\(\text{impulse} = \text{2,4}\text{ kg·m·s$^{-1}$}~\text{into the wall}\)
\(\text{impulse} = \text{2,4}\text{ kg·m·s$^{-1}$}~\text{into the wall}\)The force exerted by the clay on the wall if it is in contact with the wall for \(\text{0,5}\) \(\text{s}\) before it comes to rest.
\begin{align*} F& = \frac{\Delta \vec{p}}{\Delta t} \\ F& = \frac{\text{2,4}}{\text{0,5}} \\ & = \text{4,8}\text{ N}~\text{into the wall} \end{align*}
|
Previous
2.4 Conservation of momentum
|
Table of Contents |
Next
2.6 Physics in action: Impulse
|