10.5 Extension: Wheatstone bridge [Not examinable]

10.5 Extension: Wheatstone bridge [Not examinable] (ESCPZ)

Using what we know about parallel networks of resistors we can devise another method of finding an unknown resistance, the Wheatstone bridge. A Wheatstone bridge is a measuring instrument that is used to measure an unknown electrical resistance by balancing two legs of a bridge circuit, one leg of which includes the unknown component. Its operation is similar to the original potentiometer except that in potentiometer circuits the meter used is a sensitive galvanometer.

Despite the fact that we have given this circuit a special name, it is just a circuit containing a parallel configuration of four resistors. This is not actually a new concept, this particular configuration is just particularly useful.

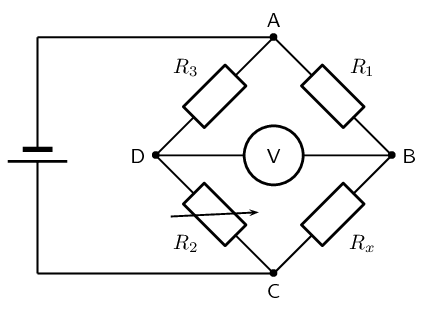
In the circuit of the Wheatstone bridge, \({R}_{x}\) is the unknown resistance. \({R}_{\text{1}}\), \({R}_{\text{2}}\) and \({R}_{\text{3}}\) are resistors of known resistance and the resistance of \({R}_{\text{2}}\) is adjustable. If the ratio of \({R}_{\text{2}}\): \({R}_{\text{1}}\) is equal to the ratio of \({R}_{x}\): \({R}_{\text{3}}\), then the potential difference between the two midpoints will be zero and no current will flow between the midpoints. In order to determine the unknown resistance, \({R}_{\text{2}}\) is varied until this condition is reached. That is when the voltmeter reads 0 V.

Worked example 11: Wheatstone bridge

What is the resistance of the unknown resistor \({R}_{x}\) in the diagram below if \({R}_{\text{1}} = \text{4}\) \(\text{Ω}\) \({R}_{\text{2}} =\text{8}\) \(\text{Ω}\) and \({R}_{\text{3}} =\text{6}\) \(\text{Ω}\).

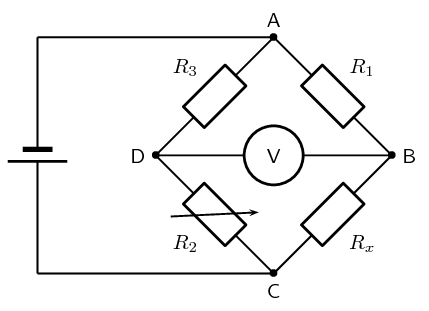
Determine how to approach the problem

The arrangement is a Wheatstone bridge. So we use the equation:

\begin{align*} {R}_{x}:{R}_{\text{3}}& = {R}_{\text{2}}:{R}_{\text{1}} \end{align*}

Solve the problem

\begin{align*} {R}_{x}:{R}_{\text{3}}& = {R}_{\text{2}}:{R}_{\text{1}}\\ {R}_{x}:6& = 8:4\\ {R}_{x}& = 12\phantom{\rule{3.33333pt}{0ex}}\Omega \phantom{\rule{4pt}{0ex}} \end{align*}

Write the final answer

The resistance of the unknown resistor is \(\text{12}\) \(\text{Ω}\).