19.3 Amount of substance | Quantitative aspects of chemical change

19.3 Amount of substance (ESAGC)

Molar Volumes of Gases (ESAGD)

Molar volume of gases: One mole of gas occupies $\text{22,4}$ $\text{dm$^{3}$}$ at standard temperature and pressure.

This applies to any gas that is at standard temperature and pressure. In grade 11 you will learn more about this and the gas laws.

Standard temperature and pressure (S.T.P.) is defined as a temperature of $\text{273,15}$ $\text{K}$ and a pressure of $\text{0,986}$ $\text{atm}$.

For example, $\text{2}$ $\text{mol}$ of $\text{H}_{2}$ gas will occupy a volume of $\text{44,8}$ $\text{dm$^{3}$}$ at standard temperature and pressure (S.T.P.). and $\text{67,2}$ $\text{dm$^{3}$}$ of ammonia gas ($\text{NH}_{3}$) contains $\text{3}$ $\text{mol}$ of ammonia.

Molar concentrations of liquids (ESAGE)

A typical solution is made by dissolving some solid substance in a liquid. The amount of substance that is dissolved in a given volume of liquid is known as the concentration of the liquid. Mathematically, concentration ($c$) is defined as moles of solute ($n$) per unit volume ($V$) of solution.

\[c = \frac{n}{V}\]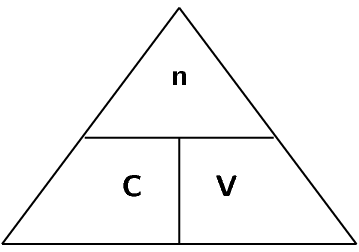

For this equation, the units for volume are $\text{dm$^{3}$}$ (which is equal to litres). Therefore, the unit of concentration is $\text{mol·dm$^{-3}$}$.

Concentration: Concentration is a measure of the amount of solute that is dissolved in a given volume of liquid. It is measured in $\text{mol·dm$^{-3}$}$.

temp text

Worked example 12: Concentration calculations 1

If $\text{3,5}$ $\text{g}$ of sodium hydroxide ($\text{NaOH}$) is dissolved in $\text{2,5}$ $\text{dm$^{3}$}$ of water, what is the concentration of the solution in $\text{mol·dm$^{-3}$}$?

Find the number of moles of sodium hydroxide

\begin{align*} n & = \frac{m}{M} \\ & = \frac{\text{3,5}\text{ g}}{\text{40,01}\text{ g·mol$^{-1}$}} \\ & = \text{0,0875}\text{ mol} \end{align*}

Calculate the concentration

\begin{align*} c & = \frac{n}{V} \\ & = \frac{\text{0,0875}\text{ mol}}{\text{2,5}\text{ dm$^{3}$}} \\ & = \text{0,035}\text{ mol·dm$^{-3}$} \end{align*}

The concentration of the solution is $\text{0,035}$ $\text{mol·dm$^{-3}$}$.

Worked example 13: Concentration calculations 2

You have a $\text{1}$ $\text{dm$^{3}$}$ container in which to prepare a solution of potassium permanganate ($\text{KMnO}_{4}$). What mass of $\text{KMnO}_{4}$ is needed to make a solution with a concentration of $\text{0,2}$ $\text{mol·dm$^{-3}$}$?

Calculate the number of moles

$c = \dfrac{n}{V}$ therefore:

\begin{align*} n & = c \times V \\ & = \text{0,2}\text{ mol·dm$^{-3}$} \times \text{1}\text{ dm$^{3}$} \\ & = \text{0,2}\text{ mol} \end{align*}

Find the mass

\begin{align*} m & = nM \\ & = (\text{0,2}\text{ mol})(\text{158}\text{ g·mol$^{-1}$}) \\ & = \text{31,6}\text{ g} \end{align*}

The mass of $\text{KMnO}_{4}$ that is needed is $\text{31,6}$ $\text{g}$.

Worked example 14: Concentration calculations 3

How much sodium chloride (in g) will one need to prepare $\text{500}$ $\text{cm$^{3}$}$ of solution with a concentration of $\text{0,01}$ $\text{mol·dm$^{-3}$}$?

Convert the given volume to the correct units

\[V = \text{500}\text{ cm$^{3}$} \times \frac{\text{1}\text{ dm$^{3}$}}{\text{1 000}\text{ cm$^{3}$}} = \text{0,5}\text{ dm$^{3}$}\]

Find the number of moles

\begin{align*} n & = c \times V \\ & = \text{0,01}\text{ mol·dm$^{-3}$} \times \text{0,5}\text{ dm$^{3}$} \\ & = \text{0,005}\text{ mol} \end{align*}

Find the mass

\begin{align*} m & = nM \\ & = (\text{0,005}\text{ mol})(\text{58,45}\text{ g·mol$^{-1}$}) \\ & = \text{0,29225}\text{ g} \\ & \approx \text{0,29}\text{ g} \end{align*}

The mass of sodium chloride needed is $\text{0,29}$ $\text{g}$

Concentration of solutions

Textbook Exercise 19.6

$\text{5,95}$ $\text{g}$ of potassium bromide was dissolved in $\text{400}$ $\text{dm$^{3}$}$ of water. Calculate its concentration.

Solution not yet available

$\text{100}$ $\text{g}$ of sodium chloride ($\text{NaCl}$) is dissolved in $\text{450}$ $\text{cm$^{3}$}$ of water.

How many moles of $\text{NaCl}$ are present in solution?
What is the volume of water (in $\text{dm$^{3}$}$)?
Calculate the concentration of the solution.

Solution not yet available

What is the molarity of the solution formed by dissolving $\text{80}$ $\text{g}$ of sodium hydroxide ($\text{NaOH}$) in $\text{500}$ $\text{cm$^{3}$}$ of water?

Solution not yet available

What mass (g) of hydrogen chloride ($\text{HCl}$) is needed to make up $\text{1 000}$ $\text{cm$^{3}$}$ of a solution of concentration $\text{1}$ $\text{mol·dm$^{-3}$}$?

Solution not yet available

How many moles of $\text{H}_{2}\text{SO}_{4}$ are there in $\text{250}$ $\text{cm$^{3}$}$ of a $\text{0,8}$ $\text{mol·dm$^{-3}$}$ sulphuric acid solution? What mass of acid is in this solution?

Solution not yet available

19.2 Composition

Table of Contents

19.4 Stoichiometric calculations