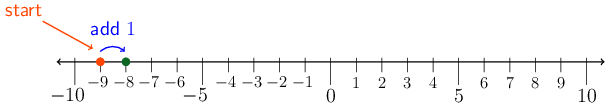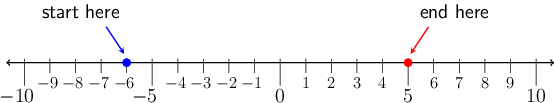|
Previous
Introduction
|
Next
Counting, ordering and comparing integers
|
When calculating with small integers, you can use the number line:
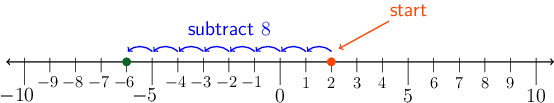
Evaluate the following without using a calculator:
\[2 - 8\]To subtract \(8\) from \(2\), we must start at \(2\) on the number line and count \(8\) to the left.
Evaluate the following without using a calculator:
\[- 9 + 1\]To add \(1\) to \(−9\), we must start at \(−9\) on the number line and count \(1\) to the right.
The following statement is true if the unknown number is \(5\):
\(15\) − (a certain number) = \(10\)
Another number will make the following statement true:
\(15\) + (a certain number) = \(10\)
If you add this number, it should have the same effect as to subtract \(5\).
That number is “negative \(5\)”. It has the property that if you add it to another number, the effect will be the same as when you subtract its positive opposite:
\(15 + ( - 5)\) is equal to \(15 - 5\).
Adding a negative number has the same effect as subtracting a positive number.
For example: \(20 + ( - 15) = 20 - 15 = 5\).
Calculate the following:
Gcina is working with the number line below. She needs to start at the blue dot at \(−6\) and end at the red dot at \(5\).
What must Gcina do to get from the starting point at \(−6\) to the end point at \(5\)? Write the number sentence to match this problem.
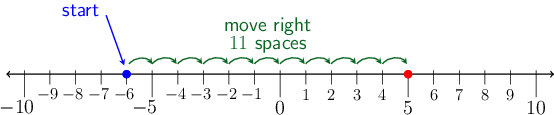
Moving from the blue dot (\(−6\)) to the red dot (\(5\)) looks like this:
Since the arrows are moving to the right, this is addition.
Adding positive numbers means going to the right, while subtracting positive numbers means going to the left.
You can count the number of arrows. This tells us how many units to add to the blue dot to get to the red dot. In this case there are \(11\) arrows.
The correct final answer is that you need to add \(11\) to \(−6\).
The picture tells us how to do this: if we start at \(−6\) and then add \(11\) spaces, we will end up at \(5\).
The number sentence is:
\[-6 + 11 = 5\]When multiplying with integers, the following rules apply:
Calculate the following without using a calculator: \(- 3 \times ( - 4)\).
When you multiply a negative number and a negative number, you get a positive number. So, the answer will be positive.
Calculate the following, without using a calculator: \(- 15 \times 2\).
When you multiply a negative number and a positive number, you get a negative number. So, the answer will be negative.
Calculate the following, without using a calculator: \(25 \times ( - 10)\).
When you multiply a positive number and a negative number, you get a negative number. So, the answer will be negative.
When dividing with integers, the following rules apply:
Calculate the following without using a calculator: \(- 30 \div ( - 5)\).
When you divide a negative number and a negative number, you get a positive number. So, the answer will be positive.
Calculate the following without using a calculator: \(- 15 \div 3\).
When you divide a negative number and a positive number, you get a negative number. So, the answer will be negative.
Calculate the following without using a calculator: \(25 \div ( - 5)\).
When you divide a positive number and a negative number, you get a negative number. So, the answer will be negative.
When it comes to the sign of product or quotient, the rules for multiplication and division with integers are the same.
When you multiply or divide with negative numbers, there are only two rules you must remember:
- If the numbers have the same signs, then the answer is positive.
- If the numbers have opposite signs, then the answer is negative.
These rules also apply when simplifying fractions. For example, \(\frac{- 49}{7} = - 7\) and \(\frac{- 14}{- 2} = 7\).
When finding the square of an integer, we multiply an integer by itself, keeping its sign in mind. For example, \(12^{2} = 12 \times 12 = 144\) and \(( - 12)^{2} = ( - 12) \times ( - 12) = + 144\).
The square of an integer is always a positive number.
When finding a cube of an integer, we multiply an integer by itself, and by itself again. For example, \(2^{3} = 2 \times 2 \times 2 = 8\) and \(( - 2)^{3} = ( - 2) \times ( - 2) \times ( - 2) = - 8\).
The cube of an integer is positive if the integer is positive, and negative if the integer is negative.
Always pay attention to where the minus sign is placed: \(- 5^{2} \neq ( - 5)^{2}\).
\[- 5^{2} = - (5 \times 5) = - 25\] \[( - 5)^{2} = ( - 5) \times ( - 5) = 25\]The minus sign of a negative integer raised to a power will always be inside the brackets.
Now have a look at the square roots of integers: \(10^{2}\) is \(100\) and \(( - 10)^{2}\) is also \(100\).
Both \(10\) and (\(−10\)) are called square roots of \(100\). \(10\) is the positive square root of \(100\) and (\(−10\)) is the negative square root of \(100\):
\(\sqrt{100} = 10\) and \(\sqrt{100} = - 10\)
Cube roots can also be positive or negative numbers. For example,
\(3^{3}\) is \(27\) and \(( - 5)^{3}\) is \(−125\).
\(3\) is called the cube root of \(27\), because \(3^{3} = 27\). So, \(\sqrt[3]{27} = 3\).
\(( - 5)\) is called the cube root of \(−125\) because \(( - 5)^{3} = - 125\). So, \(\sqrt[3]{- 125} = - 5\).
It is possible to take a cube root of a negative integer, but it is not possible to take a square root of a negative integer.
\(\sqrt[3]{- 8} = - 2\) but \(\sqrt{- 16}\) is undefined
Calculate the following without using a calculator:
\[\sqrt[3]{9 + ( - 17)}\]So,
\[\sqrt[3]{9 + ( - 17)} = \sqrt[3]{- 8} = - 2\]Calculate the following without using a calculator:
\[8 - \sqrt[3]{- 64}\]When we take the cube root of a number, we are looking for a number that, when multiplied by itself, and by itself again, gives the number within the cube root.
\(\sqrt[3]{- 64} = - 4\) because \(( - 4) \times ( - 4) \times ( - 4) = - 64\)
Remember that, in any numeric or algebraic sentence, the terms are separated by addition and subtraction symbols, and joined into one by multiplication symbols, division symbols, and brackets. To simplify or evaluate an expression, we must:
To simplify a term, we must first simplify any parts within brackets. Then we must apply the exponents. If there are no brackets, then the exponent only applies to the base immediately before it, and we must simplify the power first.
Simplify and evaluate: \(- 3 + 2 \times 3^{2}\).
There are two terms in this expression: \(( - 3)\) and \((2 \times 3^{2})\).
The first term doesn’t need to be simplified. The second term must be simplified.
Calculate the following without using a calculator:
\[6 + (6 - 9) \div 3\]This expression has two terms: \(6\) and \((6 - 9) \div 3\).
We must simplify the second term, and then add from left to right.
The second term has brackets, so we must simplify the expression within the brackets first.
\[(6 - 9) \div 3 = ( - 3) \div 3 = - 1\]Calculate the following without using a calculator:
\[25 \div ( - 5) + 28 \div ( - 2)\]This expression has two terms: \(25 \div ( - 5)\) and \(28 \div ( - 2)\).
We must simplify each term before adding from left to right.
\(25 \div ( - 5) = - 5\) and \(28 \div ( - 2) = - 14\)
\(25 \div ( - 5) + 28 \div ( - 2)\) \(= - 5 + ( - 14)\) \(= - 5 - 14\) \(= - 19\)
|
Previous
Introduction
|
Table of Contents |
Next
Counting, ordering and comparing integers
|
