Classifying quadrilaterals

11.3 Classifying quadrilaterals

Properties of quadrilaterals

A quadrilateral is a four-sided polygon. A quadrilateral has four straight sides and four vertices. Different quadrilaterals have different angle and side relationships. The figure below shows five different types of quadrilaterals:

• A: Square
• B: Rectangle
• C: Parallelogram
• D: Rhombus
• E: Trapezium

quadrilateral

a four-sided polygon

A quadrilateral can be divided into two triangles by drawing a diagonal from one vertex to the opposite vertex. As a result, we can deduce that the sum of the interior angles of a quadrilateral is equal to twice the sum of the interior angles of a triangle:

Parallelograms

A parallelogram is a quadrilateral with both pairs of opposite sides equal and parallel.

The diagram shows parallelogram \(ABCD\):

• Opposite sides are equal: \(AB = DC\) and \(BC = AD\).
• Opposite sides are parallel: \(AB \parallel DC\) and \(BC \parallel AD\).

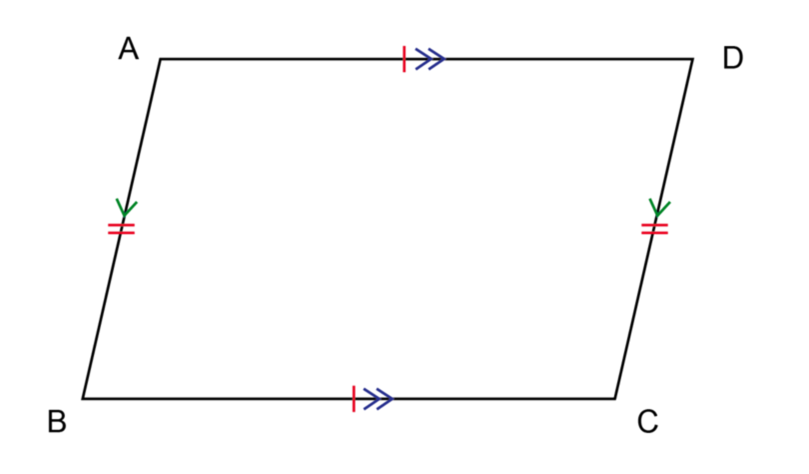
A parallelogram has four interior angles. A special property of the interior angles of parallelogram is that the interior opposite angles are equal. A parallelogram has two pairs of opposite angles that are equal.

The diagram shows parallelogram \(PQRS\) with opposite angles equal: \(\hat{P} = \hat{R}\) and \(\hat{Q} = \hat{S}\).

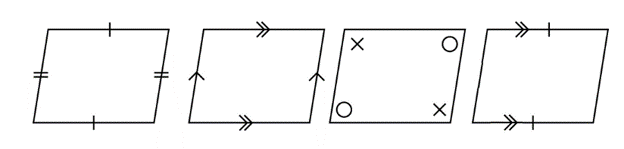
A quadrilateral can be classified as a parallelogram if any of the following properties are true:

• Both pairs of opposite sides are parallel.
• Both pairs of opposite angles are equal.
• One pair of opposite sides is equal and parallel.

Worked Example 11.7: Calculating angles and sides of a parallelogram

\(QRST\) is a parallelogram with \(\hat{Q} = 54^{\circ}\), \(\hat{R} = 126^{\circ}\), \(QT = 9 \text{ units}\), \(RS = 9 \text{ units}\) and \(TS = 5 \text{ units}\). Find the values of \(w\), \(x\) and \(y\).

Use the properties of a parallelogram to find the unknown values.

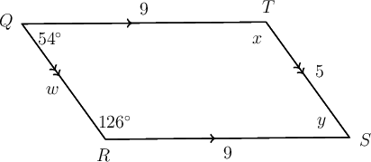
In parallelogram \(QRST\):

\[\begin{align} \hat{Q} &= \hat{S}\ (\text{opp. } \angle\text{s equal}) \\ \hat{Q} &= y \\ &= 54^{\circ} \end{align}\] \[\begin{align} \hat{R} &= \hat{T}\ (\text{opp. } \angle\text{s equal}) \\ \hat{R} &= x \\ &= 126^{\circ} \end{align}\] \[\begin{align} QR &= TS\ (\text{opp. sides equal}) \\ QR &= w \\ &= 5 \text{ units} \end{align}\]

Write the final answer.

In parallelogram \(QRST\), \(y = 54^{\circ}\), \(x = 126^{\circ}\) and \(w = 5 \text{ units}\).

Rectangles

A rectangle is quadrilateral with opposites equal and parallel and interior angles equal to \(90^{\circ}\).

rectangle

a quadrilateral with opposites equal and parallel and interior angles equal to \(90^{\circ}\)

The diagonal of a rectangle divides the rectangle into two right-angled triangles. We can use the theorem of Pythagoras to calculate the length of unknown sides of these triangles. You will learn more about this theorem in Chapter 15.

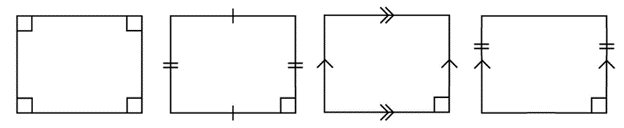
A quadrilateral can be classified as a rectangle if any of the following properties are true:

• All interior angles are equal to \(90^{\circ}\).
• Both pairs of opposite sides are equal and one interior angle is \(90^{\circ}\).
• Both pairs of opposite sides are parallel and one interior angle is \(90^{\circ}\).
• One pair of opposite sides is equal and parallel and one interior angle is \(90^{\circ}\).

Worked Example 11.8: Calculating angles and sides of a rectangle

\(MNPQ\) is a rectangle with \(\hat{N} = 90^{\circ}\) and \(M\hat{P}N = 59^{\circ}\).

Find the values of \(a\), \(b\), \(c\) and \(d\).

Use the properties of a rectangle to find the unknown angles.

In rectangle \(MNPQ\):

\[\begin{align} \hat{Q} &= \hat{N} = 90^{\circ}\ (\text{opp. } \angle\text{s equal}) \\ \hat{Q} &= a = 90^{\circ} \end{align}\]

In rectangle \(MNPQ\):

\[\begin{align} \hat{M} &= \hat{P} = 90^{\circ}\ (\text{opp. } \angle\text{s equal}) \\ b + 59^{\circ} &= 90^{\circ}\ (\text{right angle of rectangle}) \\ b &= 90^{\circ} − 59^{\circ} \\ b &= 31^{\circ} \end{align}\]

In \(\triangle MNP\):

\[\begin{align} d + 90^{\circ} + 59^{\circ} &= 180^{\circ} (\angle \text{ sum of } \triangle) \\ d &= 180^{\circ} − 149^{\circ} \\ d &= 31^{\circ} \end{align}\]

In rectangle \(MNPQ\):

\[\begin{align} c + d &= 90^{\circ}\ (\text{opp. } \angle\text{s equal}) \\ c &= 90^{\circ} − 31^{\circ} \\ c &= 59^{\circ} \end{align}\]

Write the final answer.

In rectangle \(MNPQ\), \(a = 90^{\circ}\), \(b = 31^{\circ}\), \(c = 59^{\circ}\) and \(d = 31^{\circ}\).

Squares

A square is parallelogram with all sides equal and interior angles equal to \(90^{\circ}\).

A diagonal divides the square into two right-angled isosceles triangles. We can use the theorem of Pythagoras to calculate the length of unknown sides of these triangles.

A quadrilateral can be classified as a square if any of the following properties are true:

• All sides are equal and one interior angle is \(90^{\circ}\).
• One pair of adjacent sides is equal and all interior angles are \(90^{\circ}\).

Worked Example 11.9: Calculating angles and sides of a square

\(ABCD\) is a square with \(\hat{D} = 3t\), \(AB = s\) and \(AD = 7 \text{ cm}\).

Find the values of \(s\) and \(t\).

Use the properties of a square to find the unknown values.

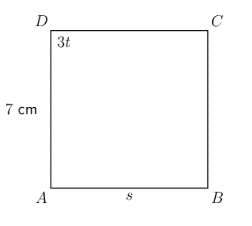
In square \(ABCD\):

\[\begin{align} \hat{D} &= 3t = 90^{\circ}\ (\text{right angle of square}) \\ 3t &= 90^{\circ} \\ \frac{3t}{3} &= \frac{90^{\circ}}{3} \\ t &= 30^{\circ} \end{align}\]

In square \(ABCD\):

\[\begin{align} AD &= AB = s\ (\text{equal sides of square}) \\ AD &= 7 \text{ cm} \\ \therefore AB &= s = 7 \text{ cm} \end{align}\]

Write the final answer.

In square \(ABCD\), \(t = 30^{\circ}\) and \(s = 7 \text{ cm}\)

Rhombus

A rhombus is a quadrilateral with both pairs of opposite sides parallel and all sides equal.

rhombus

a quadrilateral with both pairs of opposite sides parallel and all sides equal

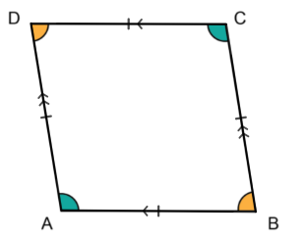
A rhombus has two pairs of opposite angles that are equal. A diagonal divides the rhombus into two isosceles triangles.

A quadrilateral can be classified as a rhombus if any of the following properties are true:

• All sides are equal.
• Both pairs of opposite sides are parallel and one pair of adjacent sides is equal.
• Both pairs of opposite angles are equal and one pair of adjacent sides is equal.

Worked Example 11.10: Calculating angles and sides of a rhombus

\(EFGH\) is a rhombus with \(\hat{H} = x + 40^{\circ}\), \(\hat{F} = 102^{\circ}\), \(FE= w\) and \(FG = 10 \text{ m}\). Find the values of \(x\) and \(w\).

Use the properties of a rhombus to find the unknown values.

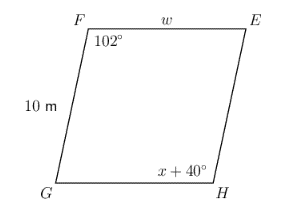
In rhombus \(EFGH\):

\[\begin{align} \hat{F} &= \hat{H} = 102^{\circ}\ (\text{opp. } \angle\text{s equal}) \\ \hat{H} &= x + 40^{\circ} = 102^{\circ} \\ x &= 102^{\circ} − 40^{\circ} \\ \therefore x &= 62^{\circ} \end{align}\]

In rhombus \(EFGH\):

\[\begin{align} FG &= FE = w\ (\text{equal sides of rhombus}) \\ FG &= 10 \text{ m} \\ \therefore w &= 10 \text{ m} \end{align}\]

Write the final answer.

In rhombus \(EFGH\), \(x = 62^{\circ}\) and \(w = 10 \text{ m}\)

Trapeziums

A trapezium is a quadrilateral with one pair of opposite sides parallel.

trapezium

a quadrilateral with one pair of opposite sides parallel

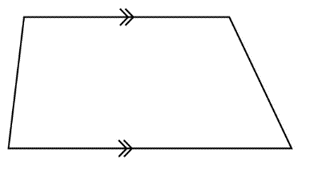
An isosceles trapezium has one pair of opposite sides parallel and the other pair of opposite sides equal. The base angles of an isosceles trapezium are equal.

Worked Example 11.11: Calculating angles of a trapezium

\(CDEF\) is a trapezium with \(\hat{C} = 82^{\circ}\), \(\hat{E} = 142^{\circ}\), \(\hat{F} = 98^{\circ}\) and \(\hat{D} = 2p\). Determine the value of \(p\).

Use the properties of a trapezium to find the unknown value.

In trapezium \(CDEF\):

\[\begin{align} \hat{C} + \hat{D} + \hat{E} +\hat{F} &= 360^{\circ}\ (\text{sum of } \angle\text{s quad.}) \\ 82^{\circ} + 2p + 142^{\circ} + 98^{\circ} &= 360^{\circ} \\ 2p &= 360^{\circ} − 322^{\circ} \\ 2p &= 38^{\circ} \\ \therefore p &= 19^{\circ} \end{align}\]

Write the final answer.

In trapezium \(CDEF\), \(p = 19^{\circ}\).

Kites

A kite is a quadrilateral with two pairs of adjacent sides equal. In kite \(ABCD\), the two shorter adjacent sides are equal, \(AD = CD\), and the two longer adjacent sides are equal, \(AB = CB\).

kite

a quadrilateral with two pairs of adjacent sides equal

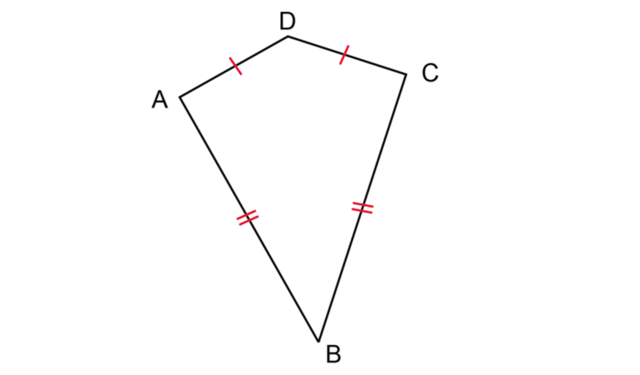
There is one pair of opposite angles that are equal.

Worked Example 11.12: Calculating angles of a kite

\(ABCD\) is a kite with \(\hat{A} = 2z + 16^{\circ}\), \(\hat{D} = 103^{\circ}\), \(\hat{C} = z\), \(AD = AB\) and \(CD = CB\). Find the values of \(z\).

Use the properties of a kite to find the unknown value.

In kite \(ABCD\):

\[\begin{align} \hat{D} &= \hat{B} = 103^{\circ}\ (\text{opp. } \angle\text{s equal}) \\ \hat{A} + \hat{B} + \hat{C} + \hat{D} &= 360^{\circ}\ (\text{sum of } \angle\text{s quad.}) \\ 2z + 16^{\circ} + 103^{\circ} + z + 103^{\circ} &= 360^{\circ} \\ 3z &= 360^{\circ} − 222^{\circ} \\ 3z &= 138^{\circ} \\ \therefore z &= 46^{\circ} \end{align}\]

Write the final answer.

In kite \(ABCD\), \(z = 46^{\circ}\).