Chapter 6: Trigonometry

Emphasize that the area/sine/cosine rule does not require a right-angled triangle.
Encourage learners to use the appropriate form of the area/sine/cosine rule.
Remind learners that angles in the Cartesian plane are always measured from the positive x-axis.
Important to note that:
$(270 \pm x)$ is not included in the section on reduction formulae.
co-function for tangent is not included.
Remind learners to check that their answers are within the required interval.
For the general solution, determine the solution in the correct quadrants and within the required interval.
Do not label all triangles as $ABC$. Learners must be able to apply the formulae with different notations and in different contexts.
Do not treat identities as equations: do NOT use = sign between the two expressions in an identity. Keep the LHS and RHS separate.
To prove identities, we usually manipulate the more complicated expression till it looks the same as the more simple expression.

6.1 Revision (EMBHG)

Trigonometric ratios

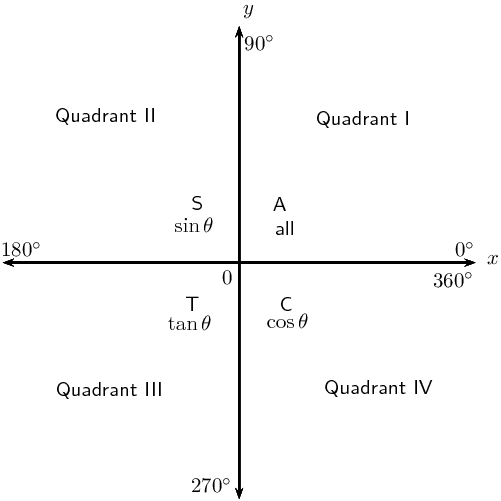
We plot the points $P(x;y)$ and $Q(-x;y)$ in the Cartesian plane and measure the angles from the positive $x$-axis to the terminal arms $(OP \text{ and } OQ)$.

$P(x;y)$ lies in the first quadrant with $P\hat{O}X = \alpha$ and $Q(-x;y)$ lies in the second quadrant with $Q\hat{O}X = \beta$.

Using the theorem of Pythagoras we have that

\begin{align*} OP^2 &= x^2 + y^2 \\ \text{And }OQ^2 &= (-x)^2 + y^2 \\ &= x^2 + y^2 \\ \therefore OP &= OQ \end{align*}

Let $OP = OQ = r$.

Trigonometric ratios

\begin{align*} \sin \alpha &= \frac{y}{r} \\ \cos \alpha &= \frac{x}{r}\\ \tan \alpha &= \frac{y}{x} \end{align*}

In the second quadrant we notice that $-x < 0$

\begin{align*} \sin \beta &= \frac{y}{r} \\ \cos \beta &= -\frac{x}{r}\\ \tan \beta &= -\frac{y}{x} \end{align*}

Similarily, in the third and fourth quadrants the sign of the trigonometric ratios depends on the signs of $x$ and $y$:

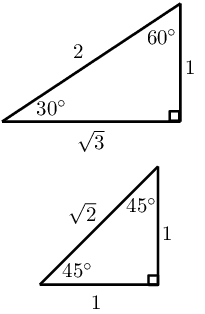
Special angles

θ	$\text{0}$$\text{°}$	$\text{30}$$\text{°}$	$\text{45}$$\text{°}$	$\text{60}$$\text{°}$	$\text{90}$$\text{°}$
$\cos θ$	$\text{1}$	$\frac{\sqrt{3}}{2}$	$\frac{1}{\sqrt{2}}$	$\frac{1}{2}$	$\text{0}$
$\sin θ$	$\text{0}$	$\frac{1}{2}$	$\frac{1}{\sqrt{2}}$	$\frac{\sqrt{3}}{2}$	$\text{1}$
$\tan θ$	$\text{0}$	$\frac{1}{\sqrt{3}}$	$\text{1}$	$\sqrt{3}$	undef

Solving equations

Worked example 1: Solving equations

Determine the values of $a$ and $b$ in the right-angled triangle $TUW$ (correct to one decimal place):

Identify the opposite and adjacent sides and the hypotenuse

Determine the value of $a$

\begin{align*} \sin \theta &= \frac{\text{opposite side}}{\text{hypotenuse}} \\ \sin \text{47}\text{°} &= \frac{30}{a} \\ a &= \frac{30}{\sin \text{47}\text{°}} \\ \therefore a &= \text{41,0} \end{align*}

Determine the value of $b$

Always try to use the information that is given for calculations and not answers that you have worked out in case you have made an error. For example, avoid using $a = \text{41,0}$ to determine the value of $b$.

\begin{align*} \tan \theta &= \frac{\text{opposite side}}{\text{adjacent side}} \\ \tan \text{47}\text{°} &= \frac{30}{b} \\ b &= \frac{30}{\tan \text{47}\text{°}} \\ \therefore b &= \text{28,0} \end{align*}

Write the final answer

$a = \text{41,0}$ units and $b = \text{28,0}$ units.

Finding an angle

Worked example 2: Finding an angle

Calculate the value of $\theta$ in the right-angled triangle $MNP$ (correct to one decimal place):

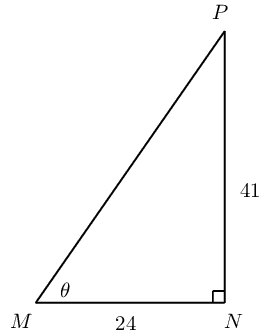
Identify the opposite and adjacent sides and the hypotenuse

Determine the value of $\theta$

\begin{align*} \tan \theta &= \frac{\text{opposite side}}{\text{adjacent side}} \\ \tan \theta &= \frac{41}{24} \\ \therefore \theta &= \tan^{-1} \left( \frac{41}{24} \right) \\ \theta &= \text{59,7}\text{°} \end{align*}

Worked example 3: Finding an angle

Given $2 \sin \frac{\theta}{2} = \cos \text{43}\text{°}, \text{ for } \theta \in [\text{0}\text{°};\text{90}\text{°}]$, determine the value of $\theta$ (correct to one decimal place).

Simplify the equation

Avoiding rounding off in calculations until you have determined the final answer. In the calculation below, the dots indicate that the number has not been rounded so that the answer is as accurate as possible.

\begin{align*} 2 \sin \frac{\theta}{2} &= \cos \text{43}\text{°} \\ \sin \frac{\theta}{2} &= \frac{\cos \text{43}\text{°}}{2} \\ \frac{\theta}{2} &= \sin^{-1} (\text{0,365} \ldots ) \\ \theta &= 2 (\text{21,449} \ldots ) \\ \therefore \theta &= \text{42,9}\text{°} \end{align*}

Two-dimensional problems

Worked example 4: Flying a kite

Thelma flies a kite on a $\text{22}$ $\text{m}$ piece of string and the height of the kite above the ground is $\text{20,4}$ $\text{m}$. Determine the angle of inclination of the string (correct to one decimal place).

Draw a sketch and identify the opposite and adjacent sides and the hypotenuse

Let the angle of inclination of the string be $\theta$.

Use an appropriate trigonometric ratio to find $\theta$

\begin{align*} \sin \theta &= \frac{\text{opposite side}}{\text{hypotenuse}} \\ &= \frac{\text{20,4}}{22} \\ \theta &= \sin^{-1} (\text{0,927} \ldots ) \\ \therefore \theta &= \text{68,0}\text{°} \end{align*}

Revision

Textbook Exercise 6.1

$\sin p + 3 \sin p = 4 \sin p$

\begin{align*} \text{LHS } &= \sin p + 3 \sin p \\ &= \sin \text{49}\text{°} + 3 \sin \text{49}\text{°} \\ &= \text{0,754} \ldots + \text{2,264} \ldots \\ &= \text{3,012} \\ \text{RHS } &= 4 \sin p \\ &= \text{3,012} \\ \therefore \text{LHS } &= \text{RHS } \end{align*}

$\dfrac{\sin q}{\cos q} = \tan q$

\begin{align*} \text{LHS } &= \dfrac{\sin q}{\cos q} \\ &= \frac{\sin \text{32}\text{°}}{\cos \text{32}\text{°}} \\ &= \frac{\text{0,53} \ldots }{\text{0,84} \ldots } \\ &= \text{0,62} \\ \text{RHS } &= \tan q \\ &= \tan \text{32}\text{°} \\ &= \text{0,62} \\ \therefore \text{LHS } &= \text{RHS } \end{align*}

$\cos (p - q) = \cos p - \cos q$

\begin{align*} \text{LHS } &= \cos (p - q)\\ &= \cos (\text{49}\text{°} - \text{32}\text{°})\\ &= \cos \text{17}\text{°} \\ &= \text{0,96} \\ \text{RHS } &= \cos p - \cos q \\ &= \cos \text{49}\text{°} - \cos \text{32}\text{°} \\ &= \text{0,65} \ldots - \text{0,84} \ldots \\ &= -\text{0,19} \\ \therefore \text{LHS } &\ne \text{RHS } \end{align*}

$\sin (2p) = 2\sin p \cos p$

\begin{align*} \text{LHS } &= \sin (2p) \\ &= \sin 2(\text{49}\text{°})\\ &= \sin \text{98}\text{°} \\ &= \text{0,99} \\ \text{RHS } &= 2 \sin p \cos p \\ &= 2 \sin \text{49}\text{°} \cos \text{49}\text{°} \\ &= 2 \times \text{0,75} \ldots \times \text{0,65} \ldots \\ &= \text{0,99} \\ \therefore \text{LHS } &= \text{RHS } \end{align*}

$\cos \alpha = \text{0,64}$

\begin{align*} \cos \alpha &= \text{0,64} \\ \therefore \alpha &= \cos^{-1} \text{0,64}\\ &= \text{50,2}\text{°} \end{align*}

$\sin \theta + 2 = \text{2,65}$

\begin{align*} \sin \theta + 2 &= \text{2,65} \\ \sin \theta &= \text{0,65} \\ \therefore \theta &= \sin^{-1} \text{0,65}\\ &= \text{40,5}\text{°} \end{align*}

$\frac{1}{2} \cos 2\beta = \text{0,3}$

\begin{align*} \frac{1}{2} \cos 2\beta &= \text{0,3} \\ \cos 2\beta &= \text{0,6} \\ \therefore 2\beta &= \cos^{-1} \text{0,6}\\ &= \text{53,1}\text{°} \ldots \\ \therefore \beta &= \frac{1}{2} \times \text{53,1}\text{°} \ldots \\ &= \text{26,6}\text{°} \end{align*}

$\tan \frac{\theta}{3} = \sin \text{48}\text{°}$

\begin{align*} \tan \frac{\theta}{3} &= \sin \text{48}\text{°} \\ &= \text{0,74} \ldots \\ \therefore \frac{\theta}{3} &= \tan^{-1} \text{0,74} \ldots \\ &= \text{36,6}\text{°} \ldots \\ \therefore \theta &= 3 \times \text{36,6}\text{°} \ldots \\ &= \text{109,8}\text{°} \end{align*}

$\cos 3p = \text{1,03}$

\begin{align*} \cos 3p &= \text{1,03} \\ \therefore 3p &= \cos^{-1} \text{1,03} \\ &= \text{No solution} \end{align*}

$2 \sin 3\beta + 1 = \text{2,6}$

\begin{align*} 2 \sin 3\beta + 1 &= \text{2,6} \\ 2 \sin 3\beta &= \text{1,6} \\ \sin 3\beta &= \text{0,8} \\ \therefore 3\beta &= \sin^{-1} \text{0,8} \\ &= \text{53,1}\text{°} \ldots \\ \therefore \beta &= \frac{1}{3} \times \text{53,1}\text{°} \ldots \\ &= \text{17,7}\text{°} \end{align*}

$\dfrac{\sin \theta}{\cos \theta} = 4\frac{2}{3}$

\begin{align*} \dfrac{\sin \theta}{\cos \theta} &= 4\frac{2}{3}\\ \therefore \tan \theta &= \text{2,66} \ldots \\ \therefore \theta &= \tan^{-1} \text{2,66} \ldots \\ &= \text{69,4}\text{°} \end{align*}

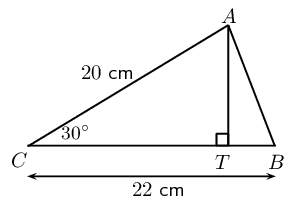
the length $TC$

\begin{align*} \text{In } \triangle CAT: \quad \cos \text{30}\text{°} &= \frac{TC}{CA}\\ &= \frac{TC}{20}\\ \therefore TC &= 20 \times \cos \text{30}\text{°} \\ &= \text{17,3}\text{ cm} \end{align*}

the length $AT$

\begin{align*} \text{In } \triangle CAT: \quad \sin \text{30}\text{°} &= \frac{AT}{CA}\\ &= \frac{AT}{20}\\ \therefore AT &= 20 \times \sin \text{30}\text{°} \\ &= \text{10}\text{ cm} \end{align*}

the angle $B\hat{A}T$

\begin{align*} CB &= CT + TB \\ 22 &= \text{17,3} + TB \\ \therefore TB &= 22 - \text{17,3} \\ &= \text{4,7}\text{ cm} \\ \text{In } \triangle BAT: \quad \tan \hat{B} &= \frac{AT}{BT}\\ &= \frac{10}{\text{4,7}} \\ \therefore \hat{B} &= \tan^{-1} \left( \frac{10}{\text{4,7}} \right) \\ &= \text{64,8}\text{°} \end{align*}

Determine the length of the sides.

\begin{align*} \text{Length sides } &= \frac{40}{4} \\ &= \text{10}\text{ cm} \end{align*}

Determine the lengths of the diagonals.

Diagonals of a rhombus bisect each other at right-angles and also bisect interior angles. Let the shorter diagonal be $2x$:

\begin{align*} \sin \text{15}\text{°} &= \frac{x}{10} \\ \therefore x &= 10 \sin \text{15}\text{°} \\ \therefore \text{Shorter diagonal }&= 2 \times 10 \sin \text{15}\text{°} \\ &= \text{5,2}\text{ cm} \end{align*}

Let the longer diagonal be $2y$:

\begin{align*} \cos \text{15}\text{°} &= \frac{y}{10} \\ \therefore y &= 10 \cos \text{15}\text{°} \\ \therefore \text{Longer diagonal }&= 2 \times 10 \cos \text{15}\text{°} \\ &= \text{19,3}\text{ cm} \end{align*}

Calculate the area of the rhombus.

\begin{align*} \text{Area } &= b \times h_{\perp} \\ &= 10 \times \text{19,3} \times \sin \text{15}\text{°} \\ &= \text{50}\text{ cm$^{2}$} \end{align*}

$2 \sin \text{45}\text{°} \times 2 \cos \text{45}\text{°}$

\begin{align*} 2 \sin \text{45}\text{°} \times 2 \cos \text{45}\text{°} &= 2 \times \frac{1}{\sqrt{2}} \times 2 \times \frac{1}{\sqrt{2}} \\ &= 4 \times \frac{1}{\left( \sqrt{2} \right)} \\ &= \frac{4}{2} \\ &= \text{2} \end{align*}

$\cos^2 \text{30}\text{°} - \sin^2 \text{60}\text{°}$

\begin{align*} \cos^2 \text{30}\text{°} - \sin^2 \text{60}\text{°} &= \left( \frac{\sqrt{3}}{2} \right)^2 - \left( \frac{\sqrt{3}}{2} \right)^2 \\ &= \text{0} \end{align*}

$\sin \text{60}\text{°} \cos \text{30}\text{°} - \cos \text{60}\text{°} \sin \text{30}\text{°} - \tan \text{45}\text{°}$

\begin{align*} \sin \text{60}\text{°} \cos \text{30}\text{°} &- \cos \text{60}\text{°} \sin \text{30}\text{°} - \tan \text{45}\text{°} \\ &=\frac{\sqrt{3}}{2} \times \frac{\sqrt{3}}{2}-\frac{1}{2} \times \frac{1}{2}-1\\ &=\frac{3}{4}-\frac{1}{4}-1\\ &=-1\frac{1}{2} \end{align*}

$4 \sin \text{60}\text{°} \cos \text{30}\text{°} - 2 \tan \text{45}\text{°} + \tan \text{60}\text{°} - 2 \sin \text{60}\text{°}$

\begin{align*} 4 \sin \text{60}\text{°} \cos \text{30}\text{°}& - 2 \tan \text{45}\text{°} + \tan \text{60}\text{°} - 2 \sin \text{60}\text{°} \\ &=4 \times \frac{\sqrt{3}}{2} \times \frac{\sqrt{3}}{2}- 2 \times 1 + \sqrt{3} - 2 \times \frac{\sqrt{3}}{2} \\ &= 3 - 2 + \sqrt{3} - \sqrt{3} \\ &=\text{1} \end{align*}

$\sin \text{60}\text{°} \times \sqrt{2 \tan \text{45}\text{°} + 1 } - \sin \text{30}\text{°}$

\begin{align*} \sin \text{60}\text{°}& \times \sqrt{2 \tan \text{45}\text{°} + 1 } - \sin \text{30}\text{°} \\ &= \frac{\sqrt{3}}{2} \times \sqrt{2(1) + 1 } - \frac{1}{2} \\ &= \frac{\sqrt{3}}{2} \times \sqrt{3} - \frac{1}{2} \\ &= \frac{3}{2} - \frac{1}{2} \\ &=\text{1} \end{align*}

$\beta$

\begin{align*} \tan\beta &=\frac{y}{x} \\ \tan\beta &=\frac{2\sqrt{3}}{2} \\ &=\sqrt{3}\\ \therefore \beta &= \text{60}\text{°} \end{align*}

$\cos \beta$

\begin{align*} OB^2 &= x^2 + y^2 \quad (\text{ Pythagoras}) \\ OB^2=2^2+(2\sqrt{3})^2\\ &=4+4(3) \\ &=16 \\ \therefore OB &= 4 \\ \cos\beta &= \frac{x}{OB} \\ &= \frac{2}{4} \\ &= \frac{1}{2} \end{align*}

$\cos^2 \beta + \sin^2 \beta$

\begin{align*} \cos^2 \beta + \sin^2 \beta &= \left( \frac{1}{2} \right)^2 + \left( \frac{2\sqrt{3}}{4} \right)^2 \\ &= \frac{1}{4} + \frac{3}{4}\\ &= \text{1} \end{align*}

The $\text{10}$ $\text{m}$ ladder of a fire truck leans against the wall of a burning building at an angle of $\text{60}\text{°}$. The height of an open window is $\text{9}$ $\text{m}$ from the ground. Will the ladder reach the window?

\begin{align*} \frac{h}{10}&=\sin \text{60}\text{°} \\ &=\frac{\sqrt{3}}{2}\\ \therefore h &=\frac{10\sqrt{3}}{2} \\ &=5\sqrt{3} \\ &= \text{8,66}\text{ m} \therefore \text{No, the ladder is too short.} \end{align*}

End of chapter exercises

Table of Contents

6.2 Trigonometric identities

θ	\(\text{0}\)\(\text{°}\)	\(\text{30}\)\(\text{°}\)	\(\text{45}\)\(\text{°}\)	\(\text{60}\)\(\text{°}\)	\(\text{90}\)\(\text{°}\)
\(\cos θ\)	\(\text{1}\)	\(\frac{\sqrt{3}}{2}\)	\(\frac{1}{\sqrt{2}}\)	\(\frac{1}{2}\)	\(\text{0}\)
\(\sin θ\)	\(\text{0}\)	\(\frac{1}{2}\)	\(\frac{1}{\sqrt{2}}\)	\(\frac{\sqrt{3}}{2}\)	\(\text{1}\)
\(\tan θ\)	\(\text{0}\)	\(\frac{1}{\sqrt{3}}\)	\(\text{1}\)	\(\sqrt{3}\)	undef

6.1 Revision

Chapter 6: Trigonometry

6.1 Revision (EMBHG)

Worked example 1: Solving equations

Identify the opposite and adjacent sides and the hypotenuse

Determine the value of \(a\)

Determine the value of \(b\)

Write the final answer

Worked example 2: Finding an angle

Identify the opposite and adjacent sides and the hypotenuse

Determine the value of \(\theta\)

Worked example 3: Finding an angle

Simplify the equation

Worked example 4: Flying a kite

Draw a sketch and identify the opposite and adjacent sides and the hypotenuse

Use an appropriate trigonometric ratio to find \(\theta\)

Revision