\(5; 20; 45; 80; \ldots\)
3.2 Quadratic sequences
|
Previous
3.1 Revision
|
Next
3.3 Summary
|
3.2 Quadratic sequences (EMBG5)
Quadratic sequences

- Study the dotted-tile pattern shown and answer the following questions.
- Complete the fourth pattern in the diagram.
-
Complete the table below:
pattern number \(\text{1}\) \(\text{2}\) \(\text{3}\) \(\text{4}\) \(\text{5}\) \(\text{20}\) \(n\) dotted tiles \(\text{1}\) \(\text{3}\) \(\text{5}\) difference (\(d\)) \(-\) \(\text{2}\) - What do you notice about the change in number of dotted tiles?
- Describe the pattern in words: “The number of dotted tiles...”.
- Write the general term: \(T_n = \ldots\)
- Give the mathematical name for this kind of pattern.
- A pattern has \(\text{819}\) dotted tiles. Determine the value of \(n\).
- Now study the number of blank tiles (tiles without dots) and answer the following questions:
-
Complete the table below:
pattern number \(\text{1}\) \(\text{2}\) \(\text{3}\) \(\text{4}\) \(\text{5}\) \(\text{10}\) blank tiles \(\text{3}\) \(\text{6}\) \(\text{11}\) first difference \(-\) \(\text{3}\) second difference \(-\) \(-\) - What do you notice about the change in the number of blank tiles?
- Describe the pattern in words: “The number of blank tiles...”.
- Write the general term: \(T_n = \ldots\)
- Give the mathematical name for this kind of pattern.
- A pattern has \(\text{227}\) blank tiles. Determine the value of \(n\).
- A pattern has \(\text{79}\) dotted tiles. Determine the number of blank tiles.
-
- Quadratic sequence
-
A quadratic sequence is a sequence of numbers in which the second difference between any two consecutive terms is constant.
Consider the following example: \(1; 2; 4; 7; 11; \ldots\)
The first difference is calculated by finding the difference between consecutive terms:

The second difference is obtained by taking the difference between consecutive first differences:
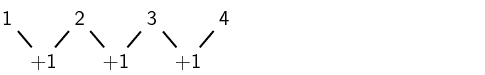
We notice that the second differences are all equal to \(\text{1}\). Any sequence that has a common second difference is a quadratic sequence.
It is important to note that the first differences of a quadratic sequence form a sequence. This sequence has a constant difference between consecutive terms. In other words, a linear sequence results from taking the first differences of a quadratic sequence.
General case
If the sequence is quadratic, the \(n^{\text{th}}\) term is of the form \({T}_{n}=a{n}^{2}+bn+c\).
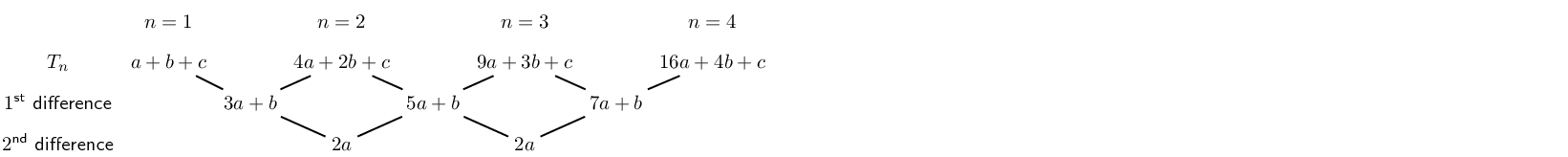
In each case, the common second difference is a \(2a\).
Quadratic sequences
Determine the second difference between the terms for the following sequences:
\(6; 11; 18; 27; \ldots\)
\(1; 4; 9; 16; \ldots\)
\(3; 0; -5; -12; \ldots\)
\(1; 3; 7; 13; \ldots\)
\(0; -6; -16; -30; \ldots\)
\(-1; 2; 9; 20; \ldots\)
\(1; -3; -9; -17; \ldots\)
\(3a+1; 12a+1; 27a+1; 48a+1 \ldots\)
\(2; 10; 24; 44; \ldots\)
\(t-2; 4t-1; 9t; 16t+1; \ldots\)
Complete the sequence by filling in the missing term:
\(11; 21; 35; \ldots; 75\)
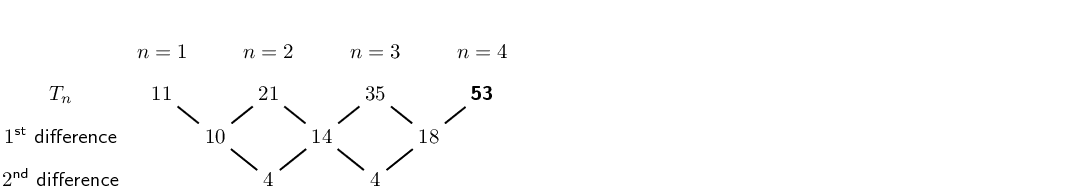
\(20; \ldots; 42; 56; 72\)
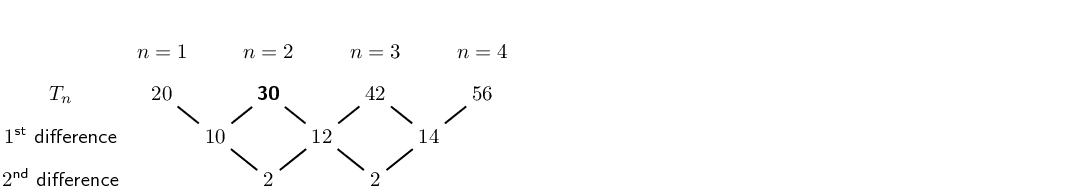
\(\ldots; 37; 65; 101\)

\(3; \ldots; -13; -27; -45\)
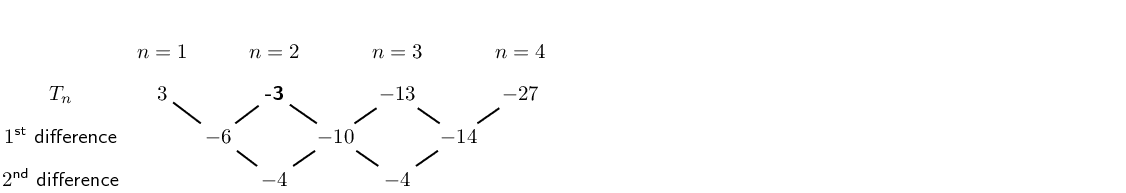
\(24; 35; 48; \ldots; 80\)
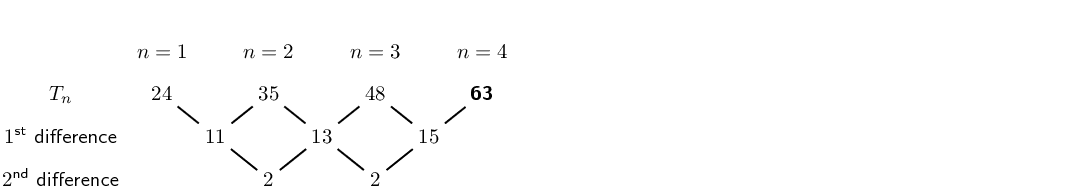
\(\ldots; 11; 26; 47\)
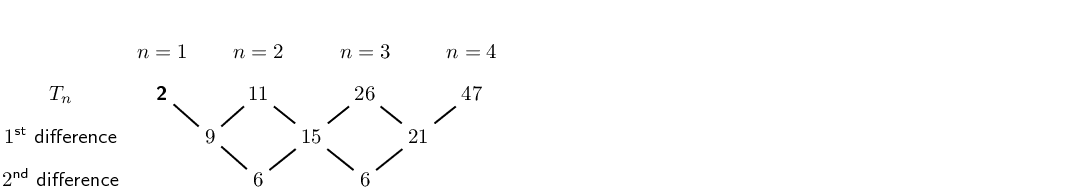
Use the general term to generate the first four terms in each sequence:
\(T_n = n^2+3n-1\)
\(T_n = -n^2-5\)
\(T_n = 3n^2-2n\)
\(T_n = -2n^2+n+1\)
Worked example 2: Quadratic sequences
Write down the next two terms and determine an equation for the \(n^{\text{th}}\) term of the sequence \(\text{5}\); \(\text{12}\); \(\text{23}\); \(\text{38}\); \(\ldots\)
Find the first differences between the terms
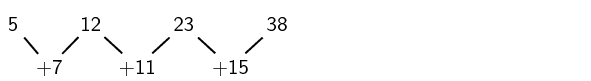
Find the second differences between the terms
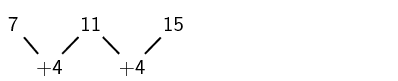
So there is a common second difference of \(\text{4}\). We can therefore conclude that this is a quadratic sequence of the form \(T_n = an^2 + bn + c\).
Continuing the sequence, the next first differences will be:
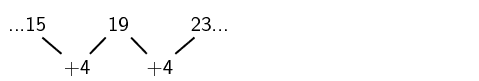
Finding the next two terms in the sequence
The next two terms will be:
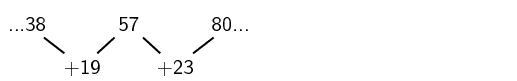
So the sequence will be: \(\text{5}\); \(\text{12}\); \(\text{23}\); \(\text{38}\); \(\text{57}\); \(\text{80}\); \(\ldots\)
Determine the general term for the sequence
To find the values of \(a\), \(b\) and \(c\) for \(T_n = an^2 + bn + c\) we look at the first \(\text{3}\) terms in the sequence:
\begin{align*} n=1: T_1 &= a + b + c \\ n=2: T_2 &= 4a + 2b + c \\ n=3: T_3 &= 9a + 3b + c \end{align*}We solve a set of simultaneous equations to determine the values of \(a\), \(b\) and \(c\)
We know that \(T_1 = 5\), \(T_2 = 12\) and \(T_3 = 23\)
\begin{align*} a + b + c &= 5 \\ 4a + 2b + c &= 12 \\ 9a + 3b + c &= 23 \end{align*}\begin{align*} T_2 - T_1 &= 4a + 2b + c - (a + b + c) \\ 12 - 5 &= 4a + 2b + c - a - b - c \\ 7 &= 3a + b \qquad \ldots (1) \end{align*}\begin{align*} T_3 - T_2 &= 9a + 3b + c - (4a + 2b + c) \\ 23 - 12 &= 9a + 3b + c - 4a - 2b - c \\ 11 &= 5a + b \qquad \ldots (2) \end{align*}\begin{align*} (2)-(1) &= 5a + b - (3a + b) \\ 11 - 7 &= 5a + b - 3a - b \\ 4 &= 2a \\ \therefore a &= 2 \end{align*}\begin{align*} \text{Using equation } (1): \quad 3(2) + b &= 7 \\ \therefore b &= 1 \\ \text{And using } \quad a + b + c &= 5 \\ 2 + 1 + c &= 5 \\ \therefore c &= 1 \end{align*}Write the general term for the sequence
\[T_n = 2n^2 + n + 2\]
Worked example 3: Plotting a graph of terms in a sequence
Consider the following sequence:
\[3; 6; 10; 15; 21; \ldots\]
- Determine the general term (\(T_n\)) for the sequence.
- Is this a linear or a quadratic sequence?
- Plot a graph of \(T_n\) vs \(n\).
Determine the first and second differences
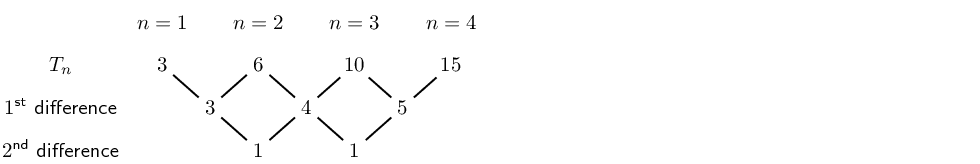
We see that the first differences are not constant and form the sequence \({3; 4; 5; \ldots}\) and that there is a common second difference of \(\text{1}\). Therefore the sequence is quadratic and has a general term of the form \(T_n = an^2 + bn + c\).
Determine the general term \(T_n\)
To find the values of \(a\), \(b\) and \(c\) for \(T_n = an^2 + bn + c\) we look at the first \(\text{3}\) terms in the sequence:
\begin{align*} n=1: T_1 &= a + b + c \\ n=2: T_2 &= 4a + 2b + c \\ n=3: T_3 &= 9a + 3b + c \end{align*}We solve this set of simultaneous equations to determine the values of \(a\), \(b\) and \(c\). We know that \(T_1 = 3\), \(T_2 = 6\) and \(T_3 = 10\).
\begin{align*} a + b + c &= 3 \\ 4a + 2b + c &= 6 \\ 9a + 3b + c &= 10 \end{align*}\begin{align*} T_2 - T_1 &= 4a + 2b + c - (a + b + c) \\ 6 - 3 &= 4a + 2b + c - a - b - c \\ 3 &= 3a + b \qquad \ldots (1) \end{align*}\begin{align*} T_3 - T_2 &= 9a + 3b + c - (4a + 2b + c) \\ 10 - 6 &= 9a + 3b + c - 4a - 2b - c \\ 4 &= 5a + b \qquad \ldots (2) \end{align*}\begin{align*} (2)-(1) &= 5a + b - (3a + b) \\ 4 - 3 &= 5a + b - 3a - b \\ 1 &= 2a \\ \therefore a &= \frac{1}{2} \end{align*}\begin{align*} \text{Using equation }(1): \quad 3\left(\frac{1}{2}\right) + b &= 3 \\ \therefore b &= \frac{3}{2} \\ \text{And using } \quad a + b + c &= 3 \\ \frac{1}{2} + \frac{3}{2} + c &= 3 \\ \therefore c &= 1 \end{align*}Therefore the general term for the sequence is \(T_n = \frac{1}{2}n^2 + \frac{3}{2}n + 1\).
Plot a graph of \(T_n\) vs \(n\)
Use the general term for the sequence, \(T_n = \frac{1}{2}n^2 + \frac{3}{2}n + 1\), to complete the table.
| \(n\) | \(\text{1}\) | \(\text{2}\) | \(\text{3}\) | \(\text{4}\) | \(\text{5}\) | \(\text{6}\) | \(\text{7}\) | \(\text{8}\) | \(\text{9}\) | \(\text{10}\) |
| \(T_n\) | \(\text{3}\) | \(\text{6}\) | \(\text{10}\) | \(\text{15}\) | \(\text{21}\) | \(\text{28}\) | \(\text{36}\) | \(\text{45}\) | \(\text{55}\) | \(\text{66}\) |
Use the table to plot the graph:
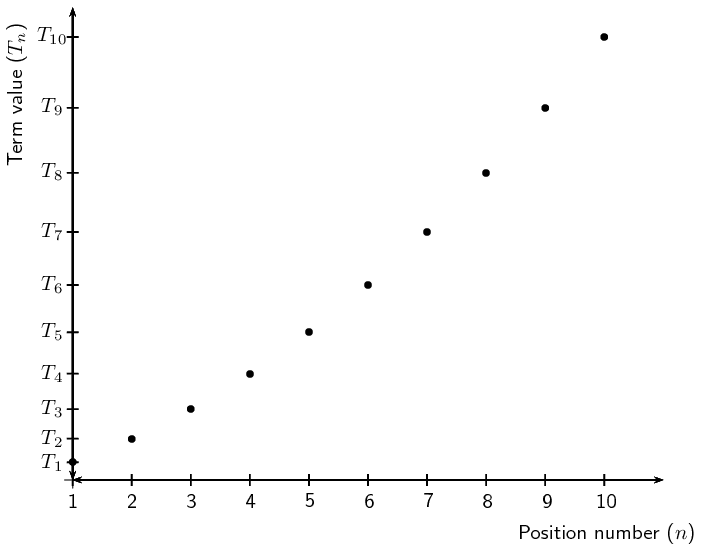
In this case it would not be accurate to join these points, since \(n\) indicates the position of a term in a sequence and can therefore only be a positive integer. We can, however, see that the plot of the points lies in the shape of a parabola.
Worked example 4: Olympic Games soccer event
In the first stage of the soccer event at the Olympic Games, there are teams from four different countries in each group. Each country in a group must play every other country in the group once.
- How many matches will be played in each group in the first stage of the event?
- How many matches would be played if there are \(\text{5}\) teams in each group?
- How many matches would be played if there are \(\text{6}\) teams in each group?
- Determine the general formula of the sequence.
Determine the number of matches played if there are \(\text{4}\) teams in a group
Let the teams from four different countries be \(A\), \(B\), \(C\) and \(D\).
| teams in a group | matches played |
| \(A\) | \(AB, AC, AD\) |
| \(B\) | \(BC, BD\) |
| \(C\) | \(CD\) |
| \(D\) | |
| \(\text{4}\) | \(3+2+1=6\) |
\(AB\) means that team \(A\) plays team \(B\) and \(BA\) would be the same match as \(AB\). So if there are four different teams in a group, each group plays \(\text{6}\) matches.
Determine the number of matches played if there are \(\text{5}\) teams in a group
Let the teams from five different countries be \(A, B, C, D\) and \(E\).
| teams in a group | matches played |
| \(A\) | \(AB, AC, AD, AE\) |
| \(B\) | \(BC, BD, BE\) |
| \(C\) | \(CD, CE\) |
| \(D\) | \(DE\) |
| \(E\) | |
| \(\text{5}\) | \(4+3+2+1=10\) |
So if there are five different teams in a group, each group plays \(\text{10}\) matches.
Determine the number of matches played if there are \(\text{6}\) teams in a group
Let the teams from six different countries be \(A, B, C, D, E\) and \(F\).
| teams in a group | matches to be played |
| \(A\) | \(AB, AC, AD, AE, AF\) |
| \(B\) | \(BC, BD, BE, BF\) |
| \(C\) | \(CD, CE, CF\) |
| \(D\) | \(DE, DF\) |
| \(E\) | \(EF\) |
| \(F\) | |
| \(\text{5}\) | \(5+4+3+2+1=15\) |
So if there are six different teams in a group, each group plays \(\text{15}\) matches.
We continue to increase the number of teams in a group and find that a group of \(\text{7}\) teams plays \(\text{21}\) matches and a group of \(\text{8}\) teams plays \(\text{28}\) matches.
Consider the sequence
We examine the sequence to determine if it is linear or quadratic:

We see that the first differences are not constant and that there is a common second difference of \(\text{1}\). Therefore the sequence is quadratic and has a general term of the form \(T_n = an^2 + bn + c\).
Determine the general term \(T_n\)
To find the values of \(a\), \(b\) and \(c\) for \(T_n = an^2 + bn + c\) we look at the first \(\text{3}\) terms in the sequence:
\begin{align*} n=1: T_1 &= a + b + c \\ n=2: T_2 &= 4a + 2b + c \\ n=3: T_3 &= 9a + 3b + c \end{align*}We solve a set of simultaneous equations to determine the values of \(a\), \(b\) and \(c\). We know that \(T_1 = 6\), \(T_2 = 10\) and \(T_3 = 15\)
\begin{align*} a + b + c &= 6 \\ 4a + 2b + c &= 10 \\ 9a + 3b + c &= 15 \end{align*}\begin{align*} T_2 - T_1 &= 4a + 2b + c - (a + b + c) \\ 10 - 6 &= 4a + 2b + c - a - b - c \\ 4 &= 3a + b \qquad \ldots (1) \end{align*}\begin{align*} T_3 - T_2 &= 9a + 3b + c - (4a + 2b + c) \\ 15- 10 &= 9a + 3b + c - 4a - 2b - c \\ 5 &= 5a + b \qquad \ldots (2) \end{align*}\begin{align*} (2)-(1) &= 5a + b - (3a + b) \\ 5-4 &= 5a + b - 3a - b \\ 1 &= 2a \\ \therefore a &= \frac{1}{2} \end{align*}\begin{align*} \text{Using equation } (1): \quad 3\left(\frac{1}{2}\right) + b &= 4 \\ \therefore b &= \frac{5}{2} \\ \text{And using } a + b + c &= 6 \\ \frac{1}{2} + \frac{5}{2} + c &= 6 \\ \therefore c &= 3 \end{align*}Therefore the general term for the sequence is \(T_n = \frac{1}{2}n^2 + \frac{5}{2}n + 3\).
Quadratic sequences
Calculate the common second difference for each of the following quadratic sequences:
\(3; \; 6; \; 10; \; 21; \; ...\)
\(4; \; 9; \; 16; \; 25; \; ...\)
\(7; \; 17; \; 31; \; 49; \; ...\)
\(2; \; 10; \; 26; \; 50; \; 82 ...\)
\(31; \; 30; \; 27; \; 22; \; 15; \; ...\)
Find the first five terms of the quadratic sequence defined by: \(T_n = 5n^2 + 3n + 4\).
Given \(T_n = 4n^2 + 5n + 10\), find \(T_9\).
Given \(T_n = 2n^2\), for which value of \(n\) does \(T_n = 32\)?
Write down the next two terms of the quadratic sequence: \(16; 27; 42; 61; \ldots\)
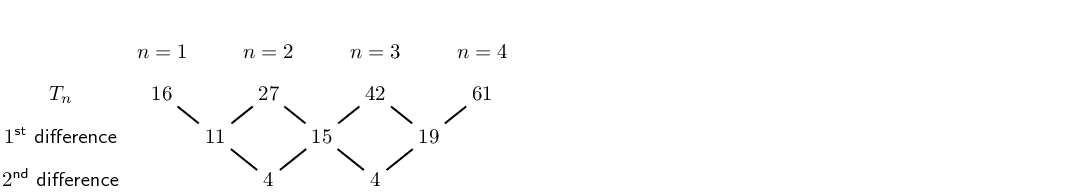
Find the general formula for the quadratic sequence above.
|
Previous
3.1 Revision
|
Table of Contents |
Next
3.3 Summary
|
