|
Previous
10.2 Measures of central tendency
|
Next
10.4 Measures of dispersion
|
A common way of handling continuous quantitative data is to subdivide the full range of values into a few sub-ranges. By assigning each continuous value to the sub-range or class within which it falls, the data set changes from continuous to discrete.
Grouping is done by defining a set of ranges and then counting how many of the data fall inside each range. The sub-ranges must not overlap and must cover the entire range of the data set.
One way of visualising grouped data is as a histogram. A histogram is a collection of rectangles, where the base of a rectangle (on the \(x\)-axis) covers the values in the range associated with it, and the height of a rectangle corresponds to the number of values in its range.
The following video explains how to group data.
The heights in centimetres of \(\text{30}\) learners are given below.
|
\(\text{142}\) |
\(\text{163}\) |
\(\text{169}\) |
\(\text{132}\) |
\(\text{139}\) |
\(\text{140}\) |
\(\text{152}\) |
\(\text{168}\) |
\(\text{139}\) |
\(\text{150}\) |
|
\(\text{161}\) |
\(\text{132}\) |
\(\text{162}\) |
\(\text{172}\) |
\(\text{146}\) |
\(\text{152}\) |
\(\text{150}\) |
\(\text{132}\) |
\(\text{157}\) |
\(\text{133}\) |
|
\(\text{141}\) |
\(\text{170}\) |
\(\text{156}\) |
\(\text{155}\) |
\(\text{169}\) |
\(\text{138}\) |
\(\text{142}\) |
\(\text{160}\) |
\(\text{164}\) |
\(\text{168}\) |
Group the data into the following ranges and draw a histogram of the grouped data:
\begin{align*} 130 \le h < 140 \\ 140 \le h < 150 \\ 150 \le h < 160 \\ 160 \le h < 170 \\ 170 \le h < 180 \end{align*}(Note that the ranges do not overlap since each one starts where the previous one ended.)
|
Range |
Count |
|
\(130\le h<140\) |
\(\text{7}\) |
|
\(140\le h<150\) |
\(\text{5}\) |
|
\(150\le h<160\) |
\(\text{7}\) |
|
\(160\le h<170\) |
\(\text{9}\) |
|
\(170\le h<180\) |
\(\text{2}\) |
Since there are \(\text{5}\) ranges, the histogram will have \(\text{5}\) rectangles. The base of each rectangle is defined by its range. The height of each rectangle is determined by the count in its range.
The histogram makes it easy to see in which range most of the heights are located and provides an overview of the distribution of the values in the data set.
A group of \(\text{10}\) learners count the number of playing cards they each have. This is a histogram describing the data they collected:
Count the number of playing cards in the following range: \(\text{0} \le \text{number of playing cards} \le \text{2}\)
From the graph the answer is: 1
From the histogram, we arrive at our answer by reading the height of the specified interval from the histogram.
A group of \(\text{15}\) learners count the number of stones they each have. This is a histogram describing the data they collected:
Count the number of stones in the following range: \(\text{0} \le \text{number of stones} \le \text{2}\)
From the graph the answer is: 1
From the histogram, we arrive at our answer by reading the height of the specified interval from the histogram.
A group of \(\text{20}\) learners count the number of playing cards they each have. This is the data they collect:
\[\begin{array}{c c c c c} 14 & 9 & 11 & 8 & 13 \\ 2 & 3 & 4 & 16 & 17 \\ 9 & 19 & 10 & 14 & 4 \\ 16 & 16 & 11 & 2 & 17 \end{array}\]
Count the number of learners who have from \(\text{12}\) up to \(\text{15}\) playing cards. In other words, how many learners have playing cards in the following range: \(\text{12} \le \text{number of playing cards} \le \text{15}\)? It may be helpful for you to draw a histogram in order to answer the question.
Firstly we sort the table into sequential order, starting with the smallest value.
\[\begin{array}{c c c c c} 2 & 2 & 3 & 4 & 4 \\ 8 & 9 & 9 & 10 & 11 \\ 11 & 13 & 14 & 14 & 16 \\ 16 & 16 & 17 & 17 & 19 \end{array}\]
Secondly, we draw a histogram of the data:
From the histogram you can see that the number of learners with playing cards in the range: \(\text{12} \le \text{number of playing cards} \le \text{15}\) is 3.
A group of \(\text{20}\) learners count the number of stones they each have. This is the data they collect:
\[\begin{array}{c c c c c} 16 & 6 & 11 & 19 & 20 \\ 17 & 13 & 1 & 5 & 12 \\ 5 & 2 & 16 & 11 & 16 \\ 6 & 10 & 13 & 6 & 17 \end{array}\]
Count the number of learners who have from \(\text{4}\) up to \(\text{7}\) stones. In other words, how many learners have stones in the following range: \(\text{4} \le \text{number of stones} \le \text{7}\)? It may be helpful for you to draw a histogram in order to answer the question.
Firstly we sort the table into sequential order, starting with the smallest value.
\[\begin{array}{c c c c c} 1 & 2 & 5 & 5 & 6 \\ 6 & 6 & 10 & 11 & 11 \\ 12 & 13 & 13 & 16 & 16 \\ 16 & 17 & 17 & 19 & 20 \end{array}\]
Secondly, we draw a histogram of the data:
From the histogram you can see that the number of learners with stones in the range: \(\text{4} \le \text{number of stones} \le \text{7}\) is 5.
A group of 20 learners count the number of stones they each have. The learners draw a histogram describing the data they collected. However, they have made a mistake in drawing the histogram.
The data set below shows the correct information for the number of stones the learners have. Each value represents the number of stones for one learner.
\[\{ 4 ; 12 ; 15 ; 14 ; 18 ; 12 ; 17 ; 15 ; 1 ; 6 ; 6 ; 12 ; 6 ; 8 ; 6 ; 8 ; 17 ; 19 ; 16 ; 8\}\]Help them figure out which column in the histogram is incorrect.
We first need to order the data:
\[\{1 ; 4 ; 6 ; 6 ; 6 ; 6 ; 8 ; 8 ; 8 ; 12 ; 12 ; 12 ; 14 ; 15 ; 15 ; 16 ; 17 ; 17 ; 18 ; 19\}\]Using the ordered data set we can group the data and draw the correct histogram:
The column with the error in it was: E.
The learners used the incorrect value of 0, when the correct value is \(5\).
A group of 20 learners count the number of stones they each have. The learners draw a histogram describing the data they collected. However, they have made a mistake in drawing the histogram.
The data set below shows the correct information for the number of stones the learners have. Each value represents the number of stones for one learner.
\[\{ 19 ; 11 ; 5 ; 2 ; 3 ; 4 ; 14 ; 2 ; 12 ; 19 ; 11 ; 14 ; 2 ; 19 ; 11 ; 5 ; 17 ; 10 ; 1 ; 12\}\]Help them figure out which column in the histogram is incorrect.
We first need to order the data:
\[\{1 ; 2 ; 2 ; 2 ; 3 ; 4 ; 5 ; 5 ; 10 ; 11 ; 11 ; 11 ; 12 ; 12 ; 14 ; 14 ; 17 ; 19 ; 19 ; 19\}\]Using the ordered data set we can group the data and draw the correct histogram:
The column with the error in it was: B.
The learners used the incorrect value of 5, when the correct value is \(3\).
A group of learners count the number of sweets they each have. This is a histogram describing the data they collected:
A cat jumps onto the table, and all their notes land on the floor, mixed up, by accident!
Help them find which of the following data sets match the above histogram:
Data Set A
\[\begin{array}{c c c c c} 2 & 1 & 20 & 10 & 5 \\ 3 & 10 & 2 & 6 & 1 \\ 2 & 2 & 17 & 3 & 18 \\ 3 & 7 & 10 & 8 & 18 \end{array}\]Data Set B
\[\begin{array}{c c c c c} 2 & 9 & 12 & 10 & 5 \\ 9 & 9 & 10 & 13 & 6 \\ 5 & 11 & 10 & 7 & 7 \end{array}\]Data Set C
\[\begin{array}{c c c c c} 3 & 12 & 16 & 10 & 15 \\ 17 & 18 & 2 & 3 & 7 \\ 11 & 12 & 8 & 2 & 7 \\ 17 & 3 & 11 & 4 & 4 \end{array}\]The correct answer is: Data Set C
A group of learners count the number of stones they each have. This is a histogram describing the data they collected:
A cleaner knocks over their table, and all their notes land on the floor, mixed up, by accident!
Help them find which of the following data sets match the above histogram:
Data Set A
\[\begin{array}{c c c c c} 12 & 4 & 2 & 15 & 10 \\ 18 & 10 & 16 & 16 & 19 \\ 1 & 2 & 9 & 10 & 16 \\ 10 & 11 & 9 & 2 & 13 \end{array}\]Data Set B
\[\begin{array}{c c c c c} 7 & 10 & 4 & 5 & 8 \\ 7 & 12 & 10 & 14 & 5 \\ 1 & 9 & 2 & 13 & 3 \end{array}\]Data Set C
\[\begin{array}{c c c c c} 9 & 3 & 8 & 5 & 8 \\ 5 & 8 & 1 & 4 & 3 \end{array}\]The correct answer is: Data Set C
A class experiment was conducted and \(\text{50}\) learners were asked to guess the number of sweets in a jar. The following guesses were recorded:
|
\(\text{56}\) |
\(\text{49}\) |
\(\text{40}\) |
\(\text{11}\) |
\(\text{33}\) |
\(\text{33}\) |
\(\text{37}\) |
\(\text{29}\) |
\(\text{30}\) |
\(\text{59}\) |
|
\(\text{21}\) |
\(\text{16}\) |
\(\text{38}\) |
\(\text{44}\) |
\(\text{38}\) |
\(\text{52}\) |
\(\text{22}\) |
\(\text{24}\) |
\(\text{30}\) |
\(\text{34}\) |
|
\(\text{42}\) |
\(\text{15}\) |
\(\text{48}\) |
\(\text{33}\) |
\(\text{51}\) |
\(\text{44}\) |
\(\text{33}\) |
\(\text{17}\) |
\(\text{19}\) |
\(\text{44}\) |
|
\(\text{47}\) |
\(\text{23}\) |
\(\text{27}\) |
\(\text{47}\) |
\(\text{13}\) |
\(\text{25}\) |
\(\text{53}\) |
\(\text{57}\) |
\(\text{28}\) |
\(\text{23}\) |
|
\(\text{36}\) |
\(\text{35}\) |
\(\text{40}\) |
\(\text{23}\) |
\(\text{45}\) |
\(\text{39}\) |
\(\text{32}\) |
\(\text{58}\) |
\(\text{22}\) |
\(\text{40}\) |
Draw up a grouped frequency table using the intervals \(10 < x \le 20\), \(20 < x \le 30\), \(30 < x \le 40\), \(40 < x \le 50\) and \(50 < x \le 60\).
|
Group |
Frequency |
|
\(10 < x \le 20\) |
\(\text{6}\) |
|
\(20 < x \le 30\) |
\(\text{13}\) |
|
\(30 < x \le 40\) |
\(\text{15}\) |
|
\(40 < x \le 50\) |
\(\text{9}\) |
|
\(50 < x \le 60\) |
\(\text{7}\) |
Draw the histogram corresponding to the frequency table of the grouped data.
With grouped data our estimates of central tendency will change because we lose some information when we place each value in a range. If all we have to work with is the grouped data, we do not know the measured values to the same accuracy as before. The best we can do is to assume that values are grouped at the centre of each range.
Looking back to the previous worked example, we started with this data set of learners' heights.
\begin{align*} \big\{132; 132; 132; 133; 138; 139; 139; 140; 141; 142; 142; 146; 150; 150; 152; \\ 152; 155; 156; 157; 160; 161; 162; 163; 164; 168; 168; 169; 169; 170; 172 \big\} \end{align*}Note that the data are sorted.
The mean of these data is \(\text{151,8}\) and the median is \(\text{152}\). The mode is \(\text{132}\), but remember that there are problems with computing the mode of continuous quantitative data.
After grouping the data, we now have the data set shown below. Note that each value is placed at the centre of its range and that the number of times that each value is repeated corresponds exactly to the counts in each range.
\begin{align*} \big\{ 135; 135; 135; 135; 135; 135; 135; 145; 145; 145; 145; 145; 155; 155; 155; \\ 155; 155; 155; 155; 165; 165; 165; 165; 165; 165; 165; 165; 165; 175; 175 \big\} \end{align*}The grouping changes the measures of central tendency since each datum is treated as if it occurred at the centre of the range in which it was placed.
The mean is now \(\text{153}\), the median \(\text{155}\) and the mode is \(\text{165}\). This is actually a better estimate of the mode, since the grouping showed in which range the learners' heights were clustered.
We can also just give the modal group and the median group for grouped data. The modal group is the group that has the highest number of data values. The median group is the central group when the groups are arranged in order.
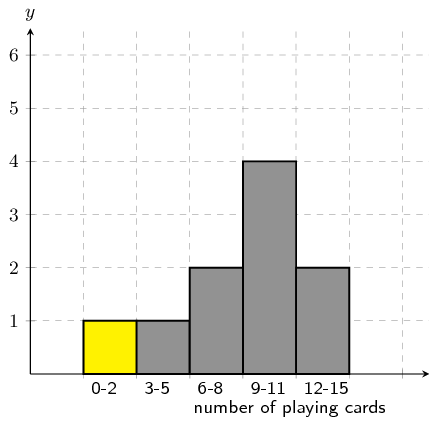
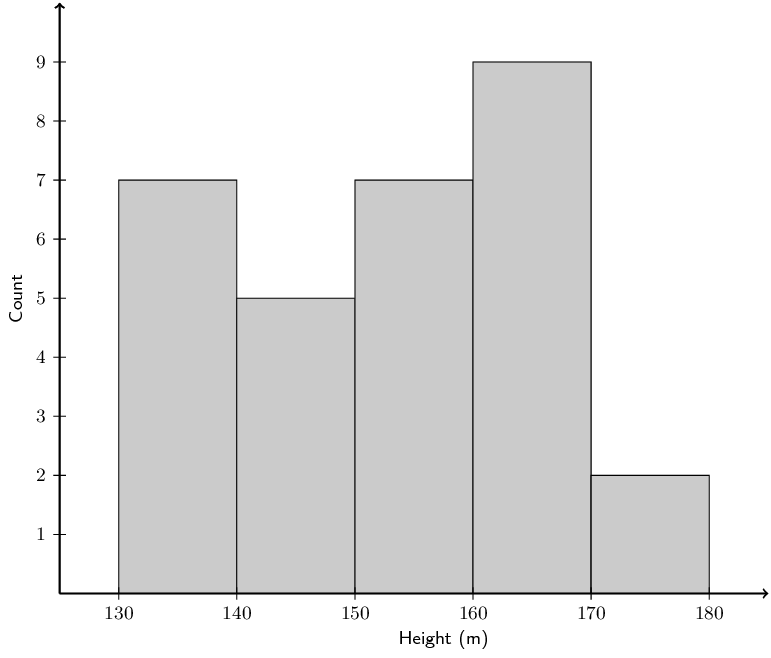
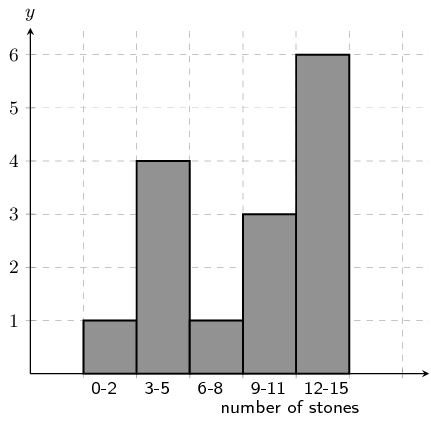
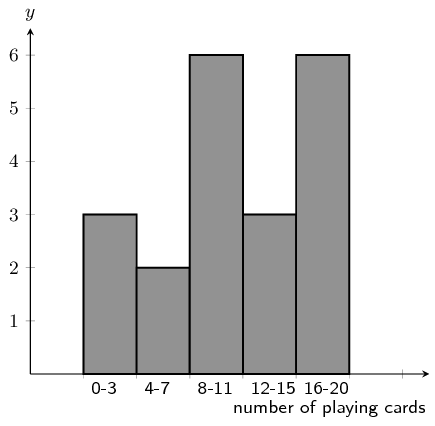
Consider the following grouped data and calculate the mean, the modal group and the median group.
|
Mass (\(\text{kg}\)) |
Count |
|
\(40 < m \le 45\) |
\(\text{7}\) |
|
\(45 < m \le 50\) |
\(\text{10}\) |
|
\(50 < m \le 55\) |
\(\text{15}\) |
|
\(55 < m \le 60\) |
\(\text{12}\) |
|
\(60 < m \le 65\) |
\(\text{6}\) |
To find the mean we use the middle value for each group. The count then tells us how many times that value occurs in the data set. Therefore the mean is:
\begin{align*} \text{mean } & = \frac{7(43) + 10(48) + 15(53) + 12(58) + 6(63)}{7 + 10 + 15 + 12 + 6} \\ & = \frac{2650}{50} \\ & = 53 \end{align*}The modal group is the group with the highest number of data values. This is \(50 <m \le 55\) with 15 data values.
The median group is the central group. There are 5 groups and so the central group is the third one: \(50 <m \le 55\).
Mean: \(\text{52}\); Modal group: \(50 <m \le 55\); Median group: \(50 < m \le 55\).
Find the mean, the modal group and the median group in this data set of how much time people needed to complete a game.
|
Time (s) |
Count |
|
\(35 < t \le 45\) |
\(\text{5}\) |
|
\(45 < t \le 55\) |
\(\text{11}\) |
|
\(55 < t \le 65\) |
\(\text{15}\) |
|
\(65 < t \le 75\) |
\(\text{26}\) |
|
\(75 < t \le 85\) |
\(\text{19}\) |
|
\(85 < t \le 95\) |
\(\text{13}\) |
|
\(95 < t \le 105\) |
\(\text{6}\) |
To find the mean we use the middle value for each group. The count then tells us how many times that value occurs in the data set. Therefore the mean is:
\begin{align*} \text{mean } & = \frac{5(\text{40,5}) + 11(\text{50,5}) + 15(\text{60,5}) + 26(\text{70,5}) + 19(\text{80,5}) + 13(\text{90,5}) + 6(\text{100,5})}{5 + 11 + 15 + 26 + 19 + 13 + 6} \\ & = \frac{\text{6 807,5}}{95} \\ & = \text{71,66} \end{align*}The modal group is the group with the highest number of data values. This is \(65 <m \le 75\) with 26 data values.
The median group is the central group. There are 7 groups and so the central group is the fourth one: \(65 <m \le 75\).
Mean: \(\text{70,66}\); Modal group: \(65 < t \le 75\); Median group: \(65 < t \le 75\).
The histogram below shows the number of passengers that travel in Alfred's minibus taxi per week.
Calculate:
the modal interval
The modal interval is the interval with the highest number of data values. For this data set it is: \(700 < x\le 800\) with 16 values.
the total number of passengers to travel in Alfred's taxi
We add up the counts in each group and then multiply these counts with the central value for each group: \(4(450) + 6(550) + 12(650) + 16(750) + 8(850) + 2(950) = \text{33 600}\).
an estimate of the mean
There are 48 values in the data set. Therefore the mean is \(\dfrac{\text{33 600}}{48} = \text{700}\).
an estimate of the median
We are looking for an estimate of the median rather than the median group here. In this case we note that there are 48 data values in the data set. Therefore the median will lie between the \(24^{\text{th}}\) and \(25^{\text{th}}\) values.
We note that 22 values in the first 3 groups and 38 values in the first four groups so the median must lie in the fourth group. Therefore we can estimate the median as the middle value of the fourth group: \(\text{750}\).
if it is estimated that every passenger travelled an average distance of \(\text{5}\) \(\text{km}\), how much money would Alfred have made if he charged \(\text{R}\,\text{3,50}\) per km?
\(\text{3,50} \times 5 \times \text{33 600} = \text{R}\,\text{588 000}\).
|
Previous
10.2 Measures of central tendency
|
Table of Contents |
Next
10.4 Measures of dispersion
|
