Measure the area of the bottom of the boxes, and the diameter of the cans. Using the length and width calculation method from the previous worked example, estimate how many cans you should be able to fit into each box.
learner-dependent answer
|
Previous
10.3 Floor plans
|
Next
End of chapter activity
|
When items are packed into a limited space like a box, cupboard or suitcase, how they are packed often determines how many items can fit into the space. A good example of this is trying to pack everything you need for your school day (like your books, sports equipment and food) into your school bag or backpack.
Your books must not be damaged in the process and you have to be able to carry everything yourself.
These same limits apply to posting a package to a friend or family member. An additional aspect to consider is the cost of the postage which is regularly determined by weight. Sending an extra container costs extra money as well. If what you are posting is fragile extra protective material must be packed between the items to make sure they reach the destination whole.

Vuyo and Sipho's father own a biscuit business called Biscuits for Africa. It is the June/July school holidays and, to make more pocket money, their father has employed Vuyo and Sipho for one week to help package the biscuits into boxes so they can be transported to stores.

Vuyo's and Sipho's father tells them that each large box can hold a maximum weight of \(\text{3,5}\) \(\text{kg}\).
If each can of chocolate biscuits weighs \(\text{300}\) \(\text{g}\), how many cans can Sipho pack into a bigger box without exceeding the weight limit?
The boys' father orders new large shipping boxes that are \(\text{45}\) \(\text{cm}\) long and \(\text{16}\) \(\text{cm}\) wide.
Length of large box = \(\text{45}\) \(\text{cm}\).
\(\text{45}\) \(\text{cm}\) \(\div\) \(\text{5}\) \(\text{cm}\) = \(\text{9}\) boxes.
Width of large box = \(\text{16}\) \(\text{cm}\).
\(\text{16}\) \(\text{cm}\) \(\div\) \(\text{5}\) \(\text{cm}\) = \(\text{3,2}\) boxes. Vuyo can't pack \(\text{0,2}\) of a box, so we round this down to the nearest whole number, \(\text{3}\) boxes.
So Vuyo can fit \(\text{9}\) rows of \(\text{3}\) boxes each. \(\text{9}\) \(\times\) \(\text{3}\) = \(\text{27}\) boxes.
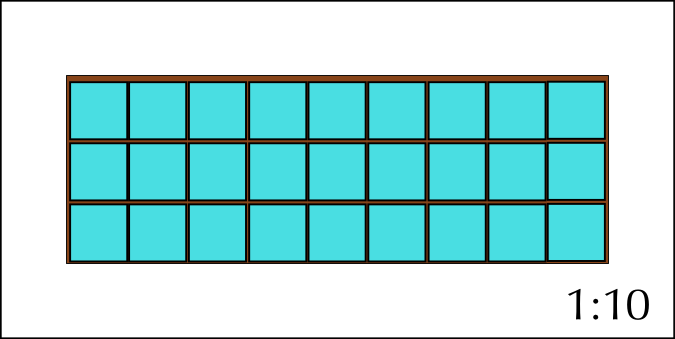
Length of large box = \(\text{45}\) \(\text{cm}\).
\(\text{45}\) \(\text{cm}\) \(\div\) \(\text{5}\) \(\text{cm}\) diameter = \(\text{9}\) cans.
Width of large box = \(\text{16}\) \(\text{cm}\).
\(\text{16}\) \(\text{cm}\) \(\div\) \(\text{5}\) \(\text{cm}\) diameter = \(\text{3,2}\) cans. Sipho can't pack \(\text{0,2}\) of a can, so we round this down to the nearest whole number: \(\text{3}\) cans.
So Sipho can fit \(\text{9}\) rows of \(\text{3}\) cans each. \(\text{9}\) \(\times\) \(\text{3}\) = \(\text{27}\) cans.
Sipho can fit \(\text{27}\) cans of chocolate biscuits into the new large shipping boxes.
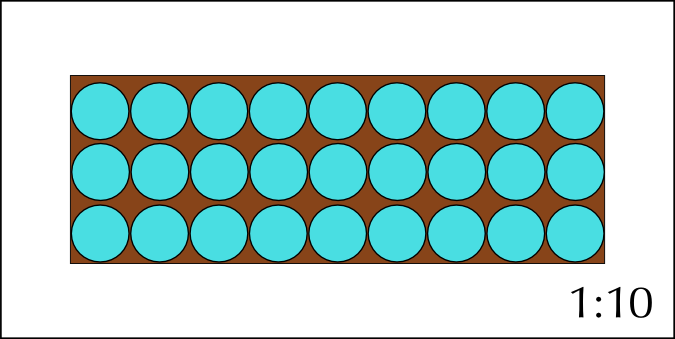
For this activity you will need collect a number cans and at least three boxes of different sizes.
Measure the area of the bottom of the boxes, and the diameter of the cans. Using the length and width calculation method from the previous worked example, estimate how many cans you should be able to fit into each box.
learner-dependent answer
Through trial and error find the best way to fit as many cans as possible into each box without damaging either the cans or the box.
learner-dependent answer
Draw a top view of your final packing arrangement.
learner-dependent answer
Compare your layout with the rest of your class.
learner-dependent answer
Would it have been easier to pack boxes than cans? Motivate your answer.
learner-dependent answer
How do you know that the cans are cylindrical rather than box-shaped? (Hint: Look at the shapes of the bases.)

learner-dependent answer.
|
Previous
10.3 Floor plans
|
Table of Contents |
Next
End of chapter activity
|