How are the debits and credits indicated on this statement?
As payments (debits) and deposits (credits) respectively.
|
Previous
11.1 Introduction and key concepts
|
Next
11.3 Interest
|
When you open an account for the first time, you need to be familiar with the different types of accounts that are available. Banks usually offer a package of a few different accounts and services.
Credit account (with credit card): An account either with a store or bank, that allows the account holder to purchase items now and pay for them later. Often the account holder is able to choose between the straight payment plan, where they will pay back the money in one lump sum, or the budget plan where the debt payments are divided up over a set number of payments. The two different payments have two different interest rates.

The type of account you should open depends on your needs. For example, you might not have a fixed income, but want to save money to buy a Playstation, so a savings account would be the most appropriate for your needs as a learner.
Someone who is employed full-time will need a cheque account, so that their salary can be deposited there and they can apply for other benefits attached to this type of account.

According to FICA (the Financial Intelligence Centre Act), people need to supply certain information and documents when they open a bank account.

A bank statement is usually sent to the account holder monthly. Bank statements show the following for each transaction:
So a bank statement gives you a running total of the amount of money in an account for the month.

Xola receives the following statement from her bank, detailing her transactions from 25/01/2013 to 25/02/2013. Study the statement and answer the questions that follow:
Date | Description | Amount | Amount | Balance |
25/01/2013 | Salary | \(\text{8 000,00}\) | \(\text{8 050,50}\) | |
27/01/2013 | Car insurance | \(--\text{100,00}\) | \(\text{7 950,50}\) | |
01/02/2013 | Electronic transfer Mr Serei (RENT) | \(--\text{3 000,00}\) | \(\text{4 950,50}\) | |
\(\text{01}\)/\(\text{02}\)/\(\text{2 013}\) | Debit order Healthsaver medical aid | \(--\text{500,00}\) | \(\text{4 450,50}\) | |
\(\text{02}\)/\(\text{02}\)/\(\text{2 013}\) | Debit order Mobi contract | \(--\text{250,00}\) | \(\text{4 200,50}\) | |
\(\text{03}\)/\(\text{02}\)/\(\text{2 013}\) | Debit order Supa Fashion Store | \(--\text{300,00}\) | \(\text{3 900,50}\) | |
05/02/2013 | Purchase at Shop 'n Save | \(--\text{2 000,00}\) | \(\text{1 900,50}\) | |
14/02/2013 | PAYMENT Mrs S Khumalo | \(\text{500,00}\) | \(\text{2 400,50}\) | |
20/02/2013 | Automechanix | \(--\text{1 000,00}\) | \(\text{1 400,50}\) | |
Total remaining: | \(\text{1 400,50}\) |
Xola wants to save \(\text{15}\%\) of her remaining money this month. How much can she save?

Credits: Salary, deposit from Mrs S Khumalo.
Debits: Car insurance, rent, medical aid, cellphone contract, clothing store account, groceries, car repair.
Here is an incomplete bank statement for Koketso's savings account at the end of March:
Date | Transaction | Payment | Deposit | Balance |
27/02/2013 | OPENING BAL | \(\text{2 304,85}\) | ||
1/03/2013 | INTEREST ON CREDIT BALANCE | \(\text{13,95}\) | ||
1/03/2013 | CHEQUE (SALARY) | \(\text{2 100,00}\) | ||
\(\text{1}\)/\(\text{03}\)/\(\text{2 013}\) | ATM CASH | \(\text{400,00}\) | ||
5/03/2013 | ATM CASH | \(\text{800,00}\) | ||
10/03/2013 | ATM DEPOSIT | \(\text{600,00}\) | ||
22/3/2013 | SPENDLESS DEBIT CARD PURCHASE | \(\text{235,95}\) |
How are the debits and credits indicated on this statement?
As payments (debits) and deposits (credits) respectively.
Copy Koketso's statement and complete the balance column as a running total.
Date | Transaction | Payment | Deposit | Balance |
27/02/2013 | OPENING BAL | \(\text{2 304,85}\) | ||
1/03/2013 | INTEREST ON CREDIT BALANCE | \(\text{13,95}\) | \(\text{2 318,80}\) | |
1/03/2013 | CHEQUE (SALARY) | \(\text{2 100,00}\) | \(\text{4 418,80}\) | |
1/03/2013 | ATM CASH | \(\text{400,00}\) | \(\text{4 018,80}\) | |
5/03/2013 | ATM CASH | \(\text{800,00}\) | \(\text{3 218,80}\) | |
10/03/2013 | ATM DEPOSIT | \(\text{600,00}\) | \(\text{3 818,80}\) | |
22/3/2013 | SPENDLESS DEBIT CARD PURCHASE | \(\text{235,95}\) | \(\text{3 582,85}\) |
What is Koketso's balance at the end of March?
\(\text{R}\,\text{3 582,95}\)
Koketso aims to keep a minimum balance of \(\text{R}\,\text{2 500}\) in his account to earn interest. Is he succeeding?

Yes.
Banks charge fees for the services they provide. Most cheque accounts, for example, have a monthly fee that needs to be paid to keep the account open. The bank will automatically deduct this fee out from the account every month. As well as this monthly fee, some banks charge a fee every time you withdraw or deposit money and for other services. These bank charges are called transaction fees. The transaction fees vary depending on which bank you use and which type of account you have.
You can reduce your transaction fees by choosing the most appropriate bank account for your own needs and also by adjusting your banking habits. For example, a bank account may offer a certain number of free withdrawals, deposits and balance enquiries per month, so if you keep within those, you can keep the fees low.

Arthur's bank, Egoli Bank list the following banking fees :
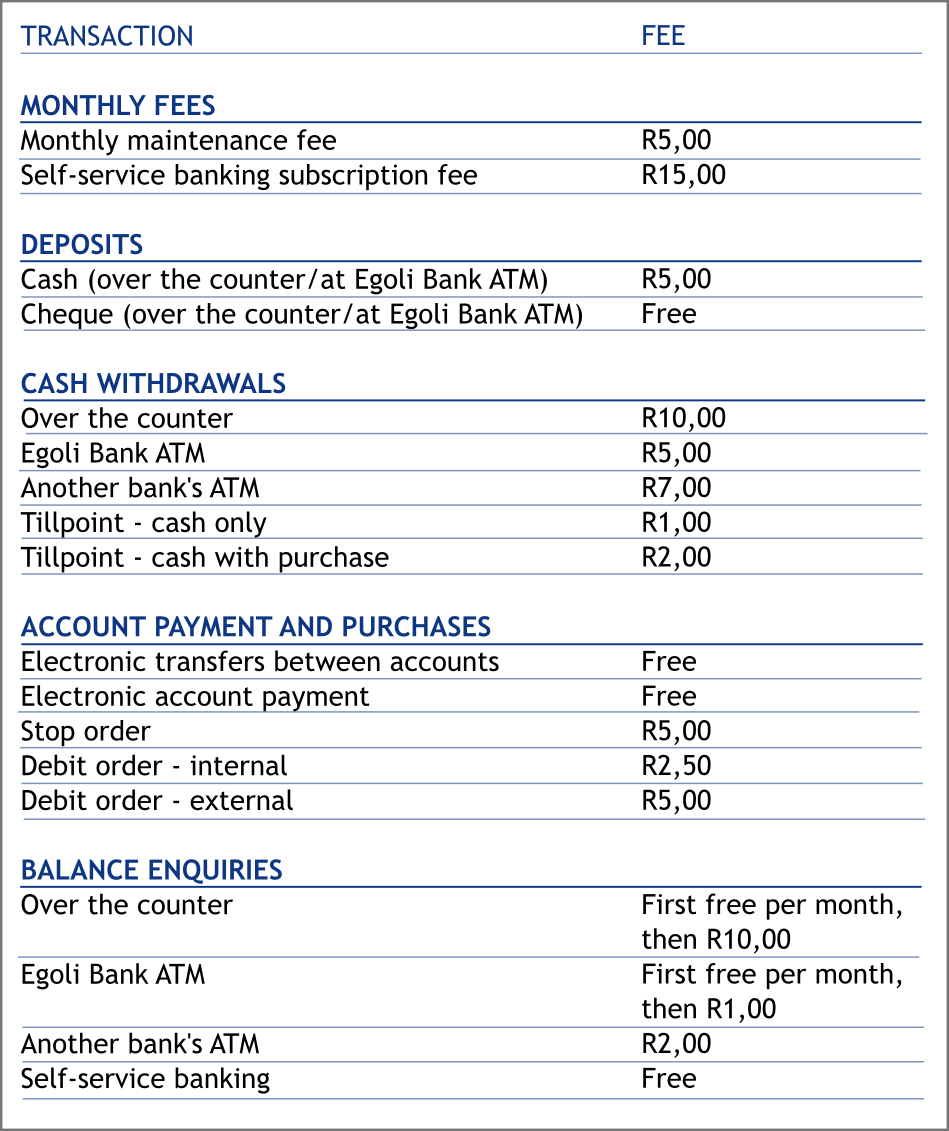
Arthur subscribes to self-service banking and pays a monthly maintenance fee. In the space of a month Arthur performs the following transactions:
Arthur has a balance of \(\text{R}\,\text{650}\) in his bank account at the end of the month.
Lulama wants to withdraw \(\text{R}\,\text{650}\) from her savings account, at the ATM. The transaction fee for withdrawals is \(\text{R}\,\text{2,25}\) for the first \(\text{R}\,\text{100}\) plus \(\text{R}\,\text{1,20}\) for every additional \(\text{R}\,\text{100}\) (or part thereof). Calculate the bank fee.

\(\text{R}\,\text{650}\) (divided into full \(\text{R}\,\text{100}\) amounts or part thereof) | ||||||
|
|
|
|
|
|
|
\(\text{R}\,\text{100}\) | \(\text{R}\,\text{100}\) | \(\text{R}\,\text{100}\) | \(\text{R}\,\text{100}\) | \(\text{R}\,\text{100}\) | \(\text{R}\,\text{100}\) | \(\text{R}\,\text{50}\) |
(tariff/fee per \(\text{R}\,\text{100}\) or part thereof) | ||||||
|
|
|
|
|
|
|
\(\text{R}\,\text{2,25}\) | \(\text{R}\,\text{1,20}\) | \(\text{R}\,\text{1,20}\) | \(\text{R}\,\text{1,20}\) | \(\text{R}\,\text{1,20}\) | \(\text{R}\,\text{1,20}\) | \(\text{R}\,\text{1,20}\) |
Therefore: \(\text{R}\,\text{2,25}\) for the first \(\text{R}\,\text{100}\) plus \(\text{R}\,\text{1,20}\) for every additional \(\text{R}\,\text{100}\) (or part thereof).
Bank fee = \(\text{R}\,\text{2,25}\) + ( \(\text{R}\,\text{1,20}\) \(\times\) \(\text{6}\)) = \(\text{R}\,\text{9,45}\)
Mia has recently open a Global account at Capital Bank. She is concerned about her monthly bank charges. Use the provided brochure and the list of her account activities for the month of April to answer the questions below:
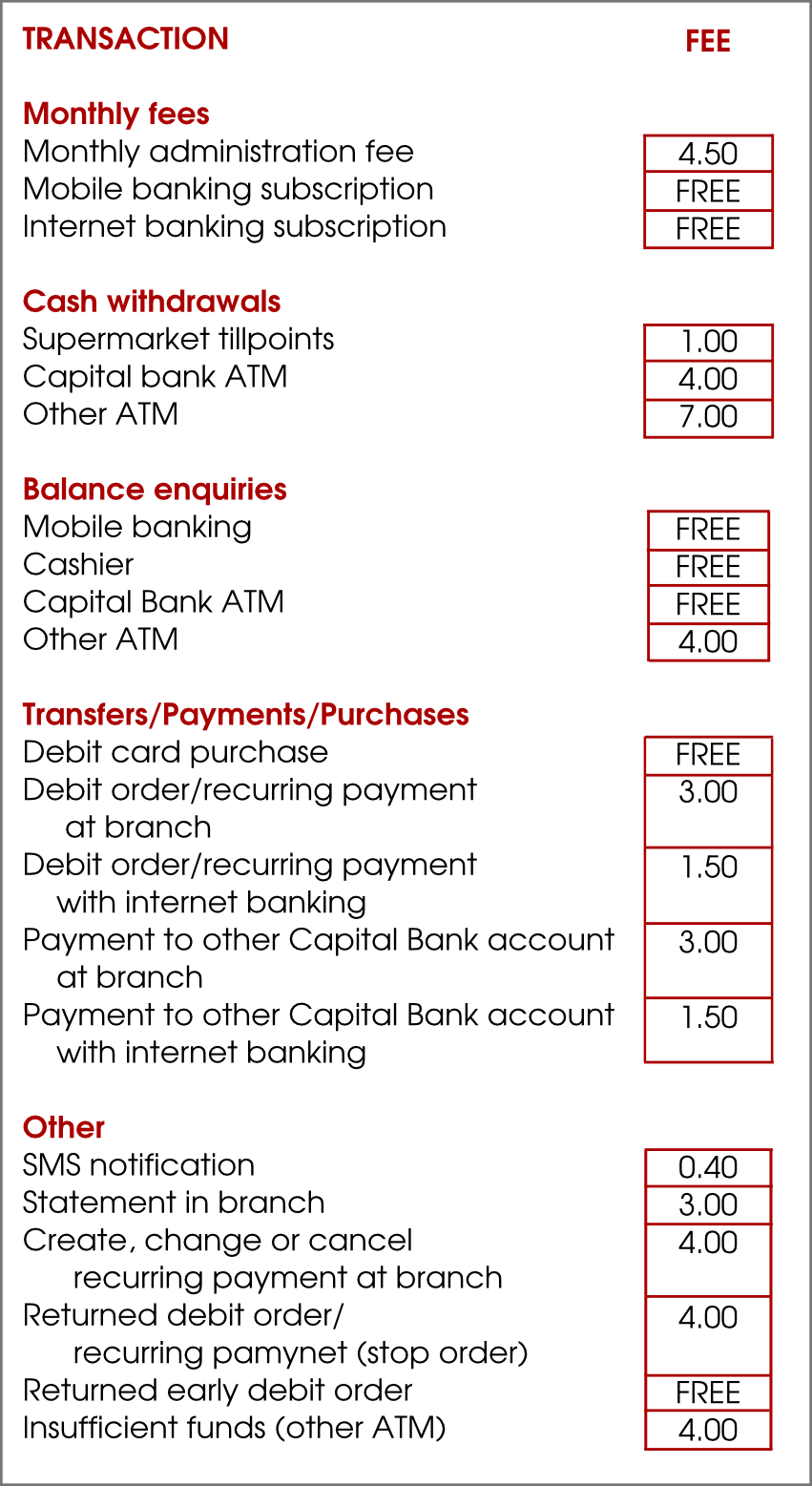
The list of Mia's transactions for April is as follows:
Date | Activities | Amounts |
1 Apr 2013 | Balance of previous month carried forward | \(\text{R}\,\text{210,25}\) |
1 Apr 2013 | Old Mutual Policy x74534: Debit order returned: insufficient funds* | \(\text{R}\,\text{254,39}\) |
1 Apr 2013 | Balance enquiry (mobile) | \(\text{R}\,\text{0,00}\) |
2 Apr 2013 | Davidsons Textiles: Salary deposit* | \(\text{R}\,\text{4 500,00}\) |
2 Apr 2013 | Shoprite: Purchases: Debit card* | \(\text{R}\,\text{847,21}\) |
2 Apr 2013 | Shoprite: Cash withdrawal*. | \(\text{R}\,\text{250,00}\) |
7 Apr 2013 | Old Mutual Policy x74534: Branch Payment | \(\text{R}\,\text{254,39}\) |
15 Apr 2013 | Edgars: Purchases: Debit card* | \(\text{R}\,\text{149,59}\) |
20 Apr 2013 | Capital Bank ATM Withdrawal* | \(\text{R}\,\text{200,00}\) |
23 Apr 2013 | Shoprite: municipal account payment* | \(\text{R}\,\text{639,00}\) |
28 Apr 2013 | FNB ATM Withdrawal* | \(\text{R}\,\text{500,00}\) |
29 Apr 2013 | Balance statement at the branch | \(\text{R}\,\text{3,00}\) |
30 Apr 2013 | Monthly Administration Fee | \(\text{R}\,\text{4,50}\) |
* denotes SMS notification for April
How many withdrawals did Mia make during this month?
Three.
Calculate the amount of money that was spent on monthly shop purchases
\(\text{R}\,\text{847,21}\) + \(\text{R}\,\text{149,59}\) = \(\text{R}\,\text{995,80}\)
Use the relevant resources and calculate the amount of bank fees that Mia should pay for April.
Returned debit order: \(\text{R}\,\text{4,00}\). Cash withdrawal at Shoprite: \(\text{R}\,\text{1,00}\). Old Mutual debit order payment at branch: \(\text{R}\,\text{3,00}\). Capital Bank ATM withdrawal: \(\text{R}\,\text{4,00}\). FNB ATM withdrawal: \(\text{R}\,\text{7,00}\). Balance query in branch: \(\text{R}\,\text{3,00}\). Monthly admin fee: \(\text{R}\,\text{4,50}\). \(\text{8}\) SMS notifications: \(\text{R}\,\text{3,20}\). Total: \(\text{R}\,\text{29,70}\)
Suggest how Mia can further reduce her banking charges.
She could do away with SMS notifications, only draw cash at tillpoints, make sure her debit orders don't get returned and so on.
A bank uses the following formula to calculate the bank charges (transaction fee) on money deposited at a branch (inside the bank): Transaction fee = \(\text{R}\,\text{2,50}\) + \(\text{0,95}\%\) of the amount deposited.
Use the above formula to calculate the bank fees on the following deposits:
\(\text{R}\,\text{450}\)
\(\text{R}\,\text{2,50}\) + \(\text{R}\,\text{4,275}\) = \(\text{R}\,\text{6,78}\)
\(\text{R}\,\text{117,35}\)
\(\text{R}\,\text{2,50}\) + \(\text{R}\,\text{1,11}\) = \(\text{R}\,\text{3,61}\)
\(\text{R}\,\text{6 500 000}\)
\(\text{R}\,\text{2,50}\) + \(\text{R}\,\text{61 750}\) = \(\text{R}\,\text{61 752,50}\)
Use this formula to see if the transaction fee of \(\text{R}\,\text{5,59}\) was correctly calculated from a deposit of \(\text{R}\,\text{325}\).
\(\text{R}\,\text{2,50}\) + \(\text{R}\,\text{3,0875}\) = \(\text{R}\,\text{5,59}\). Yes, it was calculated correctly.
Calculate the bank fee on the following deposits at the ATM if the transaction fee is \(\text{R}\,\text{0,90}\) for every \(\text{R}\,\text{100}\) (or part thereof) deposited:
\(\text{R}\,\text{450}\)
\(\text{R}\,\text{0,90}\) \(\times\) \(\text{5}\) parts of \(\text{R}\,\text{100}\) = \(\text{R}\,\text{4,50}\)
\(\text{R}\,\text{637,14}\)
\(\text{R}\,\text{0,90}\) \(\times\) \(\text{7}\) parts of \(\text{R}\,\text{100}\) = \(\text{R}\,\text{6,30}\)
\(\text{R}\,\text{3 500,05}\)
\(\text{R}\,\text{0,90}\) \(\times\) \(\text{36}\) parts of \(\text{R}\,\text{100}\) = \(\text{R}\,\text{32,40}\)
Tumi withdrew \(\text{R}\,\text{350}\) from her savings account, at the ATM. The withdrawal transaction fee is \(\text{R}\,\text{2,35}\) for the first \(\text{R}\,\text{100}\), plus \(\text{R}\,\text{1,15}\) for every additional \(\text{R}\,\text{100}\) (or part thereof). Calculate the bank fee.
\(\text{R}\,\text{2,35}\) + \(\text{3}\) \(\times\) \(\text{R}\,\text{1,15}\) = \(\text{R}\,\text{5,80}\)
The transaction fee of a cash deposit at a branch is \(\text{R}\,\text{2,45}\) + \(\text{0,85}\%\) of the deposited amount. If Tumi deposits \(\text{R}\,\text{875,00}\) at the bank, calculate the bank charges.
\(\text{R}\,\text{2,45}\) + (\(\text{0,85}\%\) of \(\text{R}\,\text{875}\)) = \(\text{R}\,\text{2,45}\) + \(\text{R}\,\text{7,44}\) = \(\text{R}\,\text{9,99}\)
Demi wants to withdraw \(\text{R}\,\text{750,00}\) from her savings account at an ATM. The withdrawal transaction fee is \(\text{R}\,\text{1,20}\) for the first \(\text{R}\,\text{100}\) plus \(\text{R}\,\text{0,75}\) for every additional \(\text{R}\,\text{100}\) (or part thereof). Calculate the bank fee.

\(\text{R}\,\text{1,20}\) + (\(\text{6}\) \(\times\) \(\text{R}\,\text{0,75}\)) = \(\text{R}\,\text{5,70}\)
A bank charges the following fees for cash deposits:
Bank teller: \(\text{2,5}\%\) of deposit value
ATM deposit: \(\text{R}\,\text{1}\) basic + \(\text{R}\,\text{1,20}\) per \(\text{R}\,\text{100}\) or part of \(\text{R}\,\text{100}\)
Is it more expensive to go into the bank or to do the transaction at an ATM? Why do you think this is?
It is cheaper to use the ATM. If you deposited \(\text{R}\,\text{200}\), for example, the bank fees would be \(\text{R}\,\text{1}\) + \(\text{R}\,\text{2,40}\) = \(\text{R}\,\text{3,40}\). The same deposit at a teller would cost \(\text{R}\,\text{5,00}\). It is cheaper to deposit the cash at an ATM, because the process is automated and does not involve a skilled employee.
Using the formulae given above, fill in the table below to show the transaction fees for the given amounts of money:
Deposit amount (R) | \(\text{500}\) | \(\text{1 000}\) | \(\text{1 500}\) | \(\text{2 000}\) | \(\text{2 500}\) | \(\text{3 000}\) | \(\text{3 500}\) |
Fee at teller | |||||||
Fee at ATM |
Deposit amount (R) | \(\text{500}\) | \(\text{1 000}\) | \(\text{1 500}\) | \(\text{2 000}\) | \(\text{2 500}\) | \(\text{3 000}\) | \(\text{3 500}\) |
Fee at teller | \(\text{R}\,\text{12,50}\) | \(\text{R}\,\text{25,00}\) | \(\text{R}\,\text{37,50}\) | \(\text{R}\,\text{50,00}\) | \(\text{R}\,\text{62,50}\) | \(\text{R}\,\text{75,00}\) | \(\text{R}\,\text{87,50}\) |
Fee at ATM | \(\text{R}\,\text{7,00}\) | \(\text{R}\,\text{13,00}\) | \(\text{R}\,\text{19,00}\) | \(\text{R}\,\text{25,00}\) | \(\text{R}\,\text{31,00}\) | \(\text{R}\,\text{37,00}\) | \(\text{R}\,\text{45,00}\) |
Plot two graphs of the transaction fees for the different amounts of money given in the table.
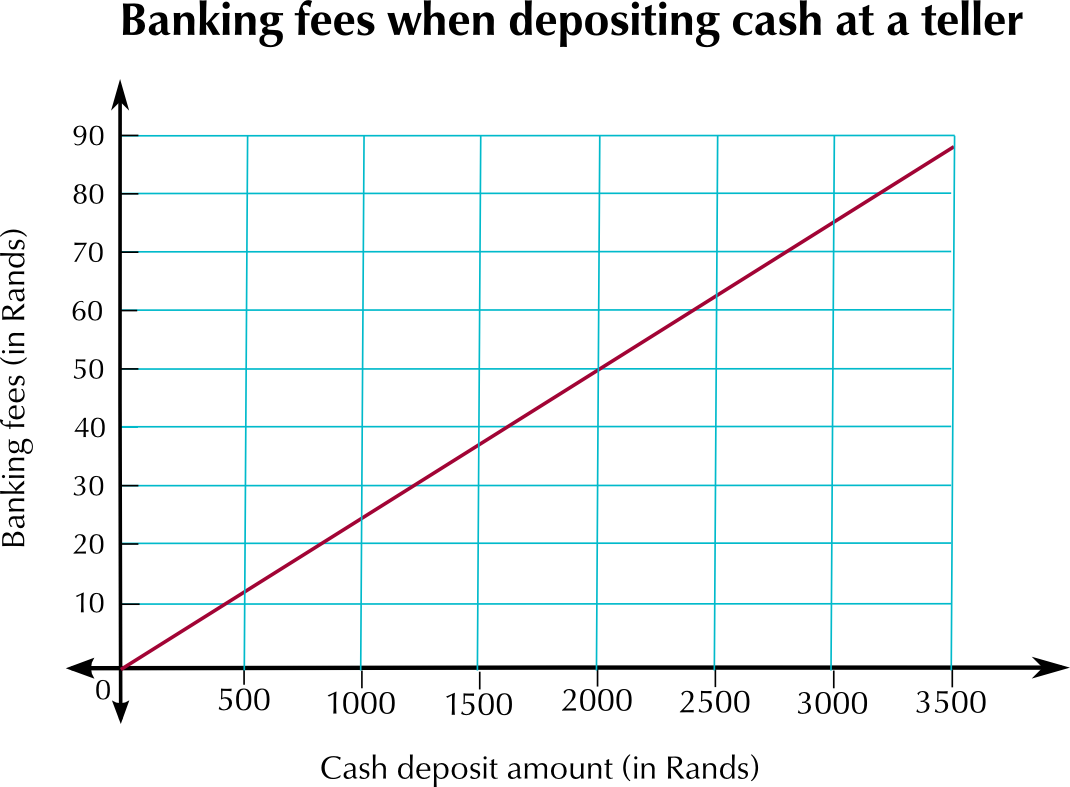
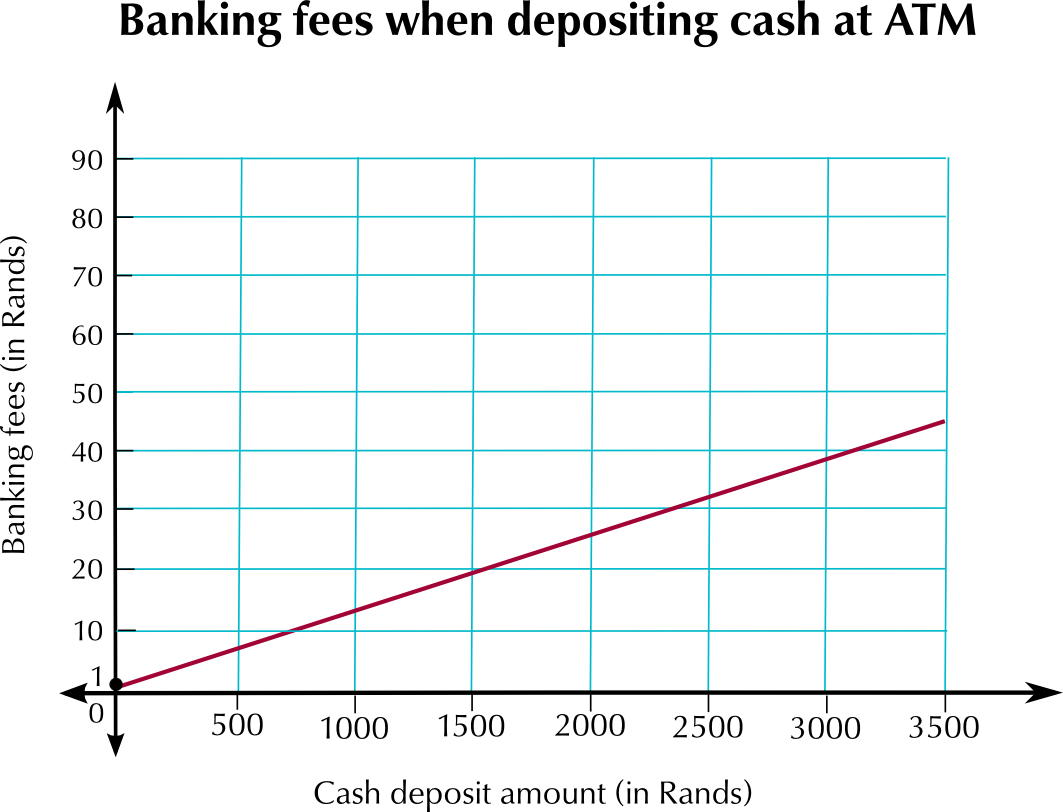
Read off the graph: how much money can you save by using an ATM if you need to deposit \(\text{R}\,\text{1 250}\)?
Approximately \(\text{R}\,\text{15}\)
|
Previous
11.1 Introduction and key concepts
|
Table of Contents |
Next
11.3 Interest
|